ETF2700/ETF5970 Mathematics for Business Assignment 2
Semester 2, 2018
Submission
This assignment comprises 10% of the assessment for ETF2700/ETF5970. You must submit a “hard copy” of your written work (with an Assignment Cover Sheet – from the “ASSIGNMENTS” section of Moodle) by 6pm on Monday 17 September 2018. Submit it to your tutor in your tutorial (or to your tutor’s mailbox, 5th floor H Block).
ENSURE that you
• Submit a printed “hard copy” of your assignment to your tutor. Your assignment must be typed.
• 
Name your assignment: Surname-Initials A2.docx, e.g., Einstein-A A2.docx.
• Upload this file to Moodle as follows:
Go to the “ASSIGNMENTS” section. Click on the “Submit your Assignment 2 here – Due on 17 Sep” link to upload. The following message will appear momentarily, “File uploaded successfully.”
[To later confirm your upload was successful, go to the “ASSIGNMENTS” section and click on the Assignment 2 uploading link. The uploaded files name will be shown.]
NB, DO NOT submit any Excel files. You may upload only ONE file.
• Submit both hard copy and electronic copy before the due, otherwise your submission will NOT be accepted.
• Retain your marked assignment until after the publication of final results for this unit.
Further Information
• A maximum penalty of 10% of the total mark allocated to this assessment will be deducted for each day that it is late, up to 4 days. An assignment may not be submitted if it is late by more than 4 days.
• Extensions beyond the due date will only be allowed in special circumstances. You may visit https://www.monash.edu/exams/changes/special-consideration
for the university policy and application procedure for special consideration.
• Do not submit your assignment in a folder: stapled pages are easier for the marker.
• Save trees! Double-sided printing is encouraged.
• If you don’t understand what the questions are asking,
– study the unit’s content prior to attempting the tutorial and assignment questions. This should enhance your ability to understand the questions.
– ask a staff member to clarify the questions for you. A staff consultation roster is on Moodle.
Avoid Plagiarism!
Intentional plagiarism amounts to cheating. See the Monash Policy.
Plagiarism: Plagiarism means to take and use another person’s ideas and or manner of ex- pressing them and to pass these off as one’s own by failing to give appropriate acknowledgement. This includes material from any source, staff, students or the internet-published and unpublished works.
Collusion: Collusion is unauthorised collaboration with another person or persons. Where there are reasonable grounds for believing that intentional plagiarism or collusion has occurred, this will be reported to the Chief Examiner, who may disallow the work concerned by prohibiting assessment or refer the matter to the Faculty Manager.
Questions
Important: There are six questions. Please attempt all the questions, show all the steps of your calculations, and provide explanations to justify your answers. To obtain full marks, it is important to provide complete answers supported by logically sound explanations, unless the question explicitly states that no explanation is needed. It is not sufficient to simply provide calculator instructions.
Total marks: 100
[This assignment is worth 10% towards the final mark of this unit].
Question 1 (20 marks)
An ice-cream lover has a total of $10 to spend one evening. The price of ice-cream is $p per litre. The person’s preferences for buying q litres of ice-cream, leaving a nonnegative amount $(10 − pq) to spend on other items, are represented by the utility function: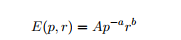
(a) Find the first-order condition for a utility maximizing quantity of ice-cream. [Hint: the square root function is a power function with exponent 1 .]
(b) Solve the first-order condition derived in (a) in order to express the utility maximizing quan- tity q∗ as a function of p.
(c) What guarantees that your quantity q∗ is really a maximum?
(d) Express the elasticity of demand for ice-cream as a function of the price $p per litre. When the price is $2.50 per litre, calculate the price elasticity of the person’s demand for ice-cream.
Question 2 (20 marks)
Suppose that a representative Norwegian family’s demand for milk depends on the price p > 0
and the family’s income r > 0 according to the function:
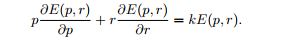
where A, a and b are positive constants.
(a) Find a constant k (in terms of the constants a and b) such that
(b) Based on data for the period 1925–1935, it is estimated that Norwegian milk demand could be represented by equation (1) with a = 1.5 and b = 2.08. Calculate the value of k in this case.
(c) For the values of a and b in part (b), suppose moreover that p = p(t) = p0(1.06)t and r = r(t) = r0(1.08)t in (1) are both functions of time t where p0 is the price and r0 is the income at time t = 0. Hence, E(p(t), r(t)) = E(t) is also a function of time t. Calculate the proportional rate of growth, that is, the derivative of function ln (E(t)).
Question 3 (20 marks)
The profit obtained by a firm from producing and selling x and y units of two brands of a com- modity is given by
P (x, y) = −0.1x2 − 0.2xy − 0.2y2 + 47x + 48y − 600.
(a) Assume P (x, y) has a maximum point. Find, step by step, the production levels that maximize profit by solving the first-order conditions. If you need to solve any system of linear equations, use Cramer’s rule and provide all calculation details.
(b) Due to technology constraints, the total production must be restricted to be 200 units. Find, step by step, the production levels that now maximize profits – using the Lagrange Method. If you need to solve any system of linear equations, use Cramer’s rule and provide all calculation details. You may assume that the optimal point exists in this case.
(c) Report the Lagrange multiplier value at the maximum point and the maximal profit value from question (b). No explanation is needed.
(d) Using new technology, the total production can now be up to 250 units. Use the values from question (c) to approximate the new maximal profit.
(e) Compare the true new maximal profit for question (d) with its approximate value you ob- tained. By what percentage is the true maximal profit larger than the approximate value from question (c)?
Question 4 (10 marks)
The following table contains the return on equity (in percentage) of four firms and the salary (in thousand of dollars) of their CEOs.
|
Firm No. |
Return on Equity (%) |
CEO Salary ($k) |
|
1 |
13.8 |
1368 |
|
2 |
20.0 |
1145 |
|
3 |
16.4 |
1078 |
|
4 |
5.9 |
578 |
Denote, for firm i (i = 1, . . . , 4), the return on equity (in percentage) as xi and the CEO salary (in thousand dollars) as yi. We have following observations:
(x1, y1) = (13.8, 1368), (x2, y2) = (20.0, 1145), (x3, y3) = (16.4, 1078), (x4, y4) = (5.9, 578).
We wish to fit a linear function
y = m^ x + ^c,
You may assume that f (m, c) has a minimum point.
(a) The first-order conditions for stationary point(s) can be rewritten as a system of linear equa- tions in form of
A m = 125735.6
c
8338
for some 2 × 2 coefficient matrix A to be worked out. Report the value of matrix A. No
explanation is needed.
(b) Multiply both sides of the equation above by A−1 to solve the first-order conditions derived in (a). Report the values of m and c rounded to 4 decimal places. No explanation is needed.
Question 5 (20 marks)
Suppose the market price P = P (Q) can be written as a function of the market demand quantity
Q ∈ (0, 100).
(a) Suppose the demand function is P (Q) = 500 − Q and the current market demand is Q0 = 40. Showing all steps of your working, evaluate the consumer surplus in this market
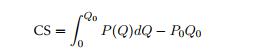
where P0 is the current market price. Round off your result to 4 decimal places.
(b) For the demand function P (Q) = 500 − 1 Q2, evaluate the consumer surplus CS = CS(Q0) as a function of Q0 ∈ (0, 100). Show that CS(Q0) is strictly increasing with Q0 by checking the sign of its derivative.
(c) Show that CS(Q0) is strictly increasing for any demand function satisfying P j(Q) < 0 for all
Q ∈ (0, 100).
Question 6 (10 marks)
Let f (x) be a function on (0, 100), having derivative f j(x) and a primitive function F (x) = ∫ x f (t)dt
defined on the same domain. For all x ∈ (0, 100), it is known that f (x) ≥ 0 and f j(x) ≤ 0.
(a) In Week 5, we learned that f j(x) ≤ 0 for all x ∈ (0, 100) implies that f (x) is decreasing. Prove this statement by using the properties of definite integrals. In other words, for all a, b ∈ (0, 100), prove that f (a) ≥ f (b) if a < b.
(b) In the same week, we also learned that F jj(x) = f j(x) ≤ 0 for all x ∈ (0, 100) implies that F (x) is concave. Prove this statement by using the properties of definite integrals and results from (a). In other words, for all a, b ∈ (0, 100), prove that
F . a + b Σ ≥ 1 (F (a) + F (b)) .
Hint: for arbitrary continuous functions h(x) and g(x) on interval [a, b],
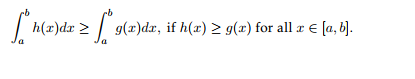
代写CS&Finance|建模|代码|系统|报告|考试
编程类：C代写,JAVA代写 ,数据库代写,WEB代写,Python代写,Matlab代写,GO语言,R代写
金融类：统计，计量，风险投资，金融工程，R语言，Python语言，Matlab，建立模型，数据分析，数据处理
服务类：Lab/Assignment/Project/Course/Qzui/Midterm/Final/Exam/Test帮助代写代考辅导
天才写手,代写CS,代写finance,代写statistics,考试助攻
E-mail:850190831@qq.com 微信：BadGeniuscs 工作时间：无休息工作日-早上8点到凌晨3点
如果您用的手机请先保存二维码到手机里面，识别图中二维码。如果用电脑，直接掏出手机果断扫描。
