MATH2089
Numerical Methods and Statistics
数值方法和统计代写 Consider an Exponential distribution, that is the probability distribution of the time between events in a Poisson point process, i.e.,
(1) TIME ALLOWED – 2 Hours
(2) TOTAL NUMBER OF QUESTIONS – 4
(3) ANSWER ALL QUESTIONS
(4) THE QUESTIONS ARE OF EQUAL VALUE
(5) THIS PAPER MAY NOT BE RETAINED BY THE CANDIDATE
(6) ONLY CALCULATORS WITH AN AFFIXED “UNSW APPROVED” STICKER
MAY BE USED
(7) STATISTICAL FORMULAE ARE ATTACHED AT END OF PAPER
Part A – Numerical Methods consists of questions 1 – 2
Part B – Statistics consists of questions 3 – 4
Both parts must be answered
All answers must be written in ink. Except where they are expressly required pencils
may only be used for drawing, sketching or graphical work.
Part A: Numerical Methods
Question 1 (20 marks) 数值方法和统计代写
Answer in a separate book marked Question 1.
Consider an Exponential distribution, that is the probability distribution of the time between events in a Poisson point process, i.e., a process in which events occur continuously and independently at a constant average rate. The probability density function of the Exponential distribution is expressed as the following
p(x) = λe-λx
Assume that the rate parameter of the distribution is λ = 1.5.
Numerically compute p(0 < x < 4).
(a) [5 marks] Apply single application trapezoidal rule.
(b) [5 marks] Apply multiple-application trapezoidal rule with ? = 4.
(c) [5 marks] Apply multiple-application trapezoidal rule with ? = 8.
(d) [5 marks] Calculate the relative error for (a), (b) and (c) given the exact solution is 0.9975. Also, show that the relative error is roughly inversely proportional to n2.
NOTE: Round to 6 decimal points when necessary.
Question 2(20 marks)
Answer in a separate book marked Question 2.
Part B – Statistics
Answer in a separate book marked Question 3 数值方法和统计代写
a) [9 marks]
Wildlife biologists inspect 153 deers taken by hunters and find 32 of them carrying ticks that test positive for Lyme disease.
i) [5 marks] Create a two-sided 96% confifidence interval for the percentage of deer that may carry such ticks.
Hint: You can use the following output from Matlab:
norminv(0.94) = 1.555, norminv(0.96) = 1.751, norminv(0.98) = 2.054.
ii) [4 marks] State two assumptions you need to make in order to determine the above confifidence interval. Explain whether each seems reasonable in this situation.
b) [21 marks]
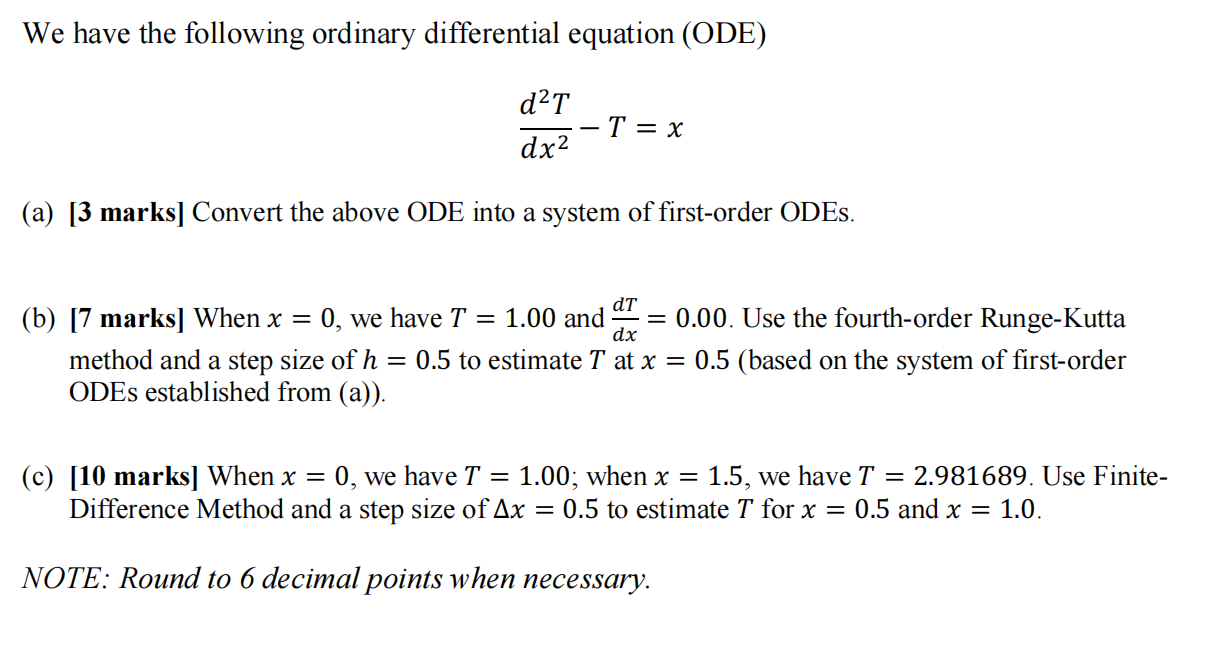
Silicon-germanium alloys have been used in certain types of solar cells. The paper “Silicon-Germanium Films Deposited by Low-Frequency Plasma-Enhanced Chemical Vapor Deposition” (J. of Material Res., 2006:88-104) reported on a study of various structural and electrical properties. Consider the accompanying data on X = Ge concentration in solid phase (ranging from 0 to 1; Ge is an abbreviation for Germanium) and Y = Fermi level position (eV):
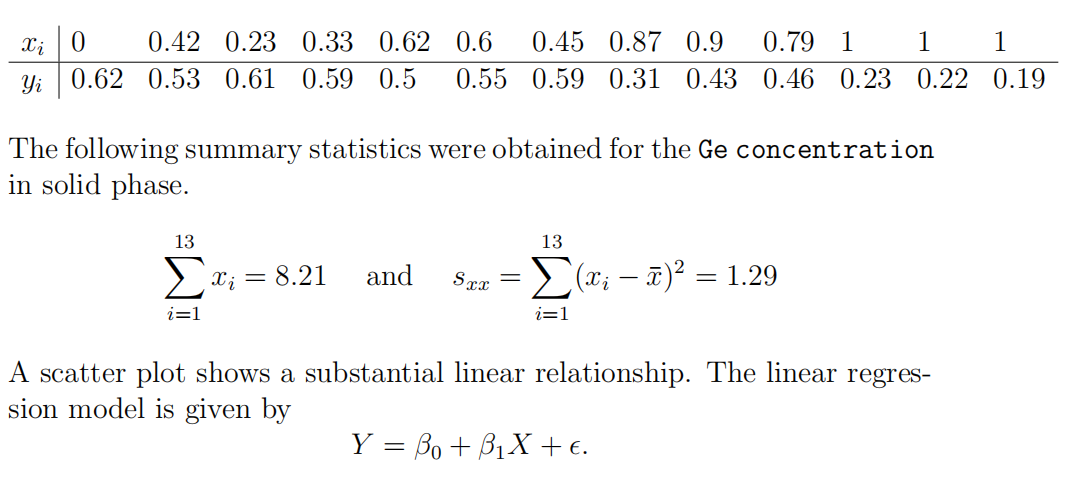
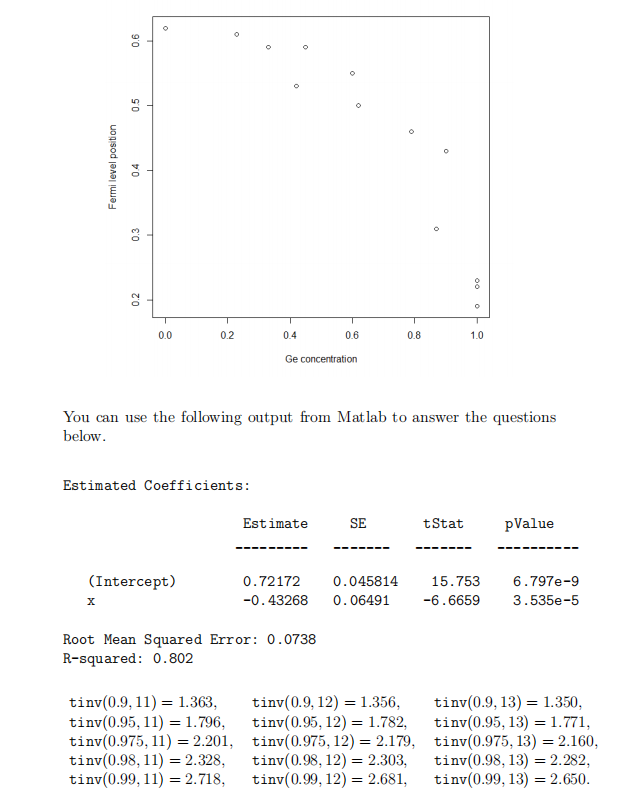
i) [1 mark] What proportion of variability in the response is explained by the predictor?
ii) [1 mark] Determine the observed sample correlation coeffiffifficient between X and Y .
iii) [7 marks] Perform a hypothesis test to determine whether the variable X is signifificant in this model, at the 5% level of signifificance. (You can use the numerical values found in the above output; however, you are required to write the details of the test: null and alternative hypotheses; rejection criterion, or observed value of the test statistics and p-value (specify the degrees of freedom if applicable); conclusion in plain language.)
iv) [3 marks] Compute a two-sided 98% confifidence interval for β1.
v) [4 marks] Compute a two-sided 90% confifidence interval for the true average Fermi level position when Ge concentration is 0.5.
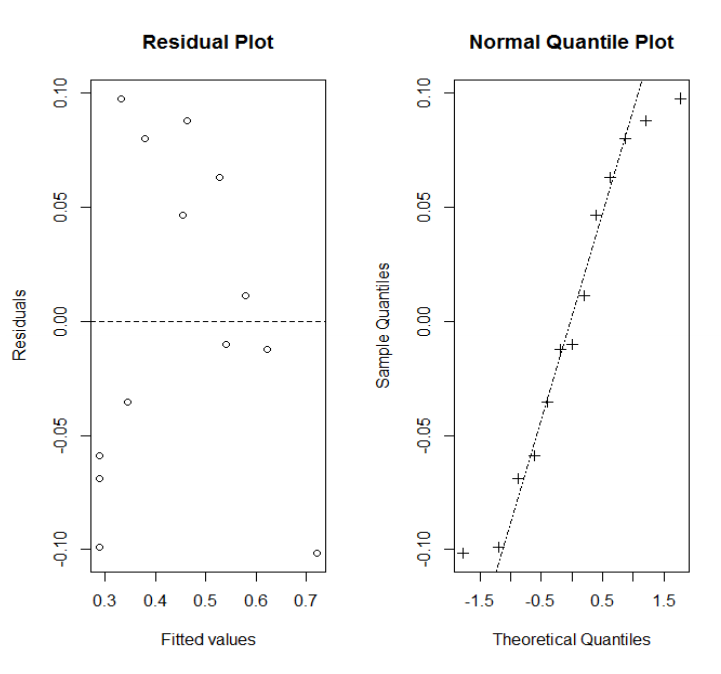
vi) [5 marks] For the above regression analysis to be valid, what are the three essential assumptions that the error in the model must satisfy? Comment on the validity of these assumptions, given the residual versus fifitted values plot and the normal quantile plot below.
Answer in a separate book marked Question 4 数值方法和统计代写
To see how much of a difffference time of day makes on the speed at which he
could download fifiles, a college sophomore performed an experiment. He placed
a fifile on a remote server and then proceeded to download it at three difffferent
time periods of the day. He downloaded the fifile 24 times in all, 8 times in each
time period. The downloading times (in seconds) are summarised in the table
below:

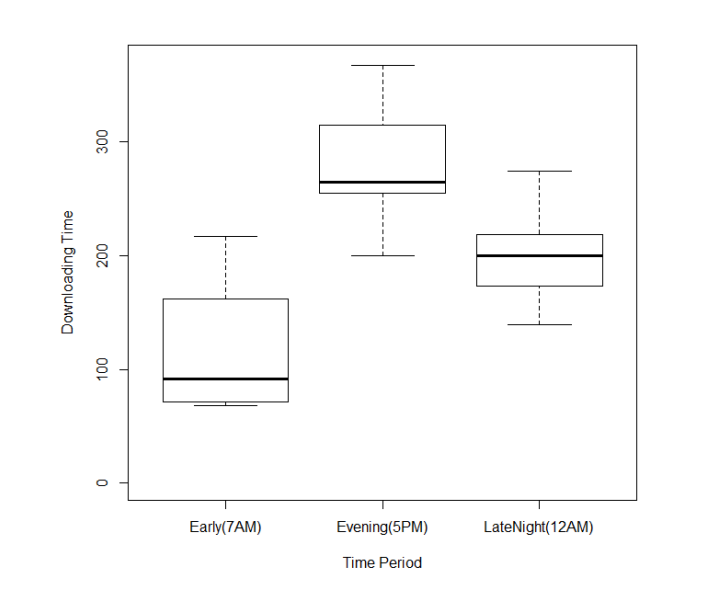
Comparative boxplots are given in the fifigure below.
You can use the following output from Matlab to answer the questions below.
tinv(0.9, 2) = 1.886, tinv(0.9, 21) = 1.323, tinv(0.9, 23) = 1.320,
tinv(0.95, 2) = 2.920, tinv(0.95, 21) = 1.721, tinv(0.95, 23) = 1.714,
tinv(0.975, 2) = 4.303, tinv(0.975, 21) = 2.080, tinv(0.975, 23) = 2.069,
tcdf(3.2729, 2) = 0.959, tcdf(3.2729, 21) = 0.9982, tcdf(3.2729, 23) = 0.9983,
finv(0.95, 2, 21) = 3.467, finv(0.95, 2, 23) = 3.422, finv(0.95, 3, 24) = 3.009,
finv(0.975, 2, 21) = 4.420, finv(0.975, 2, 23) = 4.349, finv(0.975, 3, 24) = 3.721,
fcdf(20.72, 2, 21) = 1, fcdf(20.72, 2, 23) = 1, fcdf(20.72, 3, 24) = 1.
a) [3 marks]
What do the boxplots tell you about the downloading speed at difffferent time periods of day? Comment on the shape, range and location.
b) [4 marks] List three assumptions that need to be valid for an Analysis of Variance (ANOVA) to test whether there is a difffference in average downloading time among the three time periods. Which of these three can be checked by considering the accompanying summary statistics? Explain whether these verififiable assumption(s) are supported.
Assume from now on that these assumptions are valid.
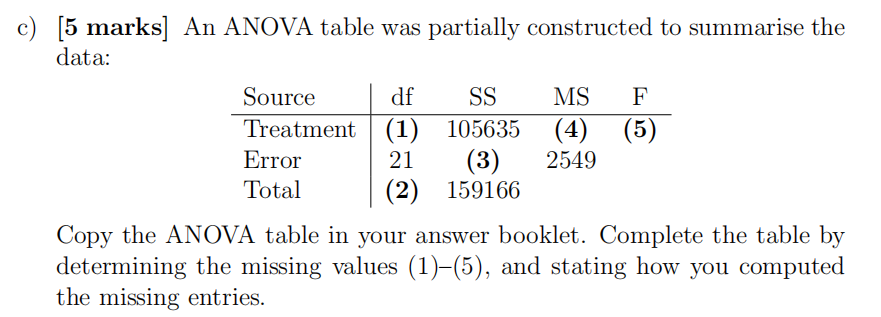
d) [7 marks] Using a signifificance level of α = 0.05, carry out the ANOVA F-test to determine whether there is a difffference in average downloading time among the three time periods.
(You can use the numerical values found in the above ANOVA table; however, you are required to write the detail of the test: null and alternative hypotheses; observed value of the test statistic; rejection criterion or the p-value (you may use bounds for the p-value, specify the degrees of freedom if applicable); conclusion in plain language.)
e) [4 marks] From the previous results, construct a 90% two-sided confifi-dence interval on the difffference between the “true” downloading times in the evening and late at night, that is, µ2 − µ3.
f) [7 marks] Using the Bonferroni adjustment, carry out a t-test comparing the “true” average downloading time in the early morning and late at night. Does this allow you to come to the same conclusion as the ANOVA F-test in d), at overall level α = 0.05? Explain.
(You can use the numerical values found in the above ANOVA table; however, you are required to write the detail of the test: null and alternative hypotheses; observed value of the test statistic and p-value (specify the degrees of freedom if applicable).)

更多代写:仿真代写 新加坡会计代考 英国靠谱论文代写机构 土木工程essay代写 文科英语assignment代写 高级财务模型代写
