Math 632 Fall 2020
Final Exam–Version 1
Name:
Math 632代写 1.Imagine a road traveled by two kinds of vehicles, trucks and cars. Three out of every five trucks are followed by acar,
- Thereare ftve problems in this exam and some have multiple The points add up to 100.
- Your solutions must be fully uploaded before 8 pm CDT (Wisconsin local time). Late submissions will not be accepted and receive 0 points. If there are technical difficulties, email your instructors as soon as possible. Be mindfulof the passage of time so that you do not miss the opportunity to scan and upload your work.
- You are allowed to use the course materials: lecture notes, materials on Canvas, Durrett’s textbook, and your own notes to solve the problems. No other materials or outside help is allowed. In particular, you cannot communicatewith anyone else or look up solutions on the internet.
- Simplify your answer if it is possible with reasonable e↵ort.
- Solutionswithout justification cannot get Cross out the writing that you do not wish to be graded on.

| Problem | Points |
| 1 | /25 |
| 2 | /15 |
| 3 | /25 |
| 4 | /30 |
| 5 | /5 |
| Total | /100 |
1. Math 632代写
Imagine a road traveled by two kinds of vehicles, trucks and cars. Three out of every five trucks are followed by acar, while one of every three cars is followed by a truck.
a)(5 pts) Construct a Markov chain model with two states, t for a truck and c for a car, where Xn represents the identity of the nth vehicle coming down the road.
b)(10 pts) Findthe limit
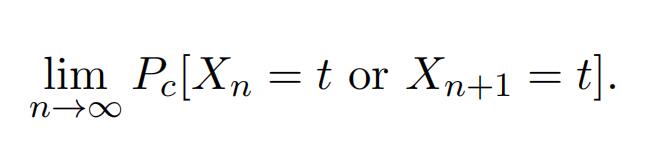
In words, this is the long-term probability that two consecutive vehicles contain at least one truck. For the sake of definiteness, we are assuming that the first vehicle we see is a car.
c)(10pts) For each integer k, let σk be the random index such that Xσk is the kth Find the limit
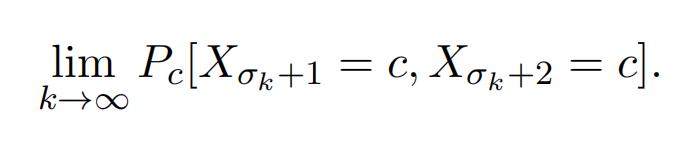
In words, this is the probability that the kth truck is followed by two cars.
2.(15 pts)
Let X1, X2, X3,. .. be i.d. random variables with possible values{—1, 0, 2} and distribution
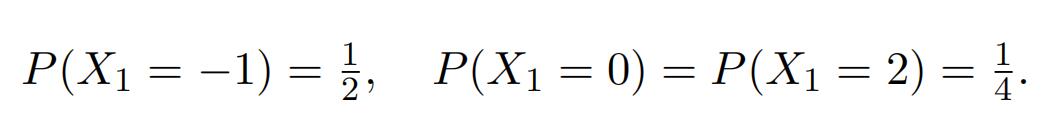
Let S0 = 0 and Sn = X1 + ·· · + Xn for n ≥ 1. Does there exist a constant A such that Mn = Sn2 — nA is a martingale with respect to {Xk : k ≥ 1}? Either justify why such a constant cannot exist, or find the value of A and justify your answer.
3.Food truck serves one customer at a time. Math 632代写
Each customer orders one of two types of meals: a simple meal with probability 2/3 and a special meal with probability 1/3, independently of the other customers. It takes 1 minute to prepare a simple meal and 4 minutes to prepare a special meal. A simple meal costs 3 dollars and a special meal 6 dollars. Customers arrive as a rate λ Poisson process (time unit is one minute). A customer who arrives when the food truck is busy leaves immediately to go buy their food elsewhere.
a)(5 pts) Let Xt= 1 if the food truck is busy at time t and Xt = 0 if there is no customer at time t. Is Xt a Markov chain? This question calls for a yes/no answer and a one-sentence justifification.
b)(10pts) What is the long term rate at which the food truck earns money?
c)(10 pts) The food truck operator wonders if the truck would earn more money over the long term if it stopped selling special meals and only provided simple meals. What is the answer? Does it depend on λ ? (To answer, assumethat the 1/3 of customers who want a special meal then go away.)
4.A delivery company has two identical cars. Math 632代写
Whenever one car breaks down, it is immediately taken to the shop for repair. If the other car breaks down before the first one is repaired, both cars are at the shop. Assume each car breaks down at exponential rate λ independently of the other, and a broken car is repaired at rate µ. If both cars are at the shop they are repaired concurrently (that is, at the same time) and independently of each other.
a)(10 pts) Let Xtbe the Markov chain that keeps track of the number of working cars (0, 1, or 2). Give the arrow diagram, routing matrix R, and generator matrix Q of this Markov chain.
b)(5 pts) Find the long-term fraction of time the company is without a working car. (Give a formula in terms of λ and µ.)
c)(5 pts) Suppose at time zero both cars are working. What is the probability that both cars function throughout the time interval [0,h]?
d)(10 pts) Suppose presently both cars are working. When the next car breaks down, what is the probability that it gets fixed before the other car breaks down? What is the probability that during the next three breakdowns,the company is never without a car?
5.(5 pts) Math 632代写
Consider the following game of chance. You start with one You flip it. If the penny comes out heads, you lose the penny and since you are now broke, the game is over. If it comes out tails, you keep the pennyand you get two more pennies. As long as you have at least one penny left, you continue in this same manner:
- Flip one of your pennies.
- Ifthe penny comes out heads, you lose that penny.
- Ifit comes out tails, you keep the penny and get two more.
The game ends if you have no pennies left. Assume that the flips of the pennies are fair coin flips.
Question: What can you say about the probability that you can go on playing forever?

其他代写:homework代写 Exercise代写 course代写 essay代写 北美代写 北美作业代写 澳大利亚代写 英国代写 app代写 algorithm代写 assignment代写 analysis代写 code代写 assembly代写 CS代写 Data Analysis代写 data代写 作业代写 作业加急 加拿大代写
