Game Theory博弈论代上网课

Final exam

Game Theory博弈论代上网课 There are two goods: a public good x and money. The unit cost of providing the public good is $1 per unit.

Question 1.  Game Theory博弈论代上网课

There are two goods: a public good x and money. The unit cost of providing the public good is $1 per unit.

Let’s consider a game where two agents have to voluntarily contribute to procure this public good that they both benefit from. The players are initially endowed only with money: Player 1 has $!₁ and Player 2 has $!₂.  Each of them chooses an amount, $z₁ and $z₂ respectively, tocontribute to procuring the public good. If player i contributes $z_i , then she is left with $!_i   z_i left over and the level of the public good is x = z₁ + z₂.

There utility of player i is

The parameter a_i is player i ’s private information and either a_i = 4 or a_i = 6. The other player believes that a_i = 4 with probability 0.5 and a_i = 6 with probability 0.5. That is, each player knows their own parameter but has a belief that the other player’s parameter is 4 or 6 with equal probability.

Find a Bayesian Nash equilibrium of this game.

Hint: A strategy specifies a player’s contribution level as a function of her parameter. Focus on finding a symmetric BNE where both players use the same strategy.

Question 2.  Game Theory博弈论代上网课

Here’s a variation on the infinite period bargaining problem we have seen. There are still only two players and they share a dollar. However, player 1 is the one who makes an offer in every period (as opposed to alternating between who makes the offer). So player 1 makes an offerin every period t = 1, 2, … of m_t [0, 1] to player 2. If player 2 accepts the offer, the game ends and if she rejects it, the game proceeds to period t + 1 and player 1 again makes an offer. The players both have the same discount factor & 2 (0, 1] so that they payoff to the offer m_t being accepted in period t is &t –1(1 – m_t ) for player 1 and &t –1m_t for player 2.

1. Define a Nash equilibrium where player 1 offers2 to player 2 in the first
2. Showthat the equilibrium you found in part 1 is not subgame perfect when & < 1?
3. Isthe equilibrium you found in part 1 subgame perfect when & = 1?
4. Finda subgame perfect equilibrium of the game when & < 

Question 3.   Game Theory博弈论代上网课

The signalling game below starts with Nature choosing the type of player 1. Player 1 then chooses either U or D. Player 2 observes the action of Player 1 but not Player 1’s type. Finally, Player 2 chooses either L or R. The payoffs are as indicated.

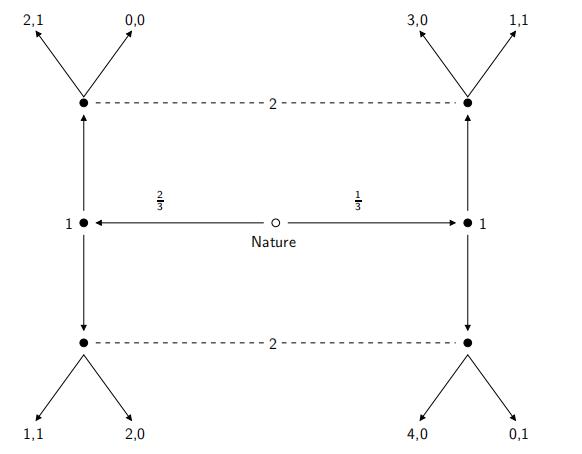
1. Is there a pure strategy separating Perfect Bayesian equilibrium? Either define the equilib- rium (remember to specify the beliefs and show that what you’ve described is a PBE) or show that one doesn’t exist.
2. Is there a pure strategy pooling Perfect Bayesian equilibrium? Either define the equilibrium(rememberto specify the beliefs and show that what you’ve described is a PBE) or show that one doesn’t  exist.

Question 4.  Game Theory博弈论代上网课

This question deals with incomplete information as a market friction. There are two traders involved in this market: a seller and a buyer. There is only one unit of the good in questionand the seller owns it. The seller knows the quality of the good, which we represent by θ. The buyer does not know θ but knows that it is uniformly distributed over [0, 1].

If the buyer buys the item and pays p for it, she enjoys a utility of

       bθ – p.

If she does not buy it, her utility is 0. For parts 1, 2, and 3 of this question, set b =

If the seller retains the item, his utility is θ and if he sells it at the price p, his utility is p.

更多代写：Bash代写 fin代考 Formal Report代写 Essay润色 澳洲英语论文代写 经济Essay代写

合作平台：essay代写 论文代写 写手招聘 英国留学生代写