Homework
Calculus作业代写 Let Σ be the plane x + 2y + 3z = 6. Consider the curve C consisting of all points on Σ that have distance 1 away from the z-axis.
Problem 1. (40 Points) Calculus作业代写
Let Σ be the plane x + 2y + 3z = 6. Consider the curve C consisting of all points on Σ that have distance 1 away from the z-axis.
(a)(5pts)What kind of shape is C? Find a parameterization of C.
(b)(5pts)Let F˙ = (ex + yz, xz y, xy). Evaluate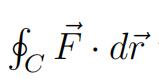
where C is oriented counter-clockwise when viewed from above.
(c)(7pts) Let C+be the part of C defined by y ≥ Suppose C+ inherits its orientation from C. Evaluate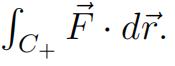 
(d)(7pts)Let R be the part of Σ enclosed by C. Find the area of R.
(e)(6pts) Let G˙ = (0, x, y) and let H˙ = curl G˙. Suppose the surface R in part (d) is oriented by upward normals. Evaluate 
(f)(4pts) Find a concave-down paraboloid S such that the intersection of S with Σ is exactly C.
(g)(6pts) Let S be the paraboloid you found in (f). Let Sjbe the part of S living above Σ and suppose S‘ is oriented by upward normals. Find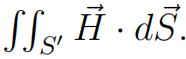 
(You don’t need the answer from (f) to do this problem.)
Problem 2. (20 Points) Calculus作业代写
(a)(4pts) Sketch the following two vectorfields:
- F˙1(x,y) = (x, y) defined on R2 − {(0, 0)}.
- F˙2(x,y) = (−x, y) defined on the upper half plane {(x, y) ∈ R2 | y > 0}.
(b)(7pts) Let a, b be constants such that a ≠ 0 and b > Find the flow-line of passing through the point (a, b). What is the shape of thisflow-line?
(c)(4pts)A nonconstant C1-function f is called an pseudo-integral of a vector field F˙ if f is constant along each flowline of F˙. Show that both F˙1 and F˙2 have pseudo-integrals.
(d)(5pts) Let g : R2 → R be a nonconstant C1-function. Define a vector field G˙ = (gy, −gx). Show that g is a pseudo-integral of G˙.
Problem 3. (40 Points)
Hansheng and his fellow mathematician Cavalieri were hanging out in a coffee shop. Theywere staring at a bagel on the table. The bagel can be described as the following geometric shape in R3: Let C0 be the circle (x− 3)2 +z2 = 1 on the xz-plane and let S be the surface obtained by revolving C0 around the z-axis. The bagel B is the solid shape enclosed by S.
(a)(8pts)“I don’t mean to brag, but I know a fast way to find the volume of the bagel,” said Cavalieri.
Find the volume of B using Cavalieri’s Principle. Calculus作业代写
(Hint: The following formula might become useful:
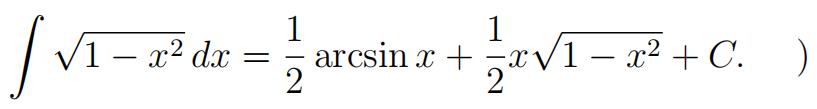
(b)(6pts)Hansheng agreed with Nonetheless, he proposed an alternative ap- proach: One can try to construct a function T : D∗ B where D∗ is a rectangular box of type [α, β] [γ, δ] [λ, η] such that T is a diffeomorphism except on a small set of measure zero. Given this, the volume of B can be calculated using the change of variable formula.
Can you construct such a function T ? (You don’t need to calculate the volume.)
“Hmm, not bad”, Cavalieri commented, “but my method was much easier.”
Out of nowhere, Hansheng remarked, “You know, if you want to calculate surface area, Cavalieri’s Principle is useless.”
Cavalieri was not thrilled.
(c)(8pts) Next day, Cavalieri announced that he established a “New Cavalieri’sPrinci- ple”. According to him, this new method calculates surface area.
He pointed to the bagel, “The surface S is just the trajectory of C0 moving around the z-axis. The center of C0 traces out a circle C : x2 + y2 = 9 on the xy-plane. Hence, it is reasonable to think of the arc length of C as the total distance traveled by C0. Therefore, the surface area of S equals
(arc length of C0) × (total distance traveled by C0) = 2π × 6π = 12π2. ”
Calculate the surface area of S and verify that Cavalieri’s answer was actually (sur- prisingly) correct. Calculus作业代写
Cavalieri continued excitedly, “The New Principle also calculates volume! For exam- ple, the volume of B equals
(the area of the unit disk enclosed by C0)×(distance traveled by C0) = π×6π = 6π2. The new method is a panacea!”
However, Hansheng pointed out that these are merely coincidences. For example, the method doesn’t help to calculate flux, as demonstrated in part (d) and (e) below.
(d)(5pts)Let’s temporarily restrict our attention to the xz-plane. Consider the 2- dimensional vector field
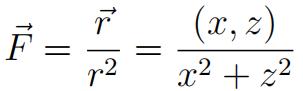
on the xz-plane. Calculate the 2-dimensional flux of F˙through the unit circle C0 : (x − 3)2 + z2 = 1.
(Recall that the “2-dimensional flux” of a vector field F˙ through a closed curve C is
∫C F˙ · ˙n ds where ˙n is the outward-pointing unit normal vector.)
(e)(5pts)Back to R3, consider the vector field
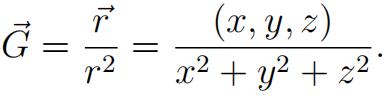
Suppose we want to calculate the flux of G˙ through S where S is oriented outward.
If the “New Cavalieri’s Principle” works, the flux ∫∫S G˙ · dS˙ should equal
(2-dimensional flux of G˙ through C0) × (distances traveled by C0)
= (your answer in part (d)) × 6π
Is this answer correct?
(f)(8pts) Consider another vector field
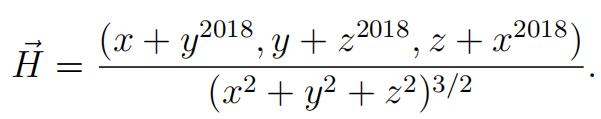
Calculate the flux of H˙through S.
Cavalieri was upset and unfriended Hansheng on Facebook.
Later, Hansheng realized that the “New Cavalieri’s Principle” may be more than just a coincidence. What do you think?
(This part does not contribute to any extra credit. Just for fun.)
更多代写：Econ网课代修价格 gmat线上考试作弊 PSY心理学代写 澳大利亚代网课 会计Midterm代考 论文summary怎么写
