ECON 701 MODULE 8 EXERCISES
经济博弈论代写 Exercise 1. Suppose that two players, Player 1 and Player 2, are involved in a sequential move game. Player 1 moves first, Player 2 moves second
Exercise 1.
Suppose that two players, Player 1 and Player 2, are involved in a sequential move game. Player 1 moves first, Player 2 moves second observing what Player 1 has done, and each player moves only once.
(i) Draw a game tree in which Player 1 has two possible actions (Up or Down) at each node and Player 2 has three possible actions (Top, Middle, or Bottom) at each node. Label the initial node of the tree “I,” each decision node “D,” and each terminal node “T.” How many of each node type are there?
(ii) Draw a game tree in which Player 1 and Player 2 each have three possible actions (Sit, Stand, or Jump) at each node. Label the nodes as in part (i). How many of each node type are there?
(iii) Draw a game tree in which Player 1 has four possible actions (North, South, East, or West) at each node and Player 2 has two possible actions (Stay or Go) at each node. Label the nodes as in part (i). How many of each node type are there?

(i) Give the associated strategic or normal form game for this extensive form game.
(ii) What are the equilibria of this game? What equilibria are subgame perfect?
Exercise 3.
Consider the following two person extensive form game.
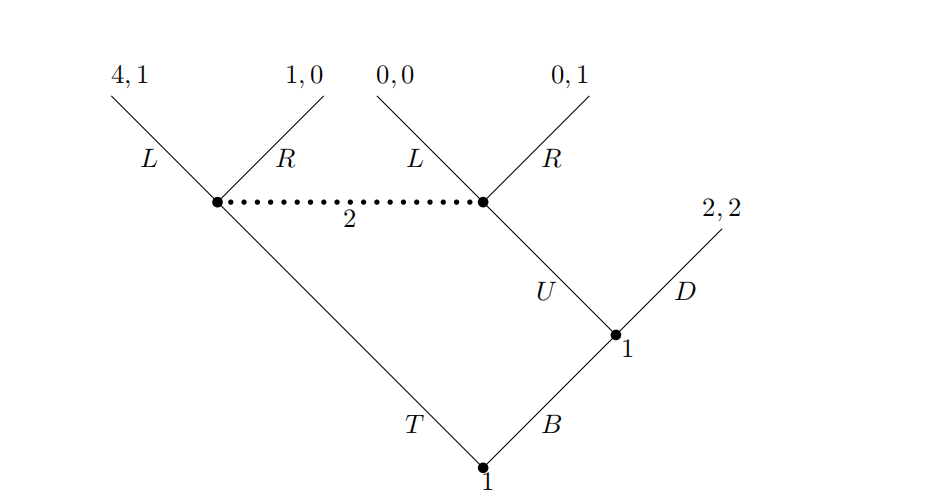
(i) How many strategies does each player have? Are any of these strategies equivalent to each other in the sense that they would give the same distribution over the terminal nodes for any particular strategy of the other player?
(ii) Give the normal form of this game. Use only one copy of the equivalent strategies.
(iii) Find all the equilibria (both in pure and mixed, or partially mixed strategies).
(iv) Identify the subgames of this game and say which equilibria are subgame perfect.
(v) Are any strategies strictly dominated for either player? (One strategy strictly dominates another if the first is strictly better that the second for any strategy of the other players.
(vi) Suppose that we delete any strictly dominated strategies strategies. Write down the game that results. Find all the equilibria of the new game. [Hint: Think, before you start.]
(vii) In this new game are there any weakly dominated strategies? If so which strategies are dominated? What happens when these strategies are deleted?
Exercise 4. 经济博弈论代写
Consider the following two person extensive form game.
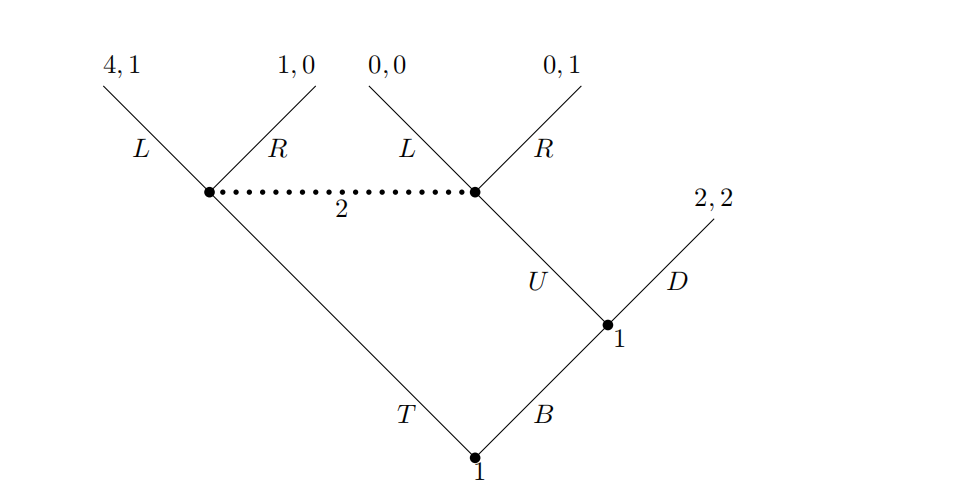
(i) How many strategies does each player have? Are any of these strategies equivalent to each other in the sense that they would give the same distribution over the terminal nodes for any particular strategy of the other player?
(ii) Give the normal form of this game. Use only one copy of the equivalent strategies.
(iii) Find all the equilibria (both in pure and mixed, or partially mixed strategies).
(iv) Identify the subgames of this game and say which equilibria are subgame perfect.
(v) Are any strategies strictly dominated for either player? (One strategy strictly dominates another if the first is strictly better that the second for any strategy of the other players.
(vi) Suppose that we delete any strictly dominated strategies strategies. Write down the game that results. Find all the equilibria of the new game. [Hint: Think, before you start.]
(vii) In this new game are there any weakly dominated strategies? If so which strategies are dominated? What happens when these strategies are deleted?
Exercise 5. 经济博弈论代写
Consider the following description of a two player extensive form game. The players are Adam and Eve. Adam moves first and chooses between X, Y , and Z. If he chooses X the game ends and both players receive $1. If he chooses Y or Z then Eve sees that he did not choose X but does not see which of Y or Z Adam chose. She then chooses between L and R. If Eve chooses L both players get nothing ($0). If Adam chose Y and Eve chose R then both players get $2.
If Adam chose Z and Eve chose R then Adam sees what has happened and chooses T or B. If Adam chooses T then both players get $5. If Adam chooses B they both have to pay $1. Assume that the players are both risk neutral so that their utility can be taken to be the amount of money they get (or the negative of the amount they have to pay).
(i) Draw, in the standard way, the extensive form of this game.
(ii) Does the game have perfect recall? Explain Why or why not.
(iii) How many pure strategies does Adam have? How many that are not equivalent to each other in the sense of Kuhn?
(iv) Give the normal form of this game. Just use one copy of the strategies that are equivalent to each other.
(v) What are the pure strategy equilibria of this game?
Exercise 6.
Consider the following two-person extensive form game.
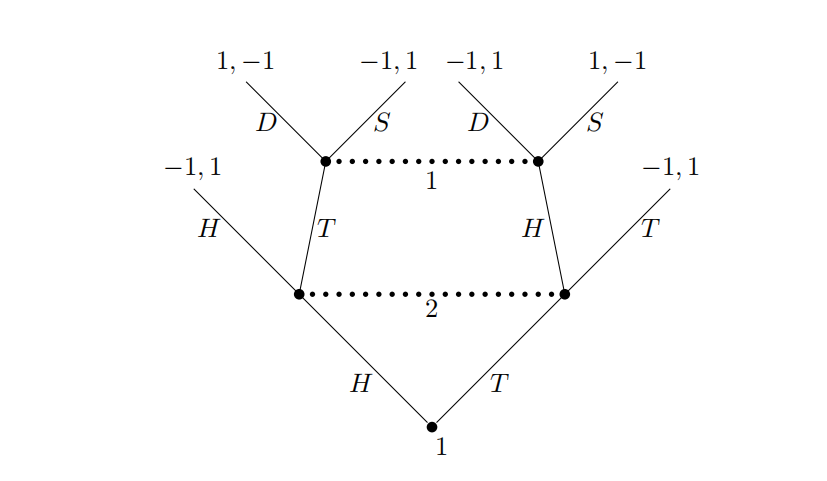
(i) Give the associated normal form of this game.
(ii) Are any of Player 1’s strategies that you found in the previous part equivalent, in the sense that they lead to the same distribution over final nodes, for any particular strategy of Player 2?
(iii) Find the Nash equilibria of this game.
(iv) What is the set of Player 1’s behaviour strategies?
Exercise 7.
Consider the following two person extensive form game. There are two players 1 and 2. Player 2 has only one information set, labelled 2. Player 1 has two information sets labelled 1a and 1b.

(i) Give a formal definition of what we mean by saying that a player in an extensive form game has perfect recall. Do the players in this game have perfect recall? Explain why or why not.
(ii) Find the associated normal form game and find all equilibria in mixed strategies. If Player 1 plays his equilibrium strategy what is the worst he can achieve for any strategy choice of Player 2?

Exercise 8. 经济博弈论代写
Consider the following two-person extensive form game. (You have looked at this game before, and, in fact already done some parts before.)
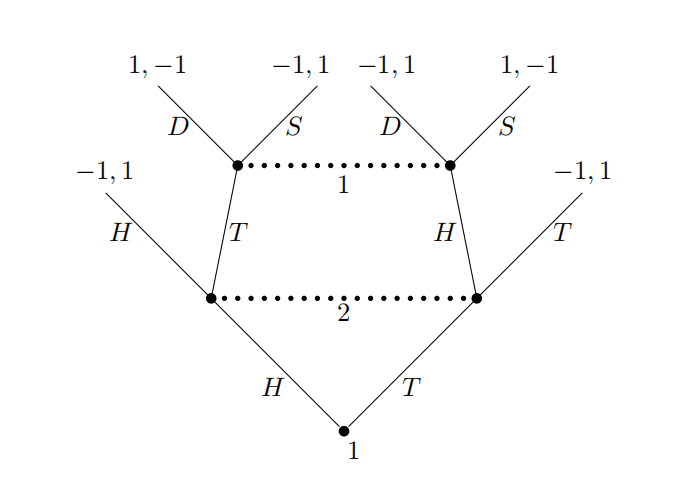
(i) Give the associated normal form of this game.
(ii) Are any of Player 1’s strategies that you found in the previous part equivalent, in the sense that they lead to the same distribution over final nodes, for any particular strategy of Player 2?
(iii) Does Player 1 have perfect recall? Does Player 2?
(iv) Find the Nash equilibria of this game.
(v) If Player 1 plays his equilibrium strategy what is the minimum that he will receive whatever Player 2 does? That is, if we fix Player 1’s mixed strategy and then tell Player 2 and let Player 2 do the worst she can against Player 1 what is Player 1’s payoff?
(vi) What is the set of Player 1’s behaviour strategies?
(vii) If we restrict Player 1 to behaviour strategies what is the most he can guarantee? That is, if we fix Player 1’s behaviour strategy and then tell Player 2 and let Player 2 do the worst she can against Player 1 what is the highest payoff that Player 1 can achieve?
Exercise 9. 经济博弈论代写
Consider the following two-person extensive form game.
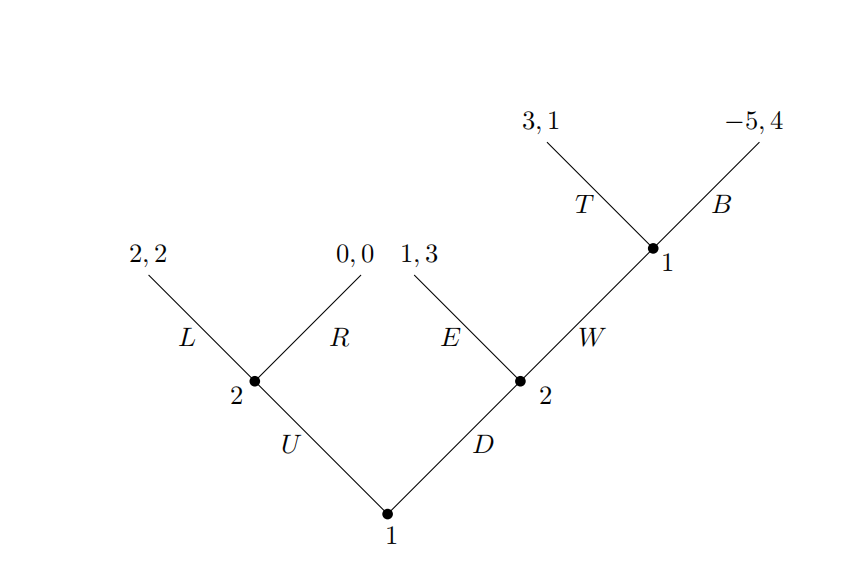
(i) List all pure strategies of each player.
(ii) Find all the proper subgames of the original game.
(iii) Carefully state what a behaviour strategy profile in this game would be and find all the subgame perfect equilibria. You might want to review the definition of a subgame perfect equilibrium before you do this.
(iv) Find the associated normal form of this game. If there are pure strategies
that are equivalent, keep only one representative pure strategy for each set of equivalent strategies. Find all the pure strategy Nash equilibria of this game. Are they all (equivalent to) subgame perfect equilibria?
Exercise 10.
Consider the following two-person extensive form game.
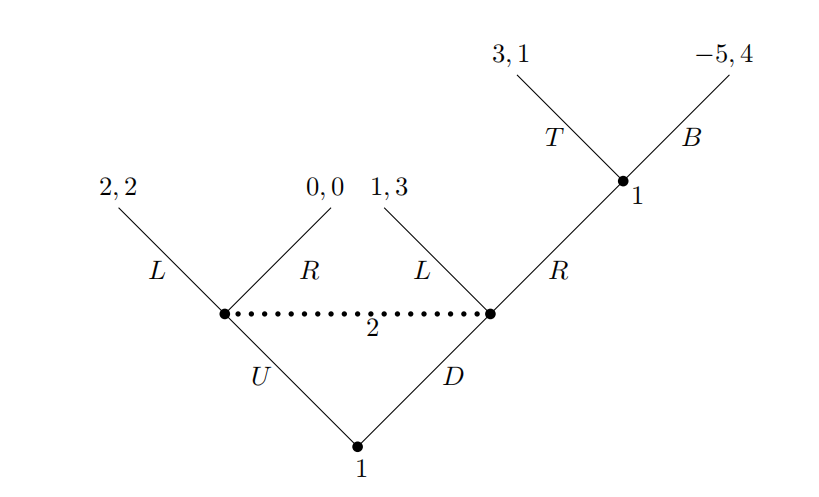
(i) List all pure strategies of each player.
(ii) Find all the proper subgames of the original game.
(iii) Carefully state what a behaviour strategy profile in this game would be and find all the subgame perfect equilibria.
(iv) Find the associated normal form of this game. If there are pure strategies that are equivalent, keep only one representative pure strategy for each set of equivalent strategies. Find all the pure strategy Nash equilibria of this game. Are they all (equivalent to) subgame perfect equilibria?
Exercise 11. 经济博弈论代写
Consider the following two-person extensive form game.
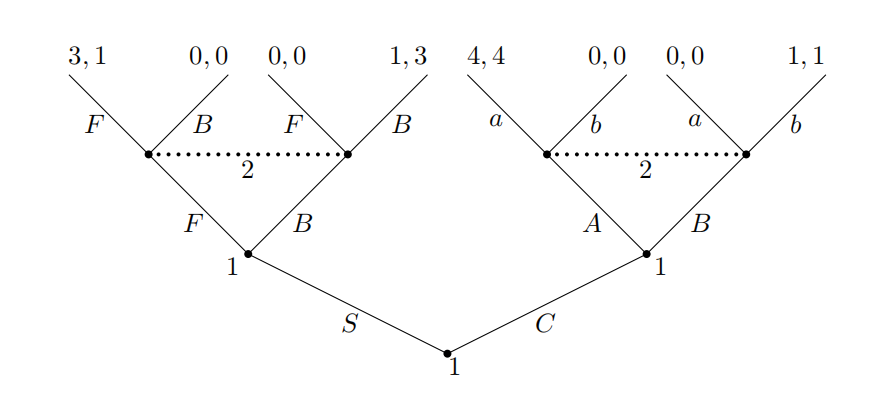
(i) List all pure strategies of each player.
(ii) Find all the proper subgames of the original game.
(iii) Carefully state what a behaviour strategy profile in this game would be and find all the subgame perfect equilibria.
(iv) Find the associated normal form of this game. If there are pure strategies that are equivalent, keep only one representative pure strategy for each set of equivalent strategies. Find all the pure strategy Nash equilibria of this game. Find one additional mixed strategy equilibrium and say whether it is equivalent to a subgame perfect equilibrium or not.
Exercise 12.
Consider the following two-person extensive form game.
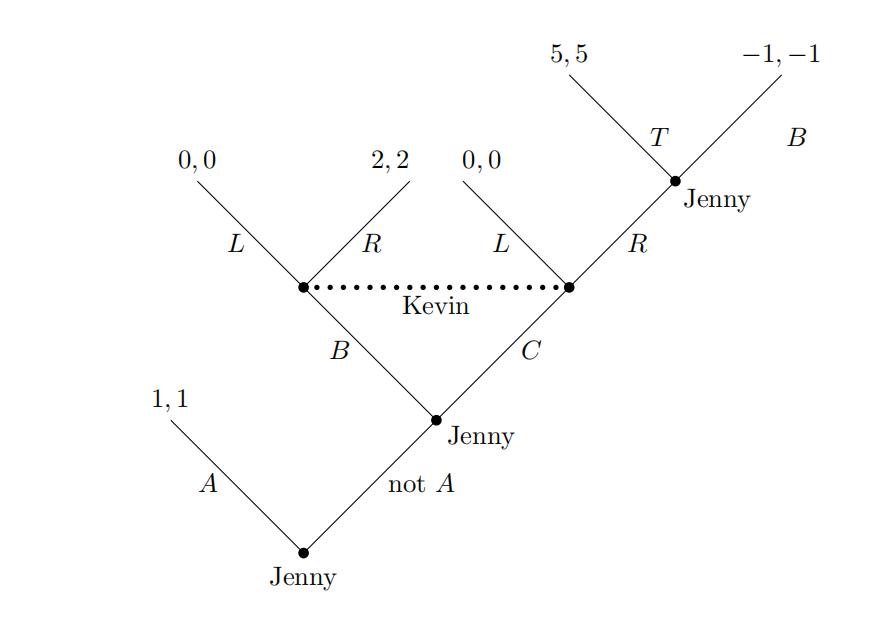
(i) Does the game have perfect recall?
(ii) Apart from the game itself how many subgames does this game have? What are they?
(iii) How many pure strategies does Jenny have? How many that are not equivalent teach other in the sense of Kuhn?
(iv) Give the normal form of this game. Just use one copy of the strategies that are equivalent to each other.
(v) What are the pure strategy equilibria of this game?
(vi) Which of the pure strategy equilibria are (equivalent to behaviour strategies that are) subgame perfect equilibria?
Exercise 13. 经济博弈论代写
Now consider the following similar game.
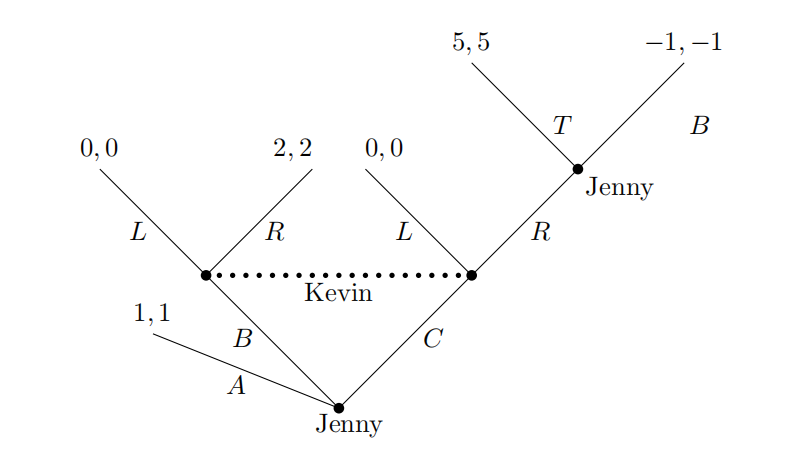
(i) Again, give the normal form of this game.
(ii) What are the subgames of this game?
(iii) What are the pure strategy equilibria of this game?
(iv) Which of the pure strategy equilibria are (equivalent to behaviour strategies that are) subgame perfect equilibria?
(v) What does this suggest about the validity of the concept of subgame perfect equilibrium as a solution concept for extensive form games?
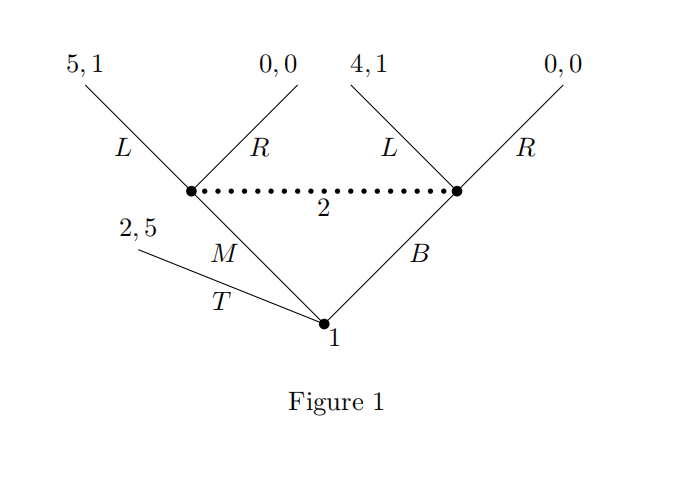
Exercise 14. 经济博弈论代写
In the game of Figure 1 if we split Player 1’s choice into two binary choices, having him first choose whether to play T and if he chose not to play T then to choose whether to play M or B, we might think that we have not very much changed the game. However in this case subgame perfection will rule out the equilibria in which Player 1 chooses T. This problem asks you to work out some of the details of this example.
(i)
Give the associated normal form of the game of Figure 1 and find all the Nash equilibria.
(ii)
What are the subgames of the game of Figure 1. Find all the subgame perfect equilibria.
(iii)
Suppose that we split Player 1’s choice into two binary choices, having him first choose whether to play T and if he chose not to play T then to choose whether to play M or B. Draw the extensive form of this game, give the associated normal form, and fifind all the Nash equilibria of the new game.
(iv)
Find the subgame perfect equilibria of the new game. Remember that sub-game perfect equilibria are defined in terms of behaviour strategies and in the new game Player 1 moves twice, so his mixed strategies and behaviour strategies are different.
(v)
Suppose that we change the game again so that Nature moves first and that with probability one half the payoffs are as the were in the original game and with probability one half the payoffs 5, 1 and 4, 1 are reversed with 5, 1 being the payoff if Player 1 chooses B and Player 2 chooses L and 4, 1 being the payoff if Player 1 chooses M and Player 2 chooses L.
Suppose that Player 1 observes Nature’s choice before making any moves and Player 2 does not observe Nature’s choice. Draw the extensive form games for these cases, both when Player 1 chooses between T, M, and B at a single node and when we split Player 1’s choice into two binary choices, having him first choose whether to play T and if he chose not to play T then to choose whether to play M or B. Find the associated normal forms and the Nash equilibria. In each case find the subgames and all the subgame perfect equilibria.
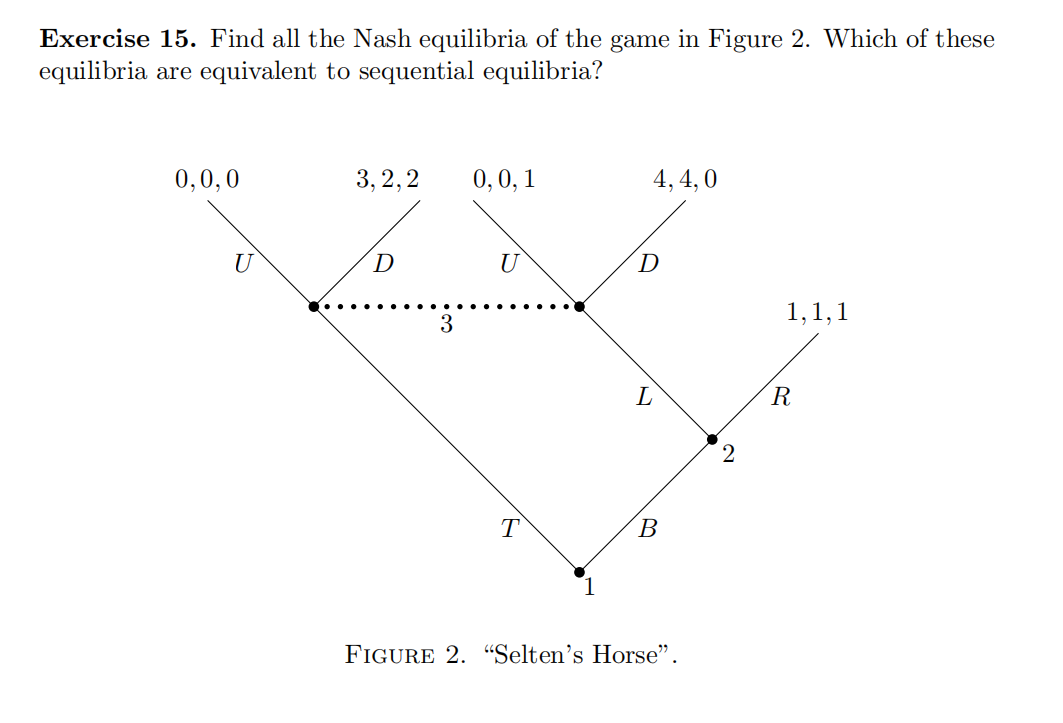

更多代写:cs quiz考试价格 gmat替考 英国math网课代考 essay写作代写 英文连接词的用法 宏观经济理论与政策代考
