MT5823 Semigroups
数学半群代考 1. (a) Show that S has 6 elements. [4] (b) Draw the left and the right Cayley graphs of S. [4] (c) Find the R-, L -, and D-classes of S.
EXAM DURATION: 2 hours
EXAM INSTRUCTIONS: Attempt ALL questions.
The number in square brackets shows the maximum marks obtainable for that question or part-question.
Your answers should contain the full working required to justify your solutions.
PERMITTED MATERIALS: No calculators
YOU MUST HAND IN THIS EXAM PAPER AT THE END OF THE EXAM.
PLEASE DO NOT TURN OVER THIS EXAM PAPER UNTIL YOU ARINSTRUCTED TO DO SO.
1. 数学半群代考
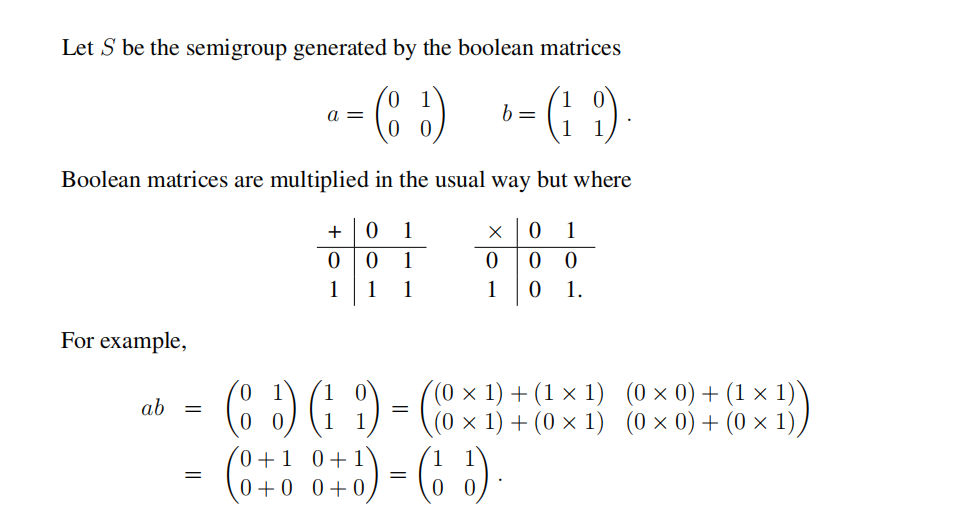
(a) Show that S has 6 elements. [4]
(b) Draw the left and the right Cayley graphs of S. [4]
(c) Find the R-, L -, and D-classes of S. Draw the partial order of the D-classes of S. [4]
(d) Show that S has 3 two-sided ideals and describe the Rees quotient of S by each of these ideals. [5]
(e) State the definitions of:
(i) a regular semigroup; [1]
(ii) the inverse of an element in a semigroup; [1]
(iii) an inverse semigroup. [1]
(f) Is S a regular semigroup? Is S an inverse semigroup? [3]
(g) Prove that an element of a semigroup is regular if and only if it has an inverse. [2]
2. 数学半群代考
A semigroup is called a rectangular group if it is isomorphic to a direct product of a group and a rectangular band. In other words, the semigroup S is a rectangular group if S ≌ G × R = {(g, r) : g ∈ G, r ∈ R} where G is a group and R is a rectangular band, with multiplication defined by
(g, r)(h, s) = (gh, rs).
A semigroup is orthodox if its idempotents form a subsemigroup.
Let G denote a finite group and R denote a finite rectangular band.
(a) State the definition of a rectangular band. [1]
(b) Let e denote the identity of G. Prove that {e} × R is the set of idempotents in G × R. Deduce that G × R is an orthodox semigroup. [3]
(c) State the definition of a simple semigroup. [1]
(d) Show that the rectangular group G × R is a simple semigroup. [4]
(e) State the Rees Theorem without giving a proof. [2]

(g) Show that for every i ∈ I and λ ∈ Λ there exist qi , rλ ∈ G such that pλ,i = rλqi . [5]
(h) Let I × Λ be a rectangular band, and denote the direct product of G and the rectangular band I × Λ by G × (I × Λ). Prove that ∅ : S → G × (I × Λ) defined by
(i, g, λ)∅ = (qigrλ,(i, λ))
is an isomorphism. Deduce that S is a rectangular group. [4]
(i) Show that a finite semigroup is simple and orthodox if and only if it is a rectangular group.

