ME 5554 / AOE 5754 / ECE 5754
FINAL EXAM – FALL 2019
机械工程代考 BEFORE YOU BEGIN: Please check to see that you have all six pages! There are four numbered problems. Please read each problem CAREFULLY!
Sign the Honor Statement: 机械工程代考
I pledge that I have neither given nor received unauthorized aid on this exam:
Please SIGN your name:
Please PRINT your name:
Exam Rules:
- You may use a scientific calculator that is NOTprogrammable
- You may use one (1) page of notes (1 front side and 1 backside)
- You may NOT use a laptop computer, PDA, Blackberry, iPhone, or Cellphone
- You may NOT use any text book or referencebook
- You may NOT use MATLAB or any equivalent processingsoftware 机械工程代考
- You may NOT discuss any part of this exam withanyone
- You MUST work on this exam by yourself with NO input fromanyone
- You MUST show ALL of your work!!! NO partial credit will be given for submissions that do not show all of the work.
BEFORE YOU BEGIN: Please check to see that you have all six pages! There are four numbered problems. Please read each problem CAREFULLY!
1.(10 pts) An open-loop LTI plant is constructed by cascading two dynamic systems together, where the output of dynamic system 1 is the input to dynamic system2:
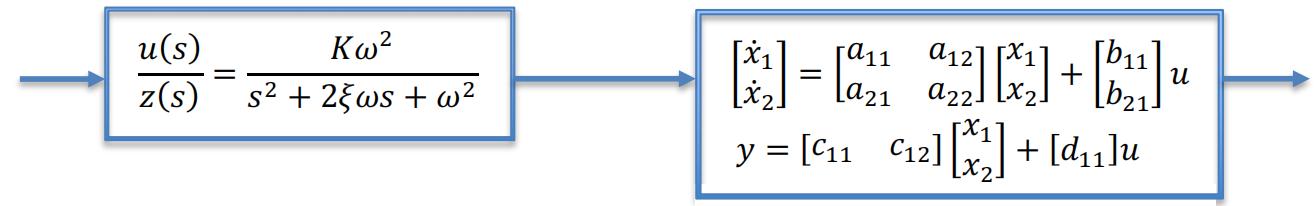
Construct the open-loop state-space representation for the complete cascaded system with z as the input and y as the output, by defining the A, B, C, and D matrices. Hint: ALGEBRA!!!
2.(10 points) Use the eigenvector matrix and its inverse (below) to transform the state- space system above into modal (i.e. diagonal) form. Inspect your result to determine which modes (or mode) are uncontrollable and which are unobservable. Explain which mode is dominant and why. 机械工程代考
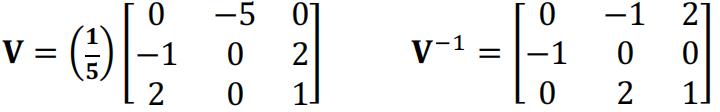
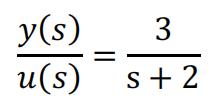
An optimal (LQR) state-feedback gain (G) will be implemented for the first order SISO dynamic system above. The LQR cost function is given by:
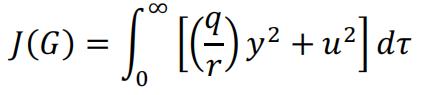
The pre-computed LQR solution for the optimal state-feedback gain (
) as a function of the relative cost weighting factor LZM for this dynamic system is given below. Note:Evaluating the function below is equivalent to calling the Matlab LQR function with Q and R weighting matrices to generate the G output.
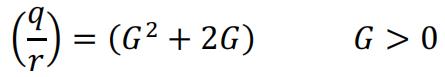
3a. (5 points) Derive an expression for the closed loop pole as a function of the state- feedback control gain. Hint: Convert the transfer function above to state-space first. 机械工程代考
3b. (3 points) Determine is the LQR optimal state-feedback gain when the output weighting is 15 times larger than the control weighting? What is the closed-loop pole associated with this gain?
3c. (2 points) Determine the relative cost weighting factor LZM associated with a closed-[loop LQR optimal pole at -5 ?
A multi-input open-loop plant is given by the following state-space realization with control input u, process noise w, and measurement noise θ:
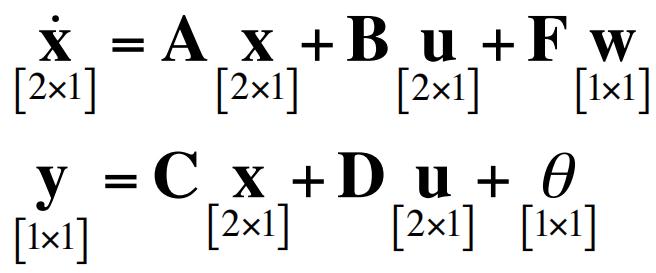
An output-feedback control system has been designed that includes full state feedback control, integral control for reference tracking, and state estimation. The following state- space realization was constructed to simulate this complete closed-loop system. In this model, perfect knowledge of the open-loop matrices has been assumed.
4a. (3 pts) Determine the open-loop A, C, and F matrices. 机械工程代考
4b. (3 pts) Determine the full state feedback ( G0) and the integral control (G1) gains.
4c. (2 pts) Determine the state estimation gains (K).
4d. (2 pts) Determine the open-loop D matrix.
