Econ 390 Midterm Exam I
线性回归模型代写 Question 1: TRUE/FALSE. State if the following statements are true or false. Only clearly and correctly explained answers will earn full credit.
Question 1: (20=4*5 pts)
TRUE/FALSE. State if the following statements are true or false. Only clearly and correctly explained answers will earn full credit.
(a) The sample correlation of the OLS residuals with explanatory variable is positive or negative dependent on whether the explanatory variable is mostly positive or negative.
(b) R² is a measure of how close the values of the dependent variable yi are to the estimated regression line: a larger value of R² indicates that the yi ’s are closer to that line.
(c) The following linear regression model that explains CEO annual salary (salary, in $1,000) in terms of annual fifirm sales (sales, in $1,000) was estimated:
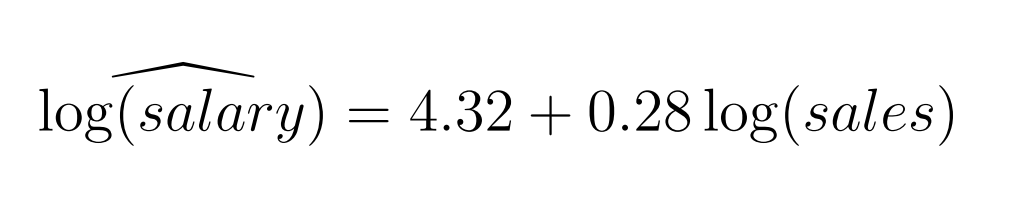
(d) In the following model, the slope coeffiffifficient for log(sales) is not statistically signifificant at the 1% level.
Question 2: (15 pts) 线性回归模型代写
Below you are asked to decide on whether or not to use a one-sided alternative or a two-sided alternative hypothesis for the slope coeffiffifficient. Brieflfly justify your decision and state the corresponding null and alternative hypotheses in terms of model’s parameter(s).
(i) Model:
CourseEval = β0 + β1OneCredit + u,
where CourseEval is course overall teaching evaluation score, and OneCreidt is a dummy variable equal to 1 if the course is a single-credit elective course; 0 otherwise.
You seek evidence that since single-credit elective courses are relatively easy, they tend to get higher teaching evaluation scores than other courses.
(ii) Model:
P B = β0 + β1P riceJelly + u,
where P B is the demand for peanut butter, and P riceJelly is the price of jelly.
You seek evidence that PB and jelly are complementary goods (the demand for one good is decreasing when the price of another good is increasing).
(iii) Model:
BMI = β0 + β1exercise + u,
where BMI is individual’s Body Mass Index, and exercise is his/her amount of physical exercise per week (in hours).
You seek evidence that exercising is an important determinant of BMI.
Question 3: (35 pts) 线性回归模型代写
The concept of purchasing power parity or PPP (“the idea that similar foreign and domestic goods should have the same price in terms of the same currency,” Abel, and B. Bernanke, Macroeconomics, 4th edition) suggests that the ratio of the Big Mac priced in the local currency to its U.S. dollar price should equal the exchange rate between the two countries.
After entering the data for 120 countries (where McDonald’s restaurants operate) into your spreadsheet program, you calculate the Big Mac exchange rate per U.S. dollar by dividing the price of a Big Mac in local currency by the U.S. price of a Big Mac.
To test for PPP, you plan to regress the actual exchange rate (XRate) on the “Big Mac exchange rate” (BMXRate):
XRate = β0 + β1BMXRate + u
(a) If PPP indeed holds, what does it imply for coeffiffifficients β0 and β1? That is, if the ratio of the Big Mac priced in the local currency to the U.S. dollar price is equal the actual exchange rate between the two countries, what should be the relationship between actual and Big Mac exchange rates and what does it mean for the values of β0 and β1?
(b) Interpret the R2 for this regression:
(c) Interpret the slope coeffiffifficient for this regression:
(d) Test your null hypothesis about β0 from part (a) at the 5% signifificance level. Justify the use of a one-sided or a two-sided test.
(e) Test your null hypothesis about β1 from part (a) at the 5% signifificance level. Justify the use of a one-sided or a two-sided test.
(f) Based on your hypotheses tests, what is your conclusion: Does the PPP principle hold in real life?
(g) Find the 99% confifidence interval for the slope coeffiffifficient:
Question 4: (20 pts) 线性回归模型代写
A psychology professor wants to measure the effffect of time pressure on exam scores, so he gives each of his 400 students the same exam, but some students get 90 minutes to complete the exam, some get 105 minutes, and some get 120 minutes. Each student is randomly assigned to one of the three examination times (say, by rolling a die). After the exams are graded, the professor plans to estimate the following model:
Scorei = β0 + β1T imei + ui
where Scorei is the number of points scored on the exam by student i, and T imei is the amount of time that student i had to complete the exam (so T imei = 90 or 105 or 120).
(a) Explain what the ui variable represents here and give at least three specifific examples of factors contained in the error term ui.
(b) Explain why in this model it may be reasonable to assume that E(ui|T imei) = 0.
(c) The estimated regression is
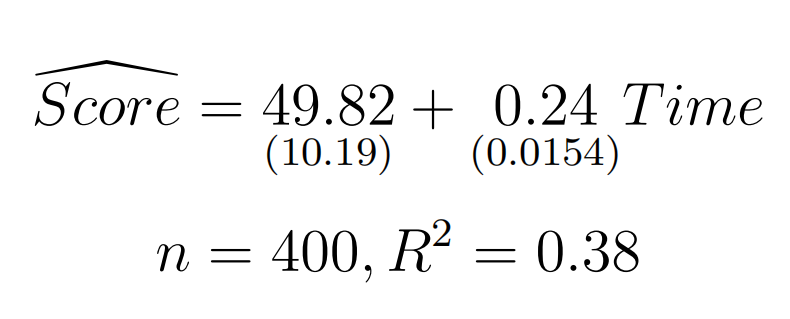
where standard errors are in parentheses.
(i) What is the predicted score for a student who is given 90 minutes to complete the exam? 100 minutes? 120 minutes?
(ii) You seek evidence that time pressure has a negative effffect on exam scores. Specify the null and alternative hypotheses corresponding to that scenario and test your hypotheses at the 1% signifificance level. What is your conclusion?
Question 5: (10 pts) 线性回归模型代写
Management of a large fast-food chain wants to study the causal effffect of competition on sales, so they propose estimating the following regression model:
Salesi = β0 + β1NumCompi + ui
where Salesi are sales (in $1000s) at store i, and NumCompi is the number of competitors within one mile of store i. They have data on 650 randomly selected stores.
(i) What are other factor (besides the number of competitors) that can affffect fastfood sales? List at least three and explain why you think that these factors can inflfluence fast-food sales.
(ii) Can any of those factor be correlated with the number of competitors? Explain.

其他代写: 作业代写 作业加急 英国代写 北美作业代写 homework代写 java代写 matlab代写 program代写 project代写 essay作业代写 finance代写 python代写 report代写 paper代写 assignment代写 加拿大代写
