MAT591: Optimization (Spring 2020) Homework 3
Homework 3: Statistical Estimation and Unconstrained Optimizaton
MAT591Optimization代写 Problem 1 (20 points):Consider a random variable x ∈ Rᵏ with values in a fifinite {a1, a2, . . . , an}, and with distribution
Requirements
- Name your report as MAT591-HW#-YOUR-NAME.pdf, and send it to to my email address [email protected]
- Write your name in BLOCK CAPITALS on top of your submission.
- You are required to answer all the questions.
- Clean and easily understandable handwriting is required. The instructor is not responsible for any misunderstanding caused by bad handwriting. Five points are reserved for presentation.
- The deadline shown as above is a hard deadline; generally any late submission will not be marked!
Problem 1 (20 points) MAT591Optimization代写
Consider a random variable x ∈ Rᵏ with values in a fifinite {a1, a2, . . . , an}, and with distribution
pᵢ = prob(x = aᵢ), i = 1, 2, . . . , n.
Show that a lower bound on the covariance of X,
S ≤ E(X − EX)(X − EX)ᵀ
is a convex constraint in p.
Problem 2 (20 points) MAT591Optimization代写
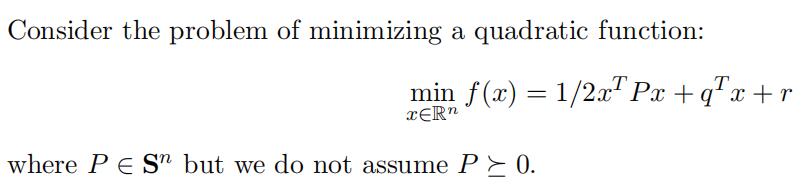
(a) Show that if P is not positive semi-defifinite, i.e. the objective function f is not convex then the problem is unbounded below
(b) Suppose that P ≥ 0 (so f is convex) but the optimality condition P x∗ = −q does not have a solution. Show that the problem is unbounded below.
Solution: [Type your solution here.]
Problem 3 (30 points) MAT591Optimization代写
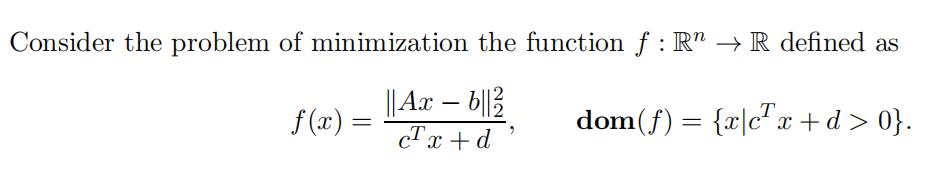
Assume that rank(A) = n and b /∈ R(A)
(a) Show that f is closed
(b) Show that the minimizer x∗ of f is given by
x∗ = x₁ + tx₂
where x₁ = (Aᵀ A)﹣¹Aᵀ b, x₂ = (Aᵀ A)﹣¹c and t ∈ R can be calculated by solving a quadratic equation.
Solution: [Type your solution here.]
Problem 4 (20 points)

Solution: [Type your solution here.]
Problem 5 (10 points) MAT591Optimization代写
Complete the following questions.
(a) Explain how to fifind a steepest descent direction in the ℓ∞-norm, and give a simple interpreta-tion.
(b) Write down the defifinition of the Newton decrement λ(x) and work out its explicit form.
Solution: [Type your solution here.]

其他代写:homework代写 Exercise代写 course代写 essay代写 app代写 algorithm代写 assignment代写 analysis代写 code代写 assembly代写 CS代写 Data Analysis代写 data代写 作业代写 作业加急 加拿大代写 北美代写 北美作业代写 澳大利亚代写 英国代写
合作平台:essay代写 论文代写 写手招聘 英国留学生代写

