Midterm Exam
Advanced Econometrics I, Fall 2015
高级计量经济学代写 Consider a 90% confidence interval of a parameter θ. It means that the prob-ability that θ is within the interval is 90%.
Check the point for each question and do not spend too much time on any one question.
Question 1 (20)
True/False questions: please answer whether the following statements are true or false. No explanation is needed, but you can add some clarification if you think you need.
1. (5) The Gauss-Markov theorem states that when all standard assumptions are satisfied, the least squares estimator has the smaller variance than any linear unbiased estimators. 高级计量经济学代写
2. (5) Consider a 90% confidence interval of a parameter θ. It means that the prob-ability that θ is within the interval is 90%.
3. (5) For any two chi-squared (x2) random variables with the same degrees of free-dom, their ratio is an F random variable.
4. (5) Consider a multiple regression where all independent variables are significant at 10% significance level. If you drop a non-constant independent variable, the adjusted goodness-of-hit always decreases.

Question 2 (10) 高级计量经济学代写
Assume that the sequence of the random vector (XN, YN)’ satisfy the following condition:
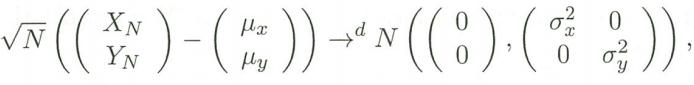
where μx≠0 and μy≠0.
1. (5) Find the asymptotic distribution of 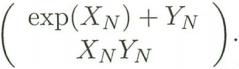
2. (5) Under what condition, would the asymptotic covariance between exp(XN)+YNand XNYN be zero?
Question 3 (10) 高级计量经济学代写
Consider a multiple regression of yi on K independent variables: yi = β1xi,1 十….十
βKxi,K + ∈i, where the first independent variable is a constant. This question asksyou to derive the expression for βk (least squares estimator of Bk). For this purpose,consider another regression of xik on xi,- -k, where xi,-k = {x,,..,.i,k-1, xi,k+…..i,K}for k > 1. Then, we can partition xik into two parts: xik = k + rik, where xik is the part of xik explained by xi,- -k and rik is the unexplained part (residual).
1. (5) Note that (β1…,βk)’is the solution of 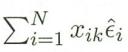 for all k=1 …K.Using this fact, find the expression of βi in terms of rik and yi. (Clearly show each step of derivation.)
for all k=1 …K.Using this fact, find the expression of βi in terms of rik and yi. (Clearly show each step of derivation.)
2. (5) What is the interpretation of βk? Explain it using the meaning of rik.
Question 4 (35)
Assume that all standard assumptions are satisfied. A multiple regression of y on a constant, x1, and x2 produces the following results:
yi=4 – 1.4xi1 + 2.1xi2,for i= 1…N,
with R2=8/60,![]() = 500, and
= 500, and
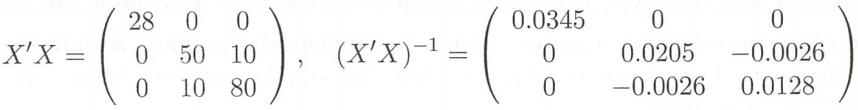
1. (5) Let the constant be β0 and the two slopes be (β1,β2). You want to test the hypothesis that the two slopes sum to 1. Express the null hypothesis in the form of Rβ=q, where β= (β0,β1, β2)’.
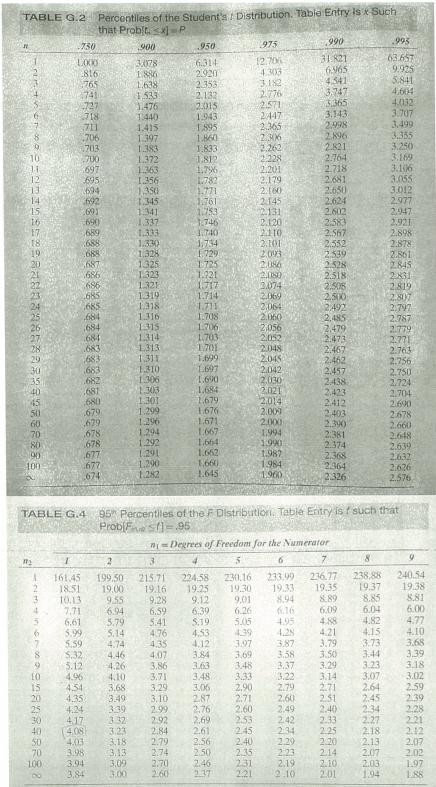
2. (10) Compute the test statistic. Is the null rejected at the 5% significance level?What about at the 1% significance level? (In each case, clearly state the critical value that you are comparing the test statistic with.) 高级计量经济学代写
Hint: See the tables in the appendix.
3. (10) Now you want to construct the confidence interval of the slope of x1. What is the 95% confidence interval? Does the confidence interval contains 0? What about at the 99% confidence interval? Based on the confidence intervals you constructed,test Ho: β1 = 0.
4. (5) For given independent variables (xo1, xo2) = (1,2), construct the 95% predic-tion interval of yo.
5. (5) What is the adjusted goodness-of-fit (R2)?
Question 5 (25) 高级计量经济学代写
Using the national time series data, you run the linear regression of real consumption (c) on a constant and real disposable income (x):
Ct =αc+βcxt+∈c,t, t= …T.
As the regression result, you obtain 2 × 1 vector of cofficient estimates (αc,βc)’, T× 1 vector of fitted values (C) and T × 1 vector of residuals ((c). You also run another regression of real savings (s) on the same independent variables, a constant and x,obtaining (αs,βs)’, 8 and ig. Note that the sum of real consumption and real savings is real disposable income, i.e., c+s= x.
1. (10) Compute(i)&c +as,(i) βc+βs, (ii)i+s, and (iv)![]() .
.
2. (5) Show that the residual sum of squares from the consumption regression (![]() ) is equal to the residual sum of squares from the savings regression (
) is equal to the residual sum of squares from the savings regression (![]() ). 高级计量经济学代写
). 高级计量经济学代写
3. (5) State true or false and explain: The R2‘s are the same for the two regressions.
4. (5) Consider the null hypothesis that the marginal propensity to cosuIC (MIPC) is equal to one.1 Explain how to test this null hypothesis based on each equation.Will the test results be consistent across those two regression equations? In other words, will the test result be invariant to whether the consumption regression is used or the savings regression is used?
1MPC is the proportion of additional real consumption to additional real disposable income: