Homework Assignment 4
英国商科作业代写 Mike is a used bikes salesman. He needs to transport his bikes from locations 1 and 2 to an auction site on location 4.
Problem 1 (30 points)
Use the data from “Beating the Market Quarterly” problem. Use that data to estimate expected returns and a covariance table for the 5 stocks from that problem. Use yourestimates to find the portfolio that maximizes return among portfolios that have standard deviation of no more than 10%. Assume you’re required to hold a position in each of the five stocks and this position needs to be between 5% and 30%.

Problem 2 (35 points) 英国商科作业代写
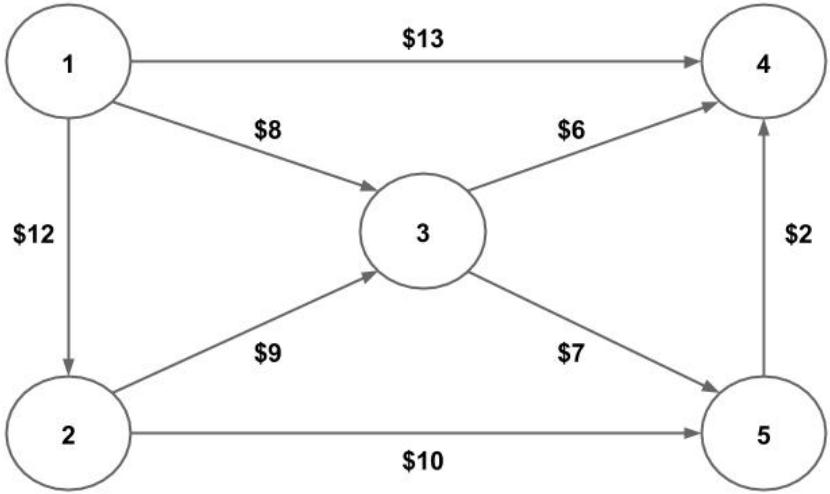
Mike is a used bikes salesman. He needs to transport his bikes from locations 1 and 2 to an auction site on location 4. He currently has 17 bikes in location 1 and 21 bikes in location 2, and he wants to transport them all to location 4.
Mike is planning to use an Uber-like service to rent vans to transport bikes between locations. A van can fit up to 5 bikes, and the costs of renting the vans are given in the network below. Each van rental covers exactly one edge in the network. The costs are pervan, not per bike. There are no limits on how many vans can be rented.
What should Mike do?
Problem 3 (35 points) 英国商科作业代写
In the year 2525, Mars is inhabited by humans who live in the Headquarters. Water is a very expensive resource. The latest drilling expeditions have discovered water in two remote locations, Water Location 1 and Water Location 2. We assume that the terrain of Mars is a 2-dimensional plane, which means that every location is defined by its x and y coordinate. The coordinates of the Headquarters and the two water locations are given in table below. A unit change in one coordinate represents a one mile distance.
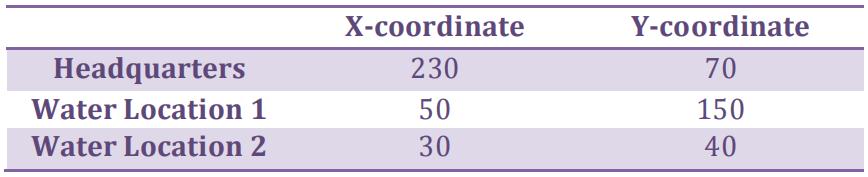
The settlers are planning to install a pipeline to carry water from the two locations to the Headquarters. However installing the pipeline on Mars’ rocky surface is a very expensive undertaking; therefore the amount of pipeline required should be minimized. The task is assigned to two analysts.
(a) (5 points) Since the shortest distance between two points is a straight line, the first analyst proposes that a separate pipe should be run from each Water Location to the Headquarters. In the year 2525, the distance between two points P1,P2 with coordinates (x1,y1),(x2,y2)is still given by the same old formula:
![]() Compute the number of miles of
Compute the number of miles of
pipes needed to implement the first analyst’s solution. 英国商科作业代写
(b) (30 points) The second analyst has a different idea: build an Intermediate Substation and run pipes from each Water Location to the Intermediate Substation, and then from the Intermediate Substation to the Headquarters. Assume that building the Substation is costless. Come up with a nonlinear optimization problem to determine where to build the Substation.

更多代写:数学课程辅导 sat考試作弊 统计网课托管推荐 香港商业essay代写 市场学论文代写 音乐专业论文写不出来怎么办
