Statistics I – Final Exam
统计exam代考 1.The Gym teachers from a junior school organized two end of year activities: a peddy paper and a treasure hunt. Kids were then asked to
Define all events, random variables, etc. Be thorough in your justifications.
Exam Rules
Students who have a legitimate justification (via Fenix) may take the exam online. The exam will be made available on the Fenix platform (PDF format). Students must solve the exam in separate sheets of paper, which must be scanned (or photographed) and sent to [email protected]. Students with grades greater than or equal to 17 will be subject to oral examination. The exam is open book.
- Exam duration: 120 min + 15 min (tolerance) [students with special education needshave been taken into account]
- Students must carry their personal ID.
- Students are not allowed to use cell phones, headsets, headphones or any other similardevice.
- Students are not allowed to communicate between them or with any other third party during the exam.
- In case of failure to comply, namely due to: usage of cell phones or other personal electronic device, copy or any other type of information exchange with other students will result in annulment of the students’ exam.
- Failure to comply will result in the interruption of the exam. A report may be elaborated on the occurence, signed by the professor and sent to the Rectory.
- No questions are allowed during the exam.
- For students taking the exam remotely, surveillance will be made via a Zoom meeting. Students must have their camera turned on and their microphone muted. The teacher must be able to see and identify the student during the whole exam.
1. 统计exam代考
The Gym teachers from a junior school organized two end of year activities: a peddy paper and a treasure hunt. Kids were then asked to rate on a 0 (hated it!) to 10 (absolutely loved it) scale how much they had enjoyed the activities. Some results from the survey are on tables 1 and 2.
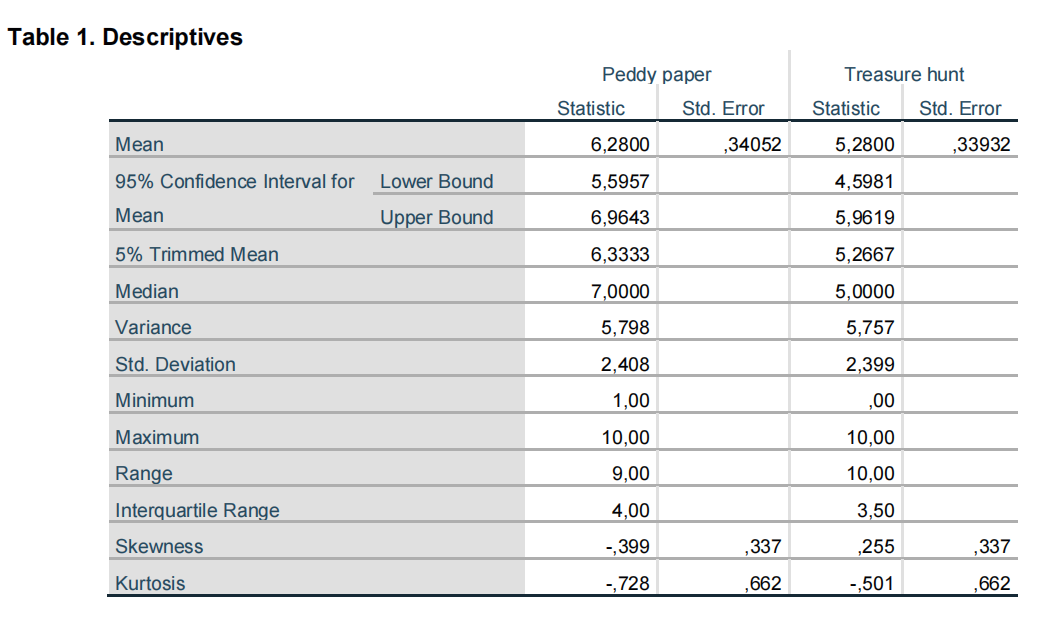
Table 2. Percentiles
| Percentiles | Peddy paper | Treasure hunt |
| 5 | 2,0000 | 1,5500 |
| 10 | 3,0000 | 3,0000 |
| 25 | 4,0000 | 3,7500 |
| 50 | 7,0000 | 5,0000 |
| 75 | 8,0000 | 7,2500 |
| 90 | 9,0000 | 8,9000 |
| 95 | 10,0000 | 10,0000 |
(1,0) a) Sketch the box-and-whiskers plots
(1,0) b) Characterize the two distributions for symmetry.
(1,0) c) Which activity have kids liked the most? Justify.
2.
Let X represent the number of times per day a production line stops and Y the number of workstations within the line. The joint probability mass function for the pair (X,Y) is provided below:
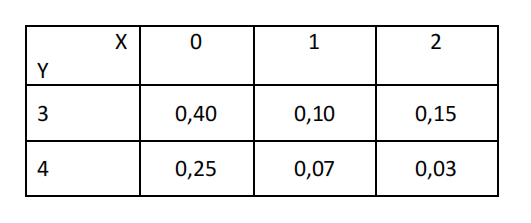
(1,0) a) Calculate the marginal probability functions of X (number of times per day a production line stops). Interpret its meaning.
(0,5) b) Calculate the average number of stops per day.
(1,5) c) Calculate the probability that a production line, known to have 4 workers, records at least 1 stop during one day.
(1,5) d) Calculate Cov (2X,Y).
3. 统计exam代考
The number of defects per 100m of steel wire can be described by a Poisson random variable with λ=6.
(0,5) a) Calculate the probability of registering 3 defects in a 100m reel of steel wire.
(0,5) b) Calculate the probability of registering 5 defects in a 100m reel of steel wire knowing that in another 100m reel 10 defects were found.
(1,0) c) Calculate the probability of registering 3 defects on a smaller 30m reel.
(1,5) d) Calculate the probability that in 7 reels with 100 meters of steel cable each, none has 3 defects.
4. 统计exam代考
The time Worker 1 spends to complete a certain task can be described as a normalrandom variable with a mean 1 minute and standard deviation 0,3 minutes.
(1,5) a) Compute the probability that Worker1 spends more than 2 minutes to complete the task.
(1,5) b) Assuming independence between repetitions of the same task, compute the probability that Worker1 spends less than 8.5 minutes to complete the same task 9 times.
(1,5) c) Worker2 is also doing the aforementioned task. The time spent by Worker2 to complete the task can also be described by a normal random variable with mean 1,5 minutes and standard deviation 0,5 minutes. Compute the probability that the time spent by Worker1 is greater than the time spent by Worker2 to complete the same task.
(1,5) d) If Worker1 is going to perform that task 100 times, calculate the probability that he will spend more than 1 minute in 40 of those 100 repetitions.
5. 统计exam代考
(1,5) Let X and Y be the following independent random variables:

6.
(1,5) Let (X1, X2, …, Xn) be a n-dimensional random sample with n> 2, drawn from a population with mean μ and variance σ2 . Let T1 and T2 be two different estimators for the mean:
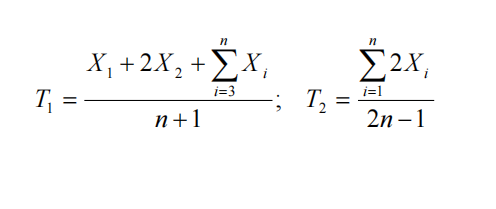
Can you compare the relative efficiency of these two estimators? Justify.

更多代写:计算机包网课机构 考试枪手 英国math数学网课代上 北美assignment代写 北美HIS历史学论文代写 essay写手代写
