Econ 659 Practice Problems 1
经济学课业代写 1.In parts (a) through (c) below, use Ito’s lemma to write the stochastic process y in the standard form dy = a(y, t)dt + b(y, t)dz.
1.
In parts (a) through (c) below, use Ito’s lemma to write the stochastic process y in the standard form dy = a(y, t)dt + b(y, t)dz. dz is a standard Wiener process.
(a) y = z2
(b) y = 2 + t + ez
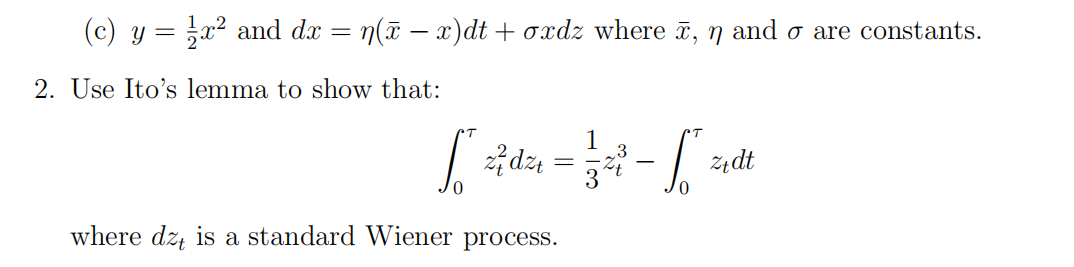
3.
Suppose you are participating in a game in which you roll a six sided dice and will win the value of whatever you roll times 1000. You can roll up to 4 times. After each roll you can either accept the prize given by that roll, or reject it and roll again. Let Vi denote the expected value of your prize assuming you act optimally over the entire game from roll i and onward. Let Wi denote the number on the dice for the ith roll. Find the expected value of your prize, V1, as well as V2, V3, and V4. Determine the optimal strategy.
4. 经济学课业代写
Consider a 1 year futures contract on an investment asset that provides no income and has no convenience yield. (An investment asset is one that is held by a significant number of individuals as an investment, rather than for consumption. Gold and silver would be examples.) It costs $10 to store the asset for a year, payable at the beginning of the year. The current spot price of the asset is $200 and the risk free interest rate is 5%.
(a) Suppose the futures price is $230. Demonstrate whether this is an equilibrium price. If not, explain how an arbitrager can make a risk free profit.
(b) Suppose the futures price is $210. Demonstrate whether this is an equilibrium price. If not, explain how an arbitrager can make a risk free profit.
(c) Discuss how the actions of arbitrageurs would be expected to cause prices to adjust to an equilibrium level.
5. 经济学课业代写
An individual enters into a one year long forward contract on an investment asset with no income or storage cost when the asset’s spot price is $50 and the risk free rate of interest is 5% perannum (with continuous compounding).
(a) What are the forward price and the initial value of the forward contract?
(b) Six months later, the price of the stock is $55 and the risk free rate is still 5%. What are the forward price and the value of the forward contract? Explain why these have changed.
6. 经济学课业代写
In class we derived the relationship between the spot and futures price of an asset. For commodities, that relationship is ft= Ste(r+u−δ)τwhere ft is the futures price at time t, St is the spot price, u is the storage cost, δ is the convenience yield and τ is the time to maturity of the forward contract. We now assume that δ includes storage costs, so we drop u and are left with
ft = Ste(r−δ)τ .
Recall that r − δ is the risk adjusted drift rate of the asset’s price in the risk neutral world. Suppose the asset S has a constant expected growth rate as in a GBM process: dS = αSdt + σSdz. Then we know that the expected value of S in the risk neutral world is given by:
E(Sτ ) = Ste(r−δ)τ .
From Equations (2) and (3), we see that the futures price f is the expected future spot price in the risk neutral world.
Suppose you are given the following information regarding light crude oil futures prices on Feb 20, 2010 in $US/barrel:
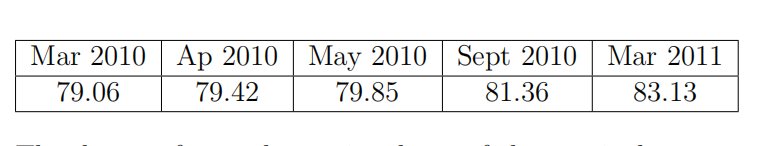
The dates refer to the expiry dates of the particular contracts. Expiry dates are on the closest business day to the 20th of the month. Use this information to estimate the risk adjusted drift rate for the price of oil between March 2010 and April 2010.
Compare this to the rate implied between March 2010 and Sept 2010, as well as between March 2010 and March 2011. Assuming a risk free rate of 1%, what are the implied convenience yields?

