CS 2100: Discrete Structures
Quiz 2
Online Exam Pledge 离散结构quiz代写
Any violation of these rules and additional rules stated in the Academic Misconduct Policy of CS 2100 will result in an Academic Misconduct filing.
- Do not communicate with anyone else (especially other students in the course) about the exam.
- Do not search or solicit solutions to exam questions online or elsewhere.
- Do not submit as one’s own work that is copied from another student or an outside source (including any online source).
- Do not violate any rule as detailed within the Academic Misconduct Policy of CS 2100.
- If you are aware of any efforts to violate these rules (including others communicating with you), it is your duty to report those violations to the instructor. A failure to communicate to the instructor a violation of these rules is itself a first class Academic Misconduct.
Allowable sources include: 离散结构quiz代写
- An 8.5×11” index card of notes (double-sided, handwritten, or print, with any texts on the card not smaller than 8 points font size).
The exam is close book, close notes.
Any resource that is not part of the allowable sources is considered an outside source and therefore is not allowed.
By signing below, I pledge that (a) I did not violate any of the above take home exam rules, and (b) I fully understand the Academic Misconduct Policy of CS 2100 and I did not violate any additional rules stated within the Academic Misconduct Policy.
Sign Here:
UID:
Online Exam Rules 离散结构quiz代写
Please submit your solutions together with the signed pledge page and your 2-page index card (as a scanned image or in PDF). Your submission will not be graded if you do not sign and submit the pledge page. It will also not be graded if you do not submit your index card.
Please read these instructions regarding the exam carefully.
To work on the exam, there are a few options (similar to solving homework problems):
– You can write your answers by hand, then submit a photo or a scanned image in PDF.
Please use a black pen. The handwritten solutions must be legible. The scanned or photo image must be in reasonably high resolution to be readable.
– Any solutions that are unreadable/illegible will not receive any points. 离散结构quiz代写
– Alternatively, you can use the provided WORD template or the LATEX template to add your solutions, then submit a converted/compiled PDF.
– You can also download the provided exam PDF and use Adobe or Preview (or similar tools) for editing your solutions directly on the PDF file.
– Please do not put more than one problem on each page. If you need more space to provide a solution to a problem, use a blank piece of paper or a blank digital page.
– Please do not use a single page for more than one problem.
– For submission, we only need your solution to each problem together with (a) the signed pledge page and (b) the PDF of your index card.
– Please match each problem to the outline specified on Gradescope: the page number containing the answer to each individual question should be specified during submission.
– If no pages are specified for each problem, then 20% is deducted from the final score.
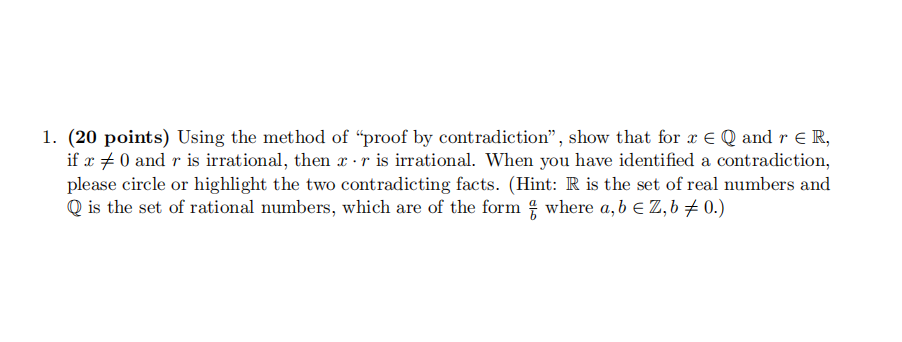
2.(10 points)
Using a direct proof, show that if a|b and a|c, then a2|(b · c). (Note: a|b means “a divides b”.)
3.(20 points) 离散结构quiz代写
Using the method of “proof by cases”, show that for all n ∈ Z, 3|(n3−n). (Hint: 3|(n3− n) means “3 divides n3 − n”.)
4.(10 points)
Using the method of “proof by contrapositive”, show that if n3+ 4n − 3 is not divisible by three, then n is not divisible by three. (Hint: start by writing the contrapositive.)
5.(20 points) 离散结构quiz代写
Using the pigeon hole principle, show that for any set of 13 integers, there are always two members of the set whose sum or difference is divisible by 13. Please explicitly state what your boxes are, how numbers are assigned to the boxes and what condition you are looking for.
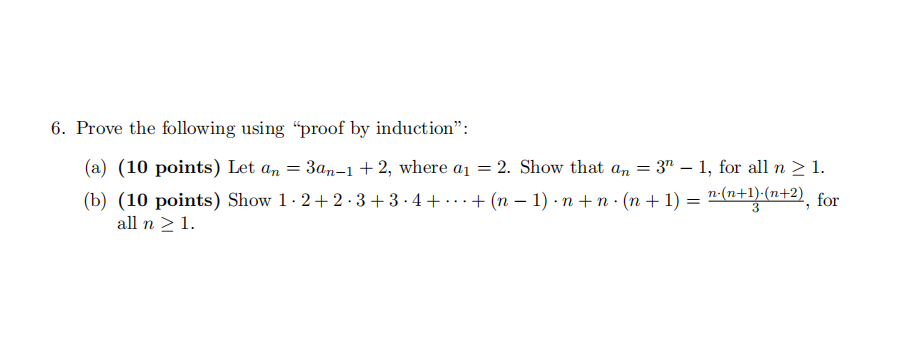

更多代写:cs澳大利亚网课代修价格 toefl代考 英国理工科代写 空间设计Essay代写 澳大利亚econ Midterm代考 金融工程编程课业代写
