A PHY 335Z EXPERIMENT 1
STATISTICS AND UNCERTAINTY
物理实验代写 SUMMARY We will perform a crude scattering experiment to measure the radius of a set of identical circles illustrated on a sheet of paper.
SUMMARY
We will perform a crude scattering experiment to measure the radius of a set of identical circles illustrated on a sheet of paper.
The technique we will employ will be to either throw/drop a pen or pencil at/onto the sheet of paper. We can treat this as a scattering experiment where one can label each event as a hit or a miss. From the number of hits and misses we will estimate both the radius and the area of the circles as well as the uncertainties in those estimates.
The second technique will employ a wooden meter stick from the lab where one will measure the diameter of the circles directly. From this list of measurements, we will come up with an alternate estimate of the radius and area of the circles.
THEORY 物理实验代写
Physics relies on evaluating the ability of competing theories to make predictions about measurements or data in general. Consider an example where Theory A predicts that a measurement of the radius of a circle is 5cm and Theory B predicts that it should be 4cm. You carry out an experiment and take a measurement to find that you obtained the circle diameter to be 4.4cm. Clearly this measurement does not agree with either theory. But does it rule out one theory or both?
Measurements aren’t perfect. Not all situations are identical. Not all equipment is accurately calibrated.
That is, errors can come from many sources: systematic error (bias), measurement precision, or statistical variation. Systematic error or bias can arise from not taking into account an important physical effect in the experiment, comparing the measurements to a theory that makes inappropriate assumptions (which is very similar to the first example), or working with poorly calibrated equipment. 物理实验代写
Measurement precision reflects one’s ability to make measurements and is quantified by a measurement uncertainty. Statistical variation occurs in cases where there are many trials and one’s inability to make sure that the trials are identical as assumed.
When reporting results, one must be mindful of the uncertainties in the resulting estimates and this must be reflected in the number of reported significant digits. A discussion of significant digits can be found in Cutnell and Johnson appendix A-1 or in Serway chapter 1.
We will rely on some specific formulae to compute estimates and uncertainties in a variety of situations.
Many of these will be derived in class and provided in handouts. All can be found in Bayesian Data Analysis: A Tutorial by Sivia (first edition) or Sivia and Skilling (second edition).
EXPERIMENT INTRODUCTION 物理实验代写
A rectangular region with area A on a sheet paper has K circles drawn on it. Each
circle has radius r. The circles do not overlap or intersect. This is all you know. The picture to the right is for illustrative purposes only. Use the sheet supplied at the end of this writeup.
EXPERIMENT A
PROCEDURE
Scattering Experiment
Print out a high quality copy of the sheet with circles on the last page of this document.
We will basically estimate the area of the circles by throwing/dropping a pen or pencil at/onto the sheet of paper and counting how many times the impact falls within the circle. Another method would be to drop very small pebbles or rice onto the sheet of paper and note how many times a pebble falls within one of the circles. I will refer to pebbles in the analysis below.
What to do with the finite boundary is up to you. Be consistent and take this in account in your analysis and technical report. Do the experiment carefully and note the difficulties this method presents, and how you chose to overcome them.
ANALYSIS 物理实验代写
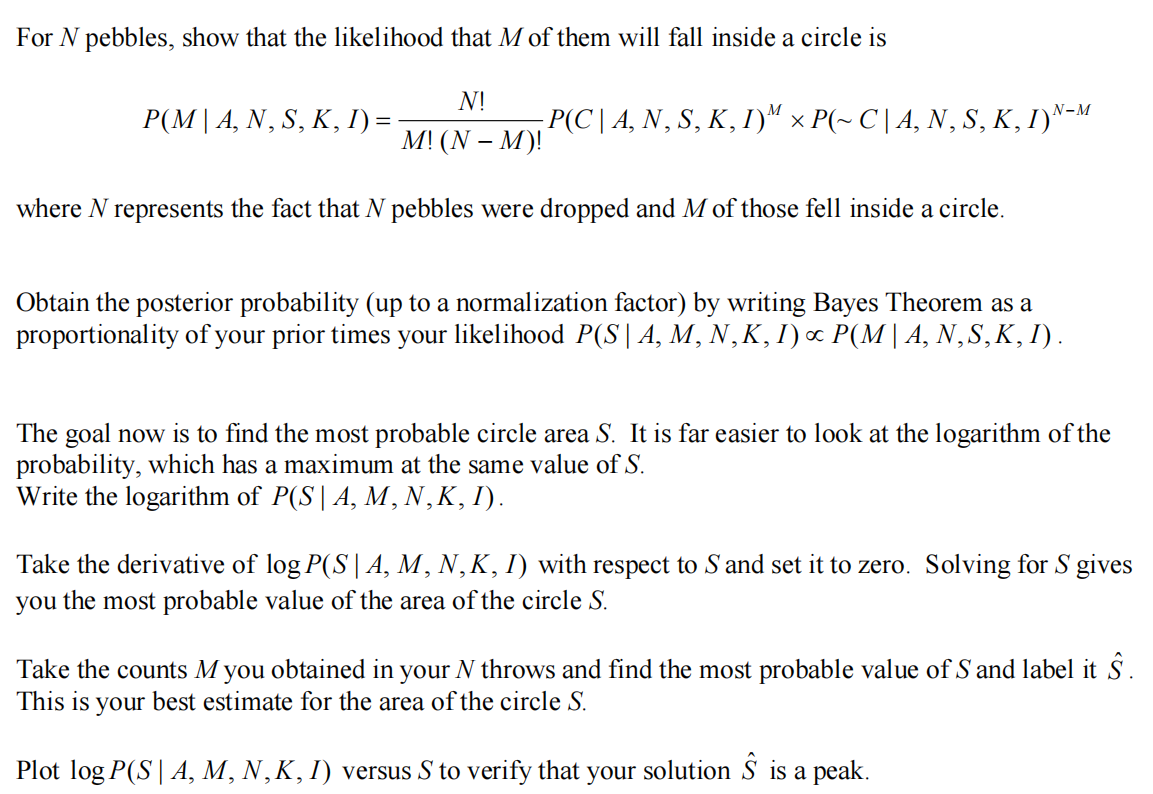
We are going to employ Bayes Theorem to derive the probability that the circles have area S given that that M pebbles fall within a circle out of N pebbles total. That is we will compute P(S | A, M , N, K, I) where I is simply a symbol which represents the prior information you possess. Bayes Theorem is then given by the proportionality
P(S | A, M , N,K, I) ∝ P(S | I)P(M | A, N, S,K, I)
which is a product of the probability that the circles have an area S and the likelihood (probability) of obtaining M hits out of N throws given that the sheet has area A and that the circles have area S. We will assign a constant probability for the prior probability of the area of the circle over some given range, and this simplifies the proportionality above to
P(S | A, M , N,K, I) ∝ P(M | A, N, S,K, I)
We will figure out the formula for P(M | A, N, S, K, I)in stages by solving the following problems:
A boy drops small pebbles (stones) onto the sheet of paper. Sometimes a stone lands in a circle.
Let A be the area of the sheet of paper (don’t plug in number for A).
Let C1 represent the statement that the pebble lands in circle number 1.
Let N=1 represent the statement that the boy has dropped one pebble.
What is the likelihood P(C1| A, N = 1, S, I) that the pebble lands on circle number 1? 物理实验代写
Given that the result P(C1| A, N = 1, S, I) has the same form for any single circle C#, find the likelihood P(C1∨ C2 | A, N = 1, S, I) that the pebble could have landed in either circle 1 (C1) or circle 2 (C2)?
From now on, we will write this as P(C | A, N = 1, S, K = 2, I) where C means that it landed in one of the K=2 circles.
Let C = C1∨ C2 ∨ C3∨K∨ CK represent the statement that the pebble lands in any one of the K circles.
What is the likelihood P(C | A, N = 1, S, K, I) = P(C1∨ C2 ∨ C3∨K∨ CK | A, N = 1, S, K, I) ?
Let ~ C represent the statement that the pebble did not land in any circle. What is the likelihood P(~ C | A, N = 1, S, K, I) ?
If two pebbles are dropped, the likelihood that both pebbles fall inside a circle is P(C | A, N = 1, S, K, I)× P(C | A, N = 1, S, K, I) . If two pebbles are dropped, the likelihood that only one pebble falls in a circle is P(C | A, N = 1, S, K, I)× P(~ C | A, N = 1, S, K, I) + P(C | A, N = 1, S, K, I) × P(~ C | A, N = 1, S, K, I).
Can you explain this?

EXPERIMENT B
PROCEDURE
Here you will determine the radius of the circle by measurement with a meter stick or ruler.
Using the same high quality copy of the sheet with circles, take a meter stick and measure the diameter of the circle to the best of your ability. You will take multiple measurements (at least 10) and use these to estimate the radius and area of the circles. Note that there are several potential situations that could lead to systematic bias. Do your best to avoid them.
Using these results, estimate the both the radius and area of the circles along with the uncertainty in the estimates. Obtaining the uncertainty in area from the estimated radius and its associated uncertainty takes some effort. We shall discuss this in class.
Given these results, how many pebbles would you have to drop in Experiment A to get the level of uncertainty in the radius obtained in Experiment B?

