MT4509 Fluid Dynamics
流体动力学代写 EXAM DURATION: 2 hours EXAM INSTRUCTIONS: Attempt ALL questions. The number in square brackets shows the maximum marks obtainable for that
EXAM DURATION: 2 hours
EXAM INSTRUCTIONS: Attempt ALL questions.
The number in square brackets shows the maximum marks obtainable for that question or part-question.
Your answers should contain the full working required to justify your solutions.
INSTRUCTIONS FOR ONLINE EXAMS:
Each page of your solution must have the page number, module code, and your student ID number at the top of the page. You must make sure all pages of your solutions are clearly legible.
1. 流体动力学代写
Consider the two-dimensional velocity field defined by u = (t − tx2 , t3y).
(a) Find the pathline (x(t), y(t)) for the fluid particle located at (0, 2) at t = 0. [2]
(b) Find the equation (x(τ ), y(τ )) of the streakline, at t = 2, passing through (0, 1). [2]
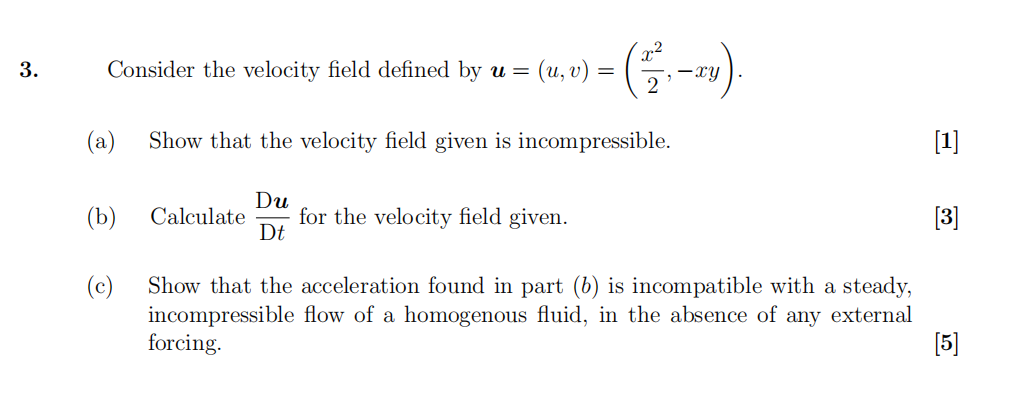
2. 流体动力学代写
Consider the two-dimensional velocity field defined by u = (x3 − 3xy2 , −3x2y + y3 ).
(a) Show that the flow is incompressible and irrotational. [2]
(b) Find the streamfunction ψ. [2]
(c) Give the general equation for the flow streamlines. [1]
(d) What are the general forms of the pathlines and streaklines for the flow? [1]

31
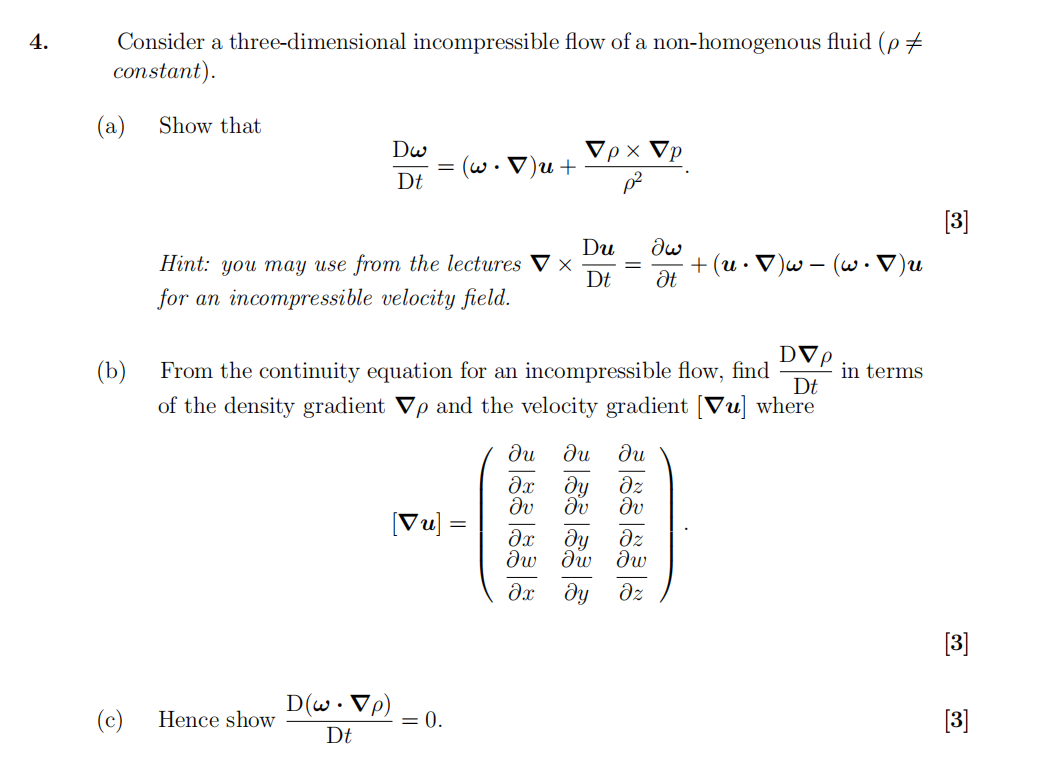
6. 流体动力学代写
A pressure probe is placed at the top of a tower at a height ht = 20 m and faces a wind blowing at uniform horizontal velocity U. It records a pressure of Pt . At the bottom of the tower hb = 0, a second pressure probe, protected from the wind, measures the same air pressure, Pb = Pt . At what speed does the wind blow? Provide all details of the calculation, clearly justifying every step. [4]
Note: You may use g = 9.81 m.s−2 , ρair = 1.225 kg.m−3 .
7. 流体动力学代写
Consider a two-dimensional flow in the (x, z)-plane. Gravity acts in the z-direction. A layer of fluid at rest of uniform density ρ2 occupies the region between z = −H and z = 0. There is a stationary solid impermeable wall at z = −H. Above this layer lies a layer of fluid of uniform density ρ1 extending from z = 0 to z → ∞, and moving at a uniform velocity u = Ui, where U is a positive constant. The fluid domain is infinite in the x-direction. You may assume that the flow remains potential for both fluids at all times. A perturbation of infinitesimal amplitude (η) is superimposed onto the interface between the two fluids,

Derive the dispersion relation σ = f(k) for the perturbation. [12]

更多代写:cs美国作弊记录 proctoru作弊 英国Argument代写 essay论文代写 芝加哥格式实例 有限数学代写
