School of Management and Marketing Curtin Singapore
Trimester 2A, 2021
Quantitative Modelling MATH2013
Individual Assignment (25%)
Due Date: Friday, September 10, 2021, by 11:59 PM through Turnitin.
新加坡数学代考 The lecturer may request an Excel copy to check on formulas used, so be prepared for this and keep a backup copy.
Instructions: 新加坡数学代考
You will download the assignment, which is a PDF file, and you need to begin to type only your answers in a Word document, excluding thequestions.
The assignment must be typed as much as possible.
Label all tables and figures correctly.
Interpretation must follow the relevant information. All results must be verified by complete workings. Single answers are generally not acceptable in any quantitative unit.
- The assignment must include a cover sheet with the student’s full details.
The lecturer may request an Excel copy to check on formulas used, so be prepared for this and keep a backup copy.
All questions must be attempted. 新加坡数学代考
On your assignment show:
Name:
Unit:
Course:
Student Identification Number:
By continuing past this point, you are acknowledging that you fully understand the instructions provided to students for this assignment
Question 1 (5 Marks): 新加坡数学代考
A company is considering manufacturing three types of electronic toys: A, B, and C. The projected profit contribution is $5.15, $7.00, and $10.00, respectively. The toys are manufactured in three stages: made, assembled, and painted. The production times in minutes are given below.
| Parts | Assembly | Paint | |
| A (x1) | 8 | 12 | 5 |
| B (x2) | 11 | 14 | 6 |
| C (x3) | 18 | 16 | 8 |
The capacity of the parts department is 500 hours per week. The capacity of the assembly department is 800 hours per week. The capacity of the paint department is 400 hours per week.
It is necessary to make at least 500 A’s and 1,000 B’s. 新加坡数学代考
a)Manuallyshow the decision variables, objective function, and constraints (at this point, you are setting out the problem. You are not solving the problem).(1 mark)
b)UseExcel to find the best weekly production plan (i.e., you will now solve the problem). State how many of each type of toy must be made and the value of the objective function. If management later decides it is necessary to produce 100 units of C, determine the reduction in profit.(4 marks)
Total (5 marks)
Question 2( 4 marks) 新加坡数学代考
An individual wishes to invest $25000 in two types: Investment A yields 15%, and investment B yields 18%. Market research suggests an allocation of at least 35% in A and at most 45% in B. In addition, investment in A should be at least twice the investment in B.
a)Show thedecision variables (0.5 marks)
b)Show theobjective function (0.5 marks) 新加坡数学代考
c)Show and name the constraints in standard form (suitable forusing Excel) ( 1 mark)
d)Solve the problem by using Excel. Do so and write a conclusion of your findings for the person.(2 marks)
Total (4 marks)
Question 3 (5 marks):
ABC company has five (5) factories. They send their products through five (5) distribution centers (DCs) that serve a specific market. To use full truckloads and to have the economy of scale in transportation, they also use two cross-dock centers as transshipment points. All the required data are represented in the following tables.
Transportation cost($) Crossdock
| Factory | Crossdock 1 | Crossdock 2 |
| Factory 1 | 15 | 40 |
| Factory 2 新加坡数学代考 | 19 | 52 |
| Factory 3 | 29 | 16 |
| Factory 4 | 60 | 15 |
| Factory 5 | 50 | 63 |
| Transportation cost($) | Distribution Center | ||||
| Crossdock | DC1 | DC2 | DC3 | DC4 | DC5 |
| Crossdock 1 | 10 | 20 | 14 | 35 | 36 |
| Crossdock 2 | 40 | 10 | 13 | 17 | 70 |
Capacity of Factory (units) 新加坡数学代考
| Factory 1 | 150 |
| Factory 2 | 320 |
| Factory 3 新加坡数学代考 | 80 |
| Factory 4 | 170 |
| Factory 5 | 200 |
Demand for each DC (units)
| DC 1 | 140 |
| DC 2 | 130 |
| DC 3 新加坡数学代考 | 180 |
| DC 4 | 250 |
| DC 5 | 150 |
ABC wants to minimize the whole distribution costs. So, please answer the following questions:
(a)Developan optimization model to solve the above transshipment Please clearly define the decision variables, constraints that must be satisfied, and objective function with the corresponding explanations.( 2 mark)
b)How many units of products are transshipped to DCs through cross-docks 1 and2?( 1 mark) 新加坡数学代考
c)Which DC’s will be served by each cross-dockcenter?( 1 mark)
d)What is the minimum total distributioncost?(1 mark)
Total (5 marks)
Question 4(2 marks): 新加坡数学代考
The table below represents the travel time in minutes between 10 locations in Singapore. Draw the corresponding network and use the shortest route algorithm to find the shortest distance from node 1 to each node and the final location at node 10. Please note that all the branches are two ways which mean they are undirected.
| Start node | End node | Travel time( minutes) | |
| Branch 1 | 1 | 2 | 15 |
| Branch 2 | 1 | 3 | 14 |
| Branch 3 | 1 | 4 | 12 |
| Branch 4 | 1 | 5 | 17 |
| Branch 5 | 2 | 3 | 7 |
| Branch 6 | 2 | 7 | 19 |
| Branch 7 | 3 | 6 | 8 |
| Branch 8 | 3 | 4 | 11 |
| Branch 9 | 4 | 5 | 13新加坡数学代考 |
| Branch 10 | 4 | 6 | 8 |
| Branch 11 | 5 | 9 | 11 |
| Branch 12 | 6 | 7 | 12 |
| Branch 13 | 6 | 8 | 6 |
| Branch 14 | 6 | 9 | 15 |
| Branch 15 | 7 | 8 | 9 |
| Branch 16 | 7 | 10 | 15 新加坡数学代考 |
| Branch 17 | 8 | 9 | 12 |
| Branch 18 | 8 | 10 | 17 |
| Branch 19 | 9 | 10 | 15 |
Total (2 marks)
Question 5 (2 marks): 新加坡数学代考
The following table shows a network identified by its starting and ending nodes. The distances between nodes are in miles. Draw the network and use the minimal spanning tree to find the minimum distance required to connect them. Highlight (make bold) the final connections.
| Branch name | Start node | End node |
Distance(miles) |
| Branch 1 | 1 | 2 | 4 |
| Branch 2 | 1 | 3 | 5 新加坡数学代考 |
| Branch 3 | 1 | 4 | 3 |
| Branch 4 | 1 | 5 | 6 |
| Branch 5 | 2 | 3 | 5 |
| Branch 6 | 2 | 8 | 6 |
| Branch 7 | 3 | 6 | 6 |
| Branch 8 | 3 | 4 | 3 |
| Branch 9 | 3 | 7 | 5 |
| Branch 10 | 4 | 5 | 4 新加坡数学代考 |
| Branch 11 | 4 | 6 | 7 |
| Branch 12 | 5 | 6 | 5 |
| Branch 13 | 5 | 10 | 3 |
| Branch 14 | 6 | 7 | 4 |
| Branch 15 | 6 | 10 | 8 |
| Branch 16 | 7 | 8 | 4 |
| Branch 17 | 7 | 10 | 5 |
| Branch 18 | 7 | 11 | 9 新加坡数学代考 |
| Branch 19 | 8 | 9 | 2 |
| Branch 20 | 9 | 11 | 5 |
| Newrow 21 | 10 | 11 | 7 |
Total (2 marks)
Question 6 (2 marks):
In the following table, the branches represent the units of electrical power that can be delivered along with the various links between relay stations in 7 different locations. The numbers in the fourth column in the table represent the maximum associated flow capacities for each branch. By using the maximal flow algorithm, fill in the second table below by showing the routes, the flow along each route, and the total flow. Also, show the blocked routes and flows at the source and sink and the totals.
| Branch name Start node End node Capacity | |||
| Branch 1 | 1 | 2 | 6 |
| Branch 2 | 1 | 3 | 4 |
| Branch 3 | 1 新加坡数学代考 | 4 | 5 |
| Branch 4 | 2 | 3 | 4 |
| Branch 5 | 2 | 6 | 7 |
| Branch 6 | 3 | 4 | 5 |
| Branch 7 | 3 | 5 | 3 |
| Branch 8 | 3 | 6 | 3 |
| Branch 9 | 4 新加坡数学代考 | 5 | 3 |
| Branch 10 | 4 | 7 | 6 |
| Branch 11 | 5 | 7 | 4 |
| Branch 12 | 6 | 7 | 5 |
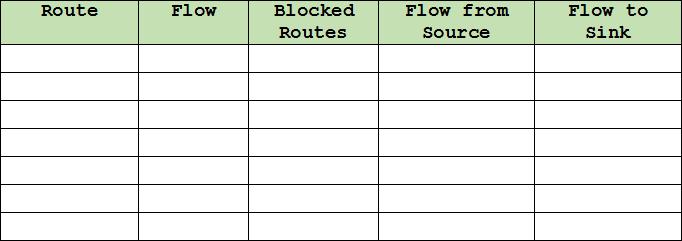
Total (2 marks)
Question 7 (5 marks):
The Main Roads Department is widening the area around two major roads to cater for increased traffic to a new hospital and activity center. This involves laying gas and water pipes and various other activities. For simplicity, these activities have not been named but are labeled A through L. Their immediate predecessors and time requirements are given in the following table.
| Activity | Optimistic time(a) | Most Likely time(m) | Pessimistic time(b) | Predecessors |
| A | 2 | 3 | 4 | |
| B | 3 | 6 | 9 | A |
| C | 2 | 5 | 7 | B |
| D | 6 | 12 | 27 | A, B |
| E | 4 | 6 | 13 | A, B, C |
| F | 11 | 17 | 29 | D, E |
| G | 6 | 12 | 16 | E, F |
| H | 3 | 6 | 10 | A, B, D, E |
| I | 2 | 5 | 7 | C, D, E, F |
| J | 4 | 7 | 13 | D, E, F, G |
| K | 3 | 6 | 12 | D, I, J |
| L | 4 | 7 | 15 | I, J, K |
a)Include columns for expected time (in months) and variance to the above table (include formulas in the table heading). Use two decimal places for expected time and variance.(1 mark) 新加坡数学代考
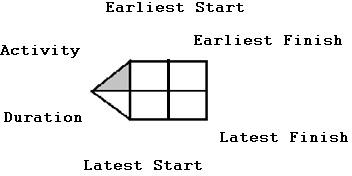
b)Draw a CPM/Pert diagram of the activities using the followingconvention:
(1 mark)
c)Calculate the expected project length, the variance of the critical path, the standard deviation of the critical path, and the activities along the critical path.(2 marks)
d)What is the probability the project will be completed within the due time of 80months?(1 mark)
Total (5 marks)

更多代写:计算机网课代修 新加坡统计Final exam代考 新西兰Essay修改 新加坡调查报告代写 新加坡paper代写 供应链管理essay代写
