IB2070 Mathematical Programming II
Assignment Instructions 数学编程课业代做
All assignments must be submitted ONLINE via my.wbs by 12pm (midday) UK time on the date displayed against this assessment.
Please ensure that you have inserted a completed assignment coversheet, which must be included as the first page of your script. This should include your Student ID number, but not your name.
Word Limit
1500 word limit.
Word Count Policy
WBS has a school-wide policy on word counts. This is strictly enforced to ensure consistency across modules and programme. You can find more information about this policy in your Student Handbook under Academic Practice – 7i. Word count policy.
This is a strict limit not a guideline: any piece submitted with more words than the limit will result in the excess not being marked.
Academic Practice 数学编程课业代做
Please ensure you read the full guidelines for Academic Practice in the Undergraduate Handbook and ensure you understand it. If in doubt, please seek clarification in advance of your submission.
This includes important information on:
- Cheating, plagiarism and collusion
- Correct referencing
- Using internet sources in assessments
- Academic writing
- English Language support
- Word count policy
When you submit this assignment online, you will be required to tick a declaration box indicating that the work involved is entirely your own. Each assignment will be put through plagiarism software to identify any collusion or inadequate referencing of materials used from different sources. Please do not submit images of your typed work unless you have been specifically requested to do so.
We would consider taking action if your work:
- is too reliant on the words of particular authors (rather than presenting your ideas in your own words), if the essay uses the ideas or words of an author without referencing them or putting their words into quotations (plagiarism).
- suggests that you have worked very closely with another student or students (unless explicitly asked to do so by your Module Leader/Tutor) (collusion).
- includes unreferenced work that you have previously submitted for any accredited course of study (unless explicitly asked to do so by your Module Leader/Tutor)(self-plagiarism).
The Use of Artificial Intelligence (AI) 数学编程课业代做
The University recognises an increasing number of technologies such as Artificial Intelligence and that they may be applicable in your completing this assessment. The assessment brief sets out specific requirements or restrictions, and your student handbook has further guidance and advice.
You are reminded that the inappropriate use of such a technology may constitute a breach of University policy, such as the Proofreading Policy or Regulation 11 (Academic Integrity). If you breach these policies, it may have significant consequences for your studies. Please make sure you read and understand the assessment brief and how AI may or may not be used.
If a generative AI or similar is permitted and has been used you MUST make clear why you used such a tool or service, what you used it for and you will be obliged to confirm that you take sole intellectual ownership of any submitted work. As appendices, and as part of your submitted work, you must provide screenshots of the question and the AI-generated response, alongside an explanation of how the content has been utilised. You should note the relevant reference alongside each screenshot.
When you submit you must complete (physically or electronically) a declaration. This requires you to explain the use of any AI. Failure to disclose at the point of submission may be prejudicial in any later investigations should they arise.
For this assessment the use of AI is:
– Prohibited
You MUST NOT use any generative Artificial Intelligence in this assessment unless specifically
authorised for reasonable adjustments. You MAY use non-generative tools such as a spell-check, basic grammar check (non-generative), calculator or similar. If you have any doubts about a tool or service you plan to use please contact the module leader.
Extensions and Self-certification 数学编程课业代做
Late submissions will incur a penalty of 5% for every 24 hour period after the due date and time, i.e. this begins one minute after the submission deadline (beginning at 12.01pm).
Requests for specific extensions (of up to 15 days) which are typically for longer and more serious concerns must be submitted via my.wbs ideally 72 hours BEFORE the deadline. Extensions can only be approved if you clearly detail your circumstances and provide supporting documentation (or a reason as to why you cannot provide the supporting documentation at the time) as set out in the Mitigating Circumstances Policy. 数学编程课业代做
Self-certification is a university-wide policy whereby you are permitted an automatic extension of 5 working days on eligible written assessed work without the need for evidence. WBS permits selfcertification for all types of written, assessed works such as essays and dissertations. It is not permitted for exams, course tests, or presentations.
You can self-certify twice within each year of study, starting from the anniversary of your course start date. This will cover all eligible written assessments that fall within the self-certification period, as long as they have not previously had an extension applied. To find out further details about the self-certification policy please see: https://my.wbs.ac.uk/-/academic/20778/item/id/1244460/.
If you wish to self-certify for an extension of 5 working days, please select ‘Self-certification’ in the Extension Type field. If you wish to request a longer extension than 5 working days, please leave the Extension Type as ‘Standard’.
Your assignment instructions begin below. 数学编程课业代做
- The assignment is for individual work. Any similarity between different submitted works will be investigated for plagiarism according to the University’s policy.
- Handwritten solutions will not be accepted. Please write your answers clearly using MS Word or LaTeX with font size 11 or 12 and then convert it to a PDF file. Make sure that each sheet has your University ID Number and the question number(s). The main body of your work should NOT exceed 4 pages.
- You can present your Excel Solver models (as screenshots) for reference in an appendix. The appendix will NOT be marked.
- Use Excel Solver whenever needed, but do not include your Excel spreadsheet(s) as an answer of any question. If necessary, your spreadsheet will be requested later for checking.
- Submit your work online (in PDF format) on or before Thursday, 5 December 2023 at 12:00 noon via my.wbs No other submission method will be accepted. Late submissions are automatically marked down.
- An online forum will be open for any requests for clarification on the assignment, but no request for clarification on the assignment will be accepted within 24 hours of the submission deadline.
Question 1 [70%] 数学编程课业代做
ABC Company provides a service to its clients. Each client has a job with a processing time of several days. Once the process starts for a client’s job, it must be carried out without any interruption. Each client’s job requires a certain number of operators in each day of its process. ABC must create a plan for the 4 available clients’ jobs for the next 5 days with minimum number of operators needed to satisfy this demand. The processing time of the client’s jobs and the number of operators each of them needs in each day is given below. For instance, client 1 has a job that lasts for 2 days, and 2 and 3 operators are needed for days 1 and 2 of its execution.
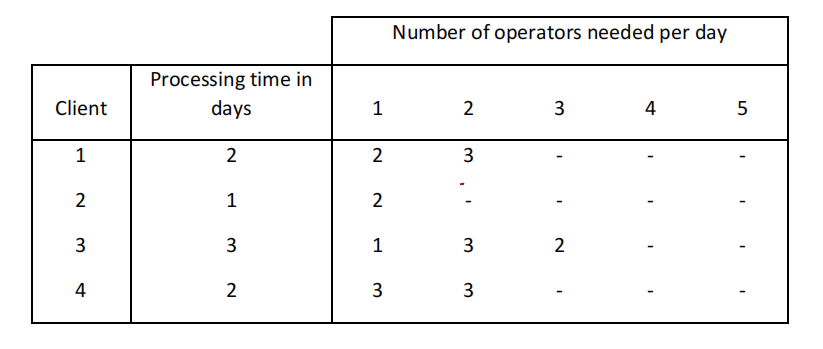
(a) Formulate an integer linear programming model to minimise the total operators needed. Describe decision variables, objective function, and constraints properly.
(b) Solve the ILP formulated in part (a) using Excel Solver. What is the optimal solution and total operators needed?
(c) Job 2 must start at least one day before job 3. For example, job 2 can start on day 1 and job 2 can start on days 2, 3, 4, or 5. Modify your model and add this condition.
(d) Solve the modified ILP formulated in part (c). Explain how the optimal solution is different from part (b).
(e) If job 1 is started on day 1 then job 4 also must be started on day 1. Modify your model to accommodate this condition.
Question 2 [30%] 数学编程课业代做
In the fashion retail industry transshipment is the flow of the products from a retail location to another one to satisfy the demand at the receiver location. Sender only sends a product if it has excess inventory to its demand and it occurs to rebalance the inventory among retail locations. Assume that there is a retailer with ? stores. There are O products nominated for transshipment. The available inventory rio, i = 1,2, … ,I, o = 1,2, … ,O, and demand levels dio, ? = 1,2, … ,I, o = 1,2, … , O, for all stores are known. Moreover, the sales prices of these products are known and are the same in all stores, po, o = 1,2, … O. There is a transportation cost when a product is sent from a store to another, cij, i,j = 1, 2, … I which is independent for the type of the product. Assume that if a product o is transshipped from store i, all its available inventory, i.e., rio, must be sent to a single store, thus, partial transshipment is not allowed. However, a store can receive the same product from different stores.
(a) Formulate a mixed integer linear programming model to maximise the total profit. Describe decision variables, objective function, and constraints properly.
(b) Now assume that all stores have a capacity on the number of products that they can transship. Modify your model to accommodate this extra condition.

更多代写:cs澳洲Final exam代考 多邻国助考 英国经济学网课代考 北美essay论文代写 北美Anthropology代写 从数据到决策的应用分析代写
