MTH 7241
Practice Problems for Test 1
数学测试代考 1).Four balls are chosen at random from a box which contains two Red balls and some number of Green balls. The probability that both Red balls are
1).
Four balls are chosen at random from a box which contains two Red balls and some number of Green balls. The probability that both Red balls are chosen is twice the probability that neither Red ball is chosen. How many Green balls are in the box?
2). 数学测试代考
A maze for rats is constructed with two doors; door 1 immediately leads to the exit, door 2 leads back to the maze after 1 minute. Assume that a rat is equally likely to choose either door at all times, and that if several rats are in the maze then they choose independently.
a). A rat is put in the maze. Find the expected time until it escapes.
b). Two rats are put in the maze. Find the expected time until the first escape occurs, and find the expected time until both escape.
c). Suppose n rats are put in the maze. Find the expected time until the first escape occurs. [Hint: you may want to condition on the first choices made by all the rats].
3). 数学测试代考
A biased coin has probability p of coming up Heads. The coin is tossed repeatedly.
Let N2 be the number of tosses until the first occurrence of the sequence (Heads, Tails). Use the conditional expectation method to compute E[N2]. [Hint: follow the methodology used in class, when we computed the expected number of tosses until the first occurrence of Heads. You will find it useful to first separately compute E[N2|H1] where H1 is the event that the first toss comes up Heads].
4).
Mary’s bowl of spaghetti contains n She selects two ends at random and joins them together. She does this until there are no ends left. What is the expected number of spaghetti hoops in the bowl?
5). 数学测试代考
3 balls are distributed in 3 boxes. At each step, one of the balls is selected at random, taken out of whichever box it is in, and moved at random to one of the other boxes. Let Xn be the number of balls in the first box, after n steps.
a). Find the transition matrix of the chain X0, X1, . . ..
b). Find the stationary distribution of the chain.
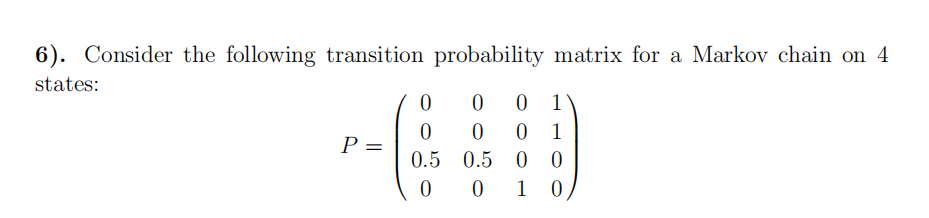
Number the states {1, 2, 3, 4} in the order presented.
a). Find and classify the equivalence classes of the states (irreducible and transient).
b). Find a stationary distribution for the chain.
7). 数学测试代考
Suppose that coin 1 has probability 0.7 of coming up Heads, and coin 2 has probability 0.4 of coming up Heads. If the coin tossed today comes up Heads, then we select coin 1 to toss tomorrow, and if it comes up Tails, then we select coin 2 to toss tomorrow. If the coin initially tossed is equally likely to be coin 1 or coin 2, then what is the probability that the coin tossed on the third day after the initial toss is coin 1?

