Math 3FF3 – Practice Exam
数学模拟代写 Math 3FF3 – Practice Exam Give full solutions to each of the following problems. Remember that you will be marked for the clarity and ···
Give full solutions to each of the following problems. Remember that you will be marked for the clarity and accuracy of your work, not just correctness. You will have plenty of time on the test, so take as much time as you need to make sure that your reasoning can be clearly understood by the reader.
1. 数学模拟代写
Suppose that h(t) is twice difffferentiable with h(0) = 0 and conisder the initial value problem
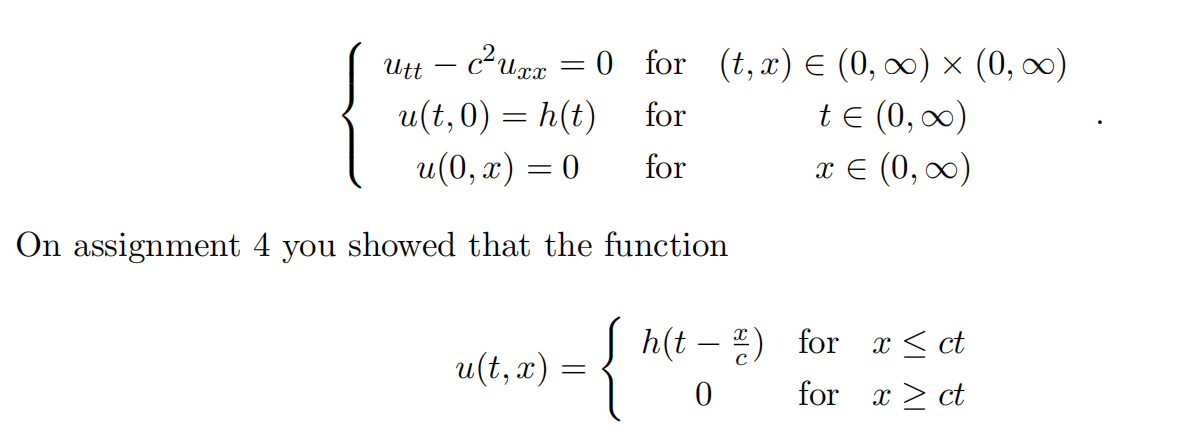
is a solution to the IVP given the assumption that h‘(0) = h”(0) = 0. Now show that this assumption is necessary, i.e. construct a function h(t) with h‘(0) = 0 or h”(0) = 0 such that u(t, x) is not a solution to the IVP.
2.
Solve the boundary value problem given by

where i is the complex unit and k ∈ R . Be sure to include enough explanation that the logic of your computation is clear.
3. 数学模拟代写
State the defifinitions of pointwise convergence, uniform convergence, and mean-square conver-gence.
(a)Explain (with justifification) the implications between these three defifinitions, i.e. which of these properties imply which others.
(b) For each implication in your answer to (a), give an example of a sequence of functions on the interval (0, 1) which satisfy one defifintion but not the other.
(c) Explain the signifificance of mean-square convergence with regard to our study of Fourier series.
4. 数学模拟代写
Write out the proof of uniqueness in Theorem 7 of the Lecture Notes that uses Green’s formula as described on page 83-84 of the Lecture Notes, but with much more detail than is given in the Lecture Notes. You are invited to include the argument from the Lecture Notes, but you will be marked on what you add to the proof.
5.
Solve the inhomogeneous initial value problem given by
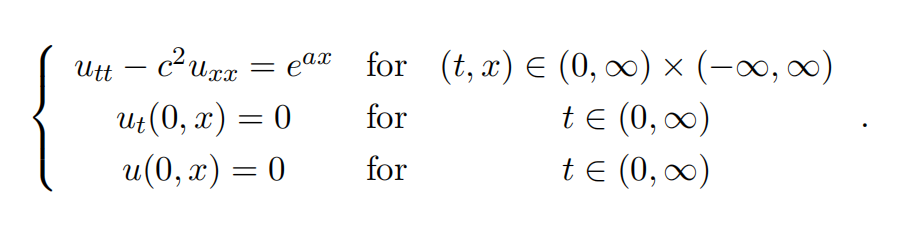
where a ∈ R. Be sure to include enough explanation that the logic of your computation is clear.
6. 数学模拟代写
Suppose that u(t, x) is a solution to the difffferential equation ut = kuxx for all t > 0, x ∈ R, where k is a fifixed real number.
(a)Prove that the translate u(t, x − x0) is also a solution for any x0 ∈ R.
(b)Prove that any derivative (e.g. ux, utt, etc.) of u is also a solution. You may assume that the derivatives exist and are continuous.

其他代写:program代写 project代写 java代写 matlab代写 finance代写 英国代写 北美作业代写 homework代写 assignment代写 加拿大代写 python代写 report代写 paper代写 essay作业代写 作业代写
