MT5863 Semigroup theory: Problem sheet 8
(0-)Simple semigroups, Rees (0-)matrix semigroups
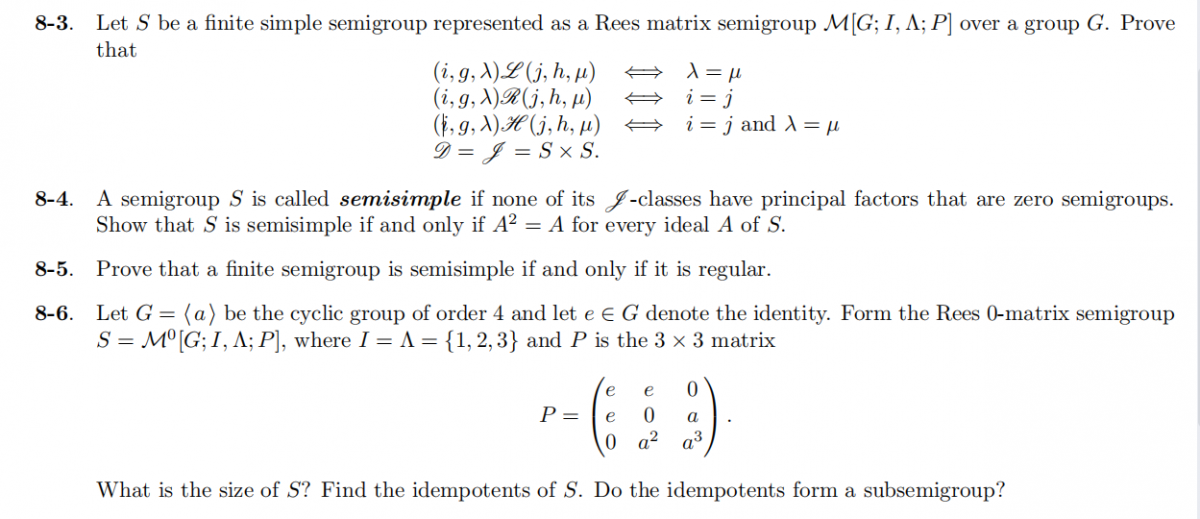
数学半群代做 Simple semigroups 8-1. Let S be a finite 0-simple semigroup. Prove thatabc = 0 ⇒ ab = 0 or bc = 0 for any a, b, c ∈ S. Is it true that ab = 0
Simple semigroups 数学半群代做
8-1. Let S be a finite 0-simple semigroup. Prove that
abc = 0 ⇒ ab = 0 or bc = 0
for any a, b, c ∈ S.
Is it true that ab = 0 implies that a = 0 or b = 0?
8-2. A sequence of (two sided) ideals S1 ⊃ S2 ⊃ · · · ⊃ Sm is called a principal series for a semigroup S if S1 = S, Sm is the minimum ideal of S and there is no ideal I such that Si ⊂ I ⊂ Si+1.
Show that the factors S1/S2, S2/S3, . . . Sm−1/Sm are isomorphic to the principal factors of the semigroup which correspond to J -classes which are not the minimal ideal of the semigroup. Deduce that any two principal series have isomorphic factors.
Rees matrix semigroups 数学半群代做
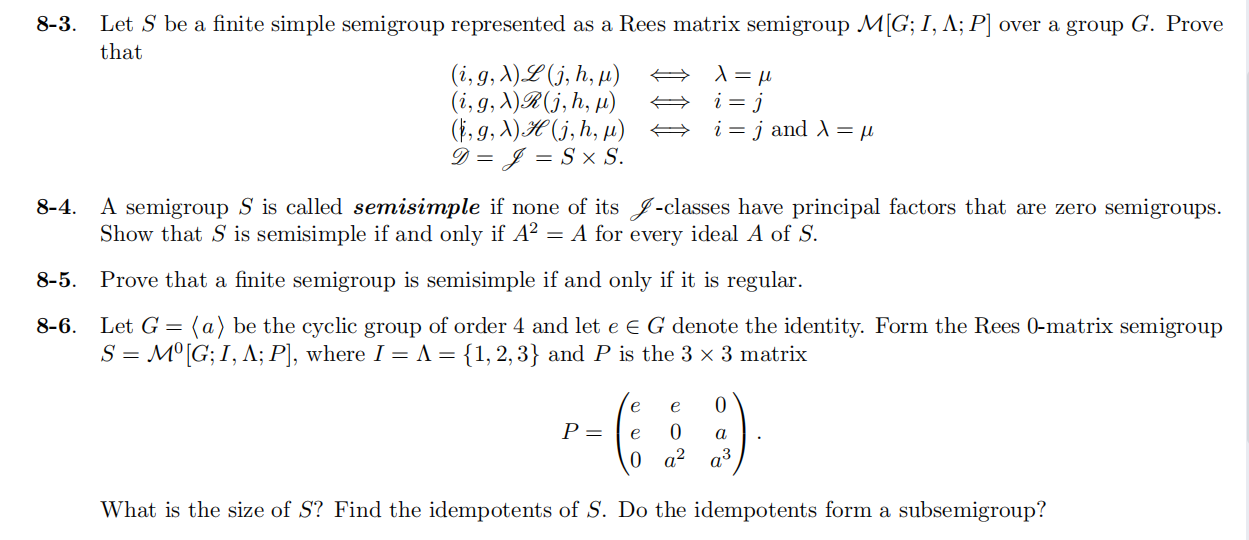
8-7. Consider the monoid S defined by the presentation
⟨a, b | a2 = 1, b3 = b, bab = b⟩.
Using the standard methods one may show that |S| = 10 and
S = {1, a, b, b2 , ba, b2a, ab, ab2 , aba, ab2a}.
Find the L – classes and R– classes of S. Prove that M = S\{1, a} is the minimal two-sided ideal of S. Find a Rees matrix semigroup isomorphic to M.
8-8. Let S be a Rees matrix semigroup M[T; I,Λ; P] over a semigroup T. Prove that an element (i, t, λ) ∈ S is regular if and only if there are j ∈ I and µ ∈ Λ such that the set pλjT pµi contains an inverse of t.
8-9. Prove that a Rees matrix semigroup S = M[T; I,Λ; P] over a semigroup T is simple if and only if T is simple.