Math 402-Su2020 Exam 2–Aug 20, 2021
抽象代数考试代写 (T or F) If R is a finite commutative ring with |R| = 25 and J is an ideal with |J| = 5, then thequotient ring R/J has |R/J| = 5.
Instructions.
This is an open book exam; you can use your notes and old homework as well.
Conventions: 抽象代数考试代写
- Thezero ring is neither a field nor an integral Every domain has at least two elements.
- Z= the ring of integers with the usual operations.
- Q= the ring of rational numbers with the usual operations.
- R= the ring of real numbers with the usual operations.
- C= the ring of complex numbers with the usual operations.
- Zn= {0, 1, . . . , n − 1}= the ring of integers mod n with the usual operations.
- Fp= Zp where p is an integer prime integer.
- If X is a finite set, |X| = the number of distinct elements inX.
PART 1: (22 points) In each part below, a statement is made. 抽象代数考试代写
On your answer sheet, you should circle ”T” if the statement is always true. Circle ”F” if the statement is not always true. No work required on this part.
- (Tor F) The polynomial ring C[x] is a fifield.
- (T orF) x4 + x + 1 is irreducible in Q[x].
- (Tor F) x4 + x + 1 is irreducible in R[x].
- (Tor F) x4 + x + 1 is irreducible in C[x].
- (Tor F) The polynomial ring Q[x] contains irreducible polynomials of degree n for every n ≥ 1.
- (Tor F) Suppose F is a field and and p(x) ∈ F[x] with deg(p) > If p(x) is irreducible in F[x], then p(x) has no roots in F. 抽象代数考试代写
- (T or F) Suppose F is a field and and p(x) ∈ F[x] with deg(p) > If p(x) has no roots in F, then p(x) is irreducible inF[x].
- (Tor F) The quotient rings F2[x]/(x4 + x + 1) and F2[x]/(x4 + x2 + 1) both have the same number of elements.
- (Tor F) The quotient ring F2[x]/(x4 + x + 1) is a fifield.
- (Tor F) The polynomial x7 + 4x3 + 2x2 + 8x − 2 has a rational root.

11.(Tor F) The polynomial x7 + 4x3 + 2x2 + 8x − 2 has a real root.
12.(T or F) Z is an ideal inZ[i].
13.(T or F) The principal ideal (5) is maximal inZ.
14.(T or F) The principal ideal (1 + i) is maximal inZ[i].
15.(Tor F) If f (x) is an irreducible polynomial in R[x], then deg(f ) ≤ 2.
16.(Tor F) The set J of non-units in Z10 is a maximal ideal in Z10.
17.(T or F) If R is a finite commutative ring with |R| = 25 and J is an ideal with |J| = 5, then thequotient ring R/J has |R/J| = 5. 抽象代数考试代写
18.(Tor F) The quotient rings Q[x]/(x − 1) and Q[x]/(x − 3) are isomorphic rings.
19.(T or F) The rings Z7and Z9 have the same number of units.
20.(Tor F) Z[x]/(x) is a fifield.
21.(Tor F) There is a ring isomorphism Q[x]/(x2 − 4) ∼= Q × Q.
22.(T or F) Assume R is a commutative ring with If φ : R ›→ Q is a surjective homo- morphism, then kernel of φ is a maximalideal.
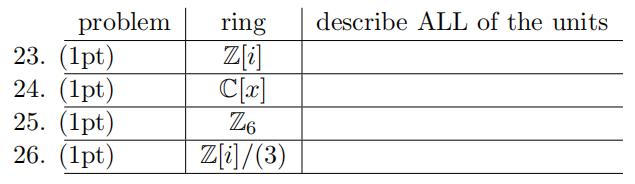
PART 2 (4 points) Fill in the table entry. Do not show work.
PART 3 (3 points) 抽象代数考试代写
Short answer, do not show work.
27.Factor x8− 1 into a product of irreducible polynomials inR[x].
PART 4 (4 points)
Short answer, do not show work. 抽象代数考试代写
28.Describe ALL maximal ideals in Z[i] containing the principal ideal (5) inZ[i].
29.Describe ALL maximal ideals in Z containing the principal ideal (12) in Z.
PART 5 (7 points)
Work required.
30.ProveZ[x]/(x2 + 1) is isomorphic to Z[i]. 抽象代数考试代写
31.Let (α) be the principal ideal generated by a non-zero Gaussian integer α. Prove that thequotient ring Z[i]/(α) is a finite (Note: In lecture, I stated this ring has exactly N (α) elements. You may NOT use that fact. Give a direct proof of finiteness.)

