ECON 701 Microeconomic Theory
FINAL ASSESSMENT
微观经济理论作业代写 NOTE: There are FOUR questions. Answer ALL FOUR questions. Each question is worth 25 marks. Please allocate your time appropriately. Good luck.
(Designed to be completed in: THREE hours)
NOTE: There are FOUR questions. Answer ALL FOUR questions. Each question is worth 25 marks. Please allocate your time appropriately. Good luck.
- This Final Assessment has been designed so that a well-prepared student could complete it within 3 hours.
- If any corrections are made during the 24 hours, you will be notified by a Canvas Announcement. Please ensure yournotifications are turned on during this period.
Submission Instructions
You answer should be submitted on CANVAS in the form af a single pdf file.
Please ensure that your answer clearly labels on each page the Question number that you are answering on that page. Please start each Question on a new page.
Question 1. (25 marks) 微观经济理论作业代写
This question deals with the theory of the consumer.
(1) What properties does an indirect utility function derived from the maximisation of a direct utility function subject to a standard (Walrasian) budget constraint necessarily satisfy? (5 marks)
(2) Consider a consumer with wealth w who consumes only two goods, good 1 and good 2 with corresponding prices p1 and p2. Suppose that this consumer has an indirect utility function
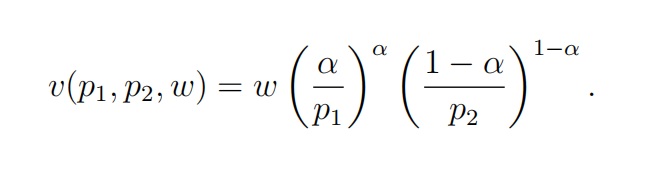
Does this indirect utility function satisfy the homogeneity and monotonicity properties that you stated in part (1)? [Notice that you are not asked about all of the properties you stated in part (1), just the homogeneity and monotonicity properties.] (5 marks)
(3) Find this consumer’s Marshallian and Hicksian demand functions, carefully explaining how you do so and what results from the theory of the consumer you are using. (15 marks)
Question 2. (25 marks) 微观经济理论作业代写
Consider an economy with two consumers (Consumer 1 and Consumer 2) and two goods (good 1 and good 2).
Suppose that the consumers’ preferences are given by the Cobb-Douglas utility function
u1 (x11, x21) = x11x21
and the Leontieff utility function
u2 (x12, x22) = min {x12, x22}

(1) What are Consumer 1’s Walrasian demand functions for each good, that is her demand for each good as a function of the prices, given her initial endowment? (2 marks)
(2) What are Consumer 2’s Walrasian demand functions for each good? (2 marks)]
(3) Find the competitive equilibrium. (4 marks)
(4) Find the contract curve. (4 marks)
(5) Draw the Edgeworth Box. Carefully mark the initial endowment, the equilibrium allocation, the equilibrium price vector, and the contract curve. Be as neat and accurate as you can. Please use a ruler to draw straight lines and to measure distances. (8 marks)
(6) Is the allocation ((x11, x21),(x12, x22)) = ((1, 1),(3, 3)) Pareto optimal? If it is, can it be supported as a competitive equilibrium by a reallocation of initial endowments to an allocation that gives Player 1 an initial bundle in which she receives no good 1? If so, what is her reallocated initial endowment? If not, explain why it is not possible. (5 marks)
Question 3. (25 marks) 微观经济理论作业代写
This question deals with the equilibria of two person games where each player has two or three strategies.
(1) Consider the following two person game.
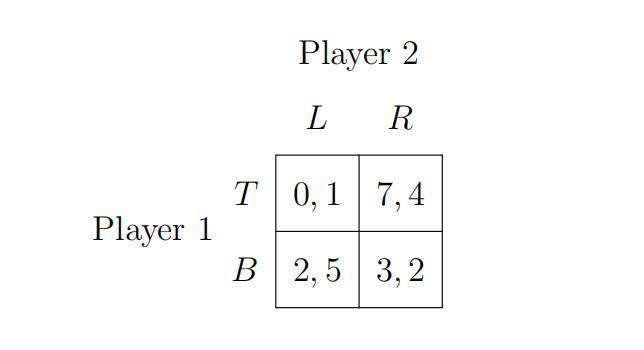
Graph the best reply correspondences for each player and mark on the graph all the mixed strategy equilibria, including the ones that are equivalent to pure strategy equilibria. Label your diagram completely, labelling the axes, any relevant values on each axis, and clearly indicating which is the graph of the best reply correspondence of Player 1 and which of Player 2. (12 marks)
(2) Consider a two player game that has best replies as indicated in the following diagram..
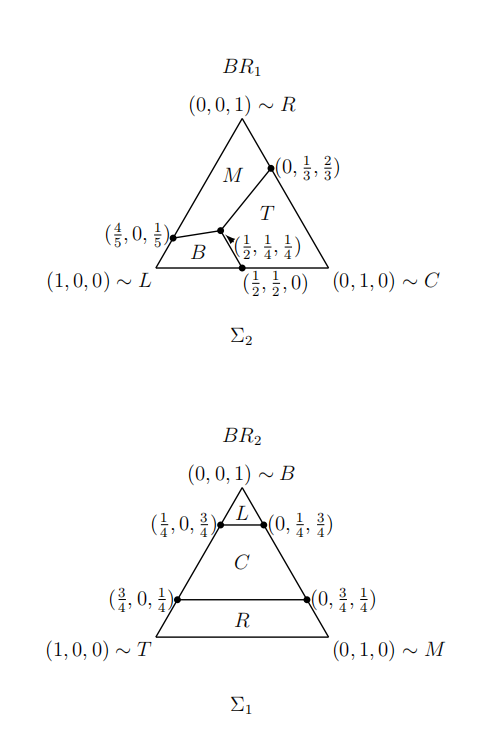
Use the best reply diagrams to find all mixed strategy equilibria. Carefully explain the process of how you found the equilibria and explain why the process does find all equilibria. You are not required to copy the diagrams into your answer unless you add some construction or labelling to them and refer to that
addition in your answer. (You should not need to do so.) (13 marks)
Question 4. (25 marks) 微观经济理论作业代写
Consider the following two-person extensive form game.
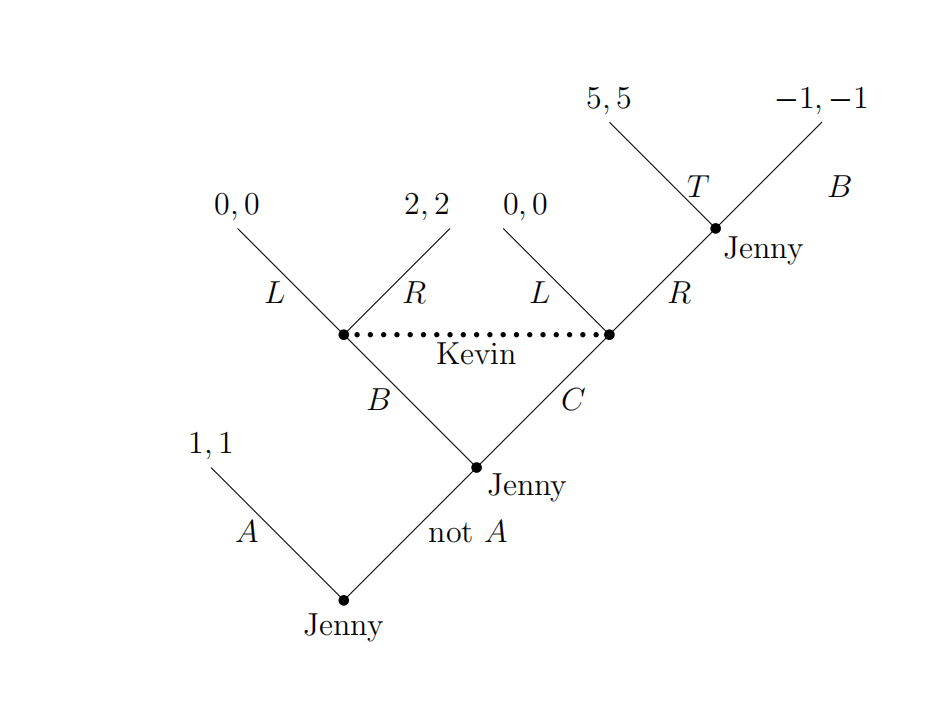
(1) Does the game have perfect recall? (2 marks)
(2) Apart from the game itself how many subgames does this game have? What are they? (2 marks)
(3) How many pure strategies does Jenny have? How many that are not equivalent to each other in the sense of Kuhn? (4 marks)
(4) Give the normal form of this game. Just use one copy of the strategies that are equivalent to each other. (4 marks)
(5) What are the pure strategy equilibria of this game? (4 marks)
(6) Which of the pure strategy equilibria are equivalent to behaviour strategy profiles that are subgame perfect equilibria? (4 marks)
(7) Find one sequential equilibrium and completely describe it, that is give both the behaviour strategies of each player and the beliefs at each nonsingleton information set, that is, at each information set containing more than one node.
[While strictly the definition requires also a specification of beliefs at singleton information sets these are trivial and you may follow standard convention in not considering them.]. (5 marks)
更多代写:cs美国作弊听证会 pte替考 英国exam代考机构 Chicago商科代写 essay润色修改 经济博弈论代写
