MATH5806 Applied Regression Analysis
Assignment 1
应用回归分析代写 Note: This assignment is due Friday 17th June, 12pm (noon) and mustbe uploaded to Moodle. Please submit name your file in PDF format using the
Note: 应用回归分析代写
- This assignment is due Friday 17th June, 12pm (noon) and mustbe uploaded to Moodle.
- Please submit name your file in PDF format using the following name A1-z1234567-FirstName-Surname.pdf.
- Assignments without signed plagiarism declaration (below) will not be accepted and late assignments will not be accepted unless accompanied by medical certificates.
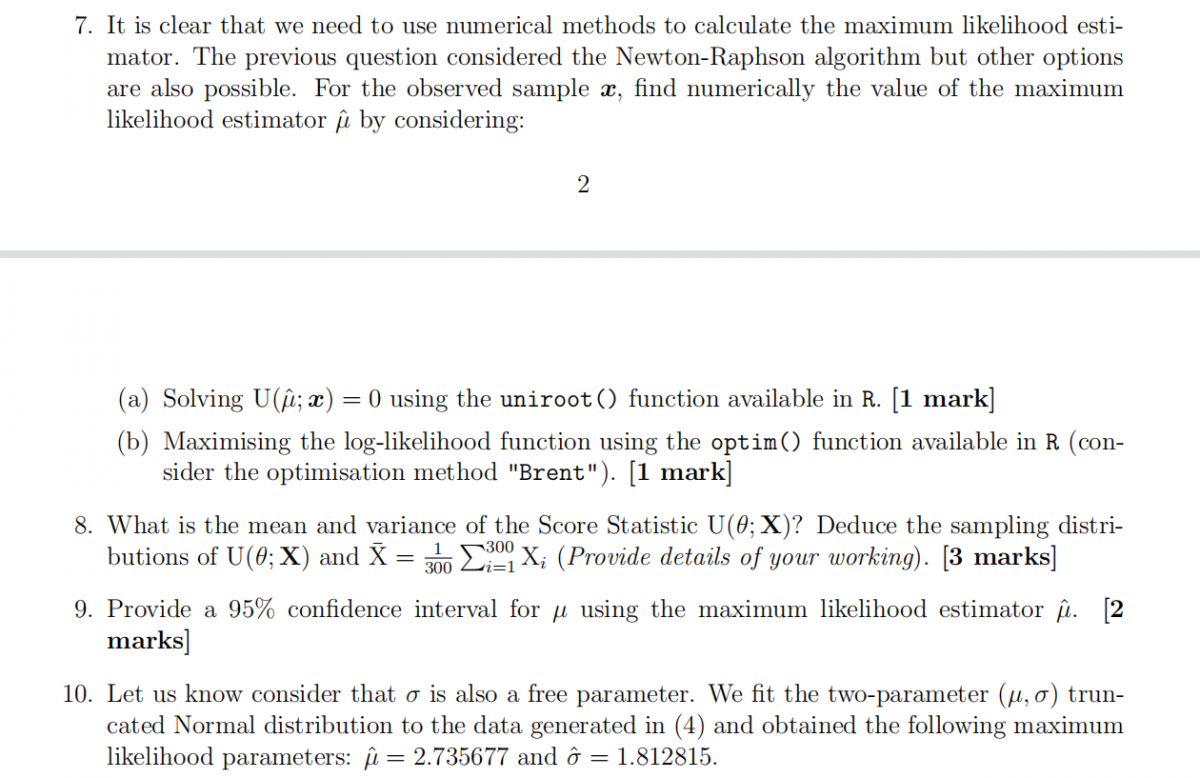
- This assignment weights for 15% of the final mark.
- There is a total of 1 exercise and 15 marks.
I declare that this assessment item is my own work, except where acknowledged, and has not been submitted for academic credit elsewhere. I acknowledge that the assessor of this item may, for the purpose of assessing this item reproduce this assessment item and provide a copy to another member of the University; and/or communicate a copy of this assessment item to a plagiarism checking service (which may then retain a copy of the assessment item on its database for the purpose of future plagiarism checking).
I certify that I have read and understood the University Rules in respect of Student Academic Misconduct.
Name Student No Signature Date
Exercise 1 [15 marks] 应用回归分析代写
The truncated Normal distribution corresponds to a restriction of the Normal distribution to some subset [a, b]; a, b ∈ R, a < b of the real line (R). We consider X to be a random variable following the truncated Normal distribution with location µ ∈ R and scale σ > 0 if it has probability density
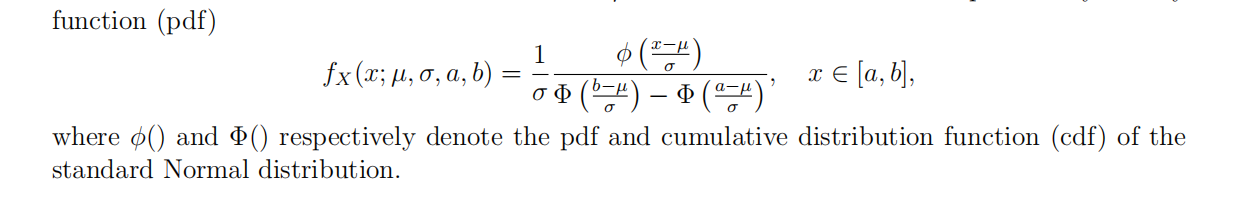
In this exercise we will consider a truncation on [1,∞) (i.e., a = 1 and b = ∞) and set σ = 2 to focus on a one parameter distribution.
1.
Calculate the expected value of X for general µ and then evaluate it at µ = 2.5 (Provide details of your working). [1 mark]
2.
What is the probability that X take a value less than 3 for general µ and for µ = 2.5? (Provide details of your working) [1 mark]
3.
Show that this one parameter distribution belongs to the exponential family of distribution.Specify whether it is of canonical form and if so, what the natural parameter is. (Provide details of your working) [1 mark]
4.
Using the R package extraDistr we generate a sample x of 300 observations from the truncated normal distribution on [1,∞) with µ = 2.5 and σ = 2 using the following lines of code:
1 # Install the package if needed 2 # install . packages (" extraDistr ") 3 library ( extraDistr ) 4 5 mu <- 2.5 6 set. seed (2022) 7 n <- 300 8 data <- rtnorm ( n =n , mean = mu , sd =2 , a =1)
For the observed sample x (object data in the above code), write down the log-likelihood function and evaluate it at the true parameter value θ = 2.5 (Provide details of your working). [1 mark]
5.
For the observed sample x, write down the expression for the score statistic U(µ; x) and evaluate it at the true parameter value µ = 2.5 (Provide details of your working). [1 mark]
6.
Implement the Newton-Raphson algorithm to find an estimate of µ such that the score function is equal to zero. Considering the starting value µ0= 0, print out the value of µ(m)for m = 1, . . . , 6. [1 mark]
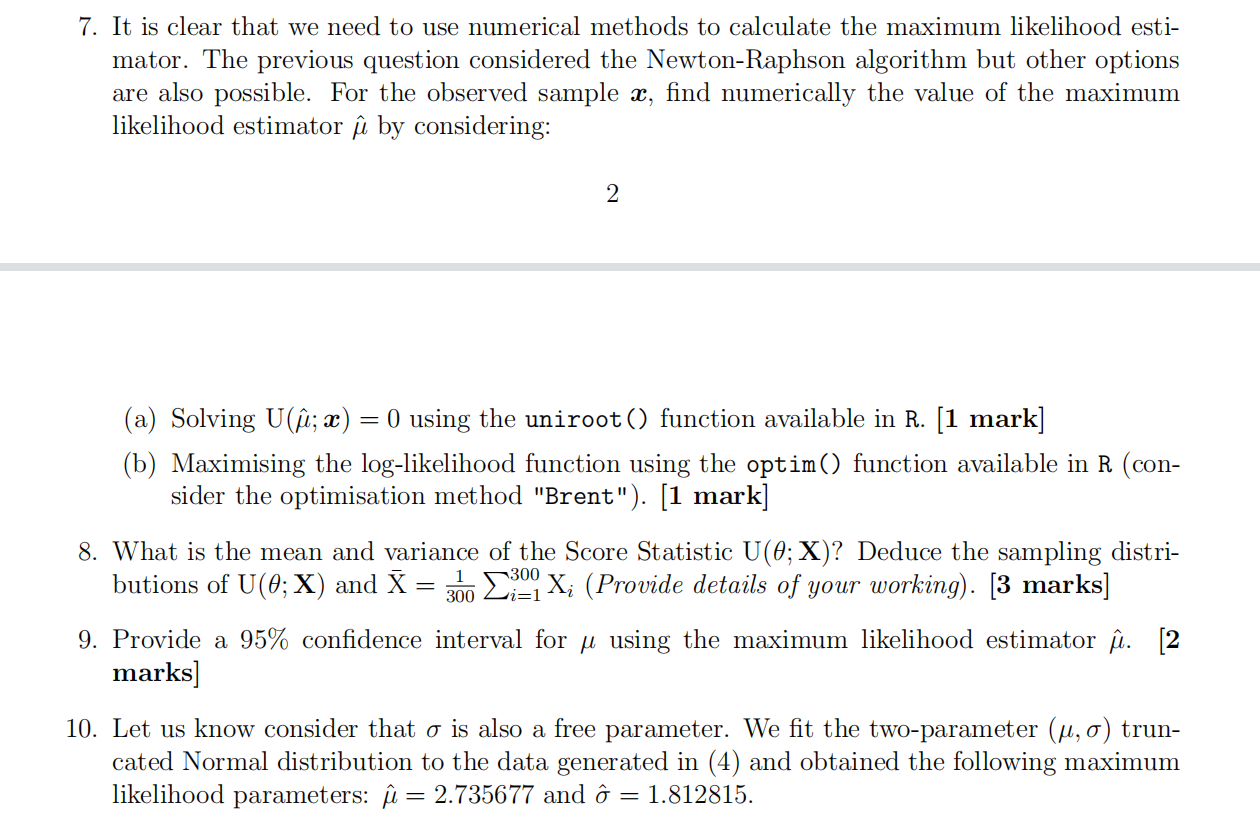
Use the deviance to test whether we should reject the null hypothesis H0 : σ = 2 for a level of significance α = 0.01. What is the observed value of the test statistic and what is the value of the critical value? What conclusion can you draw? [2 marks]

更多代写:Matlab代写推荐 代考價錢 英国Psychology代考 paper写作技巧 Outline代写 简历范文英文代写