半群作业代写 MT5863代写 半群理论代写

MT5863 Semigroup theory: Problem sheet 6

半群作业代写 Cancellative semigroups Recall that a semigroup S is cancellative if ax = bx and yc = yd implies that a = b and c = d for all a, b, c, d, x, y ∈ S.

Cancellative semigroups, regular semigroups

 

Cancellative semigroups

Recall that a semigroup S is cancellative if ax = bx and yc = yd implies that a = b and c = d for all a, b, c, d, x, y ∈ S.

6-1.

Let S be a cancellative semigroup. Prove that the semigroup S¹ (S with an identity adjoined) is cancellative if and only if S has no identity element.

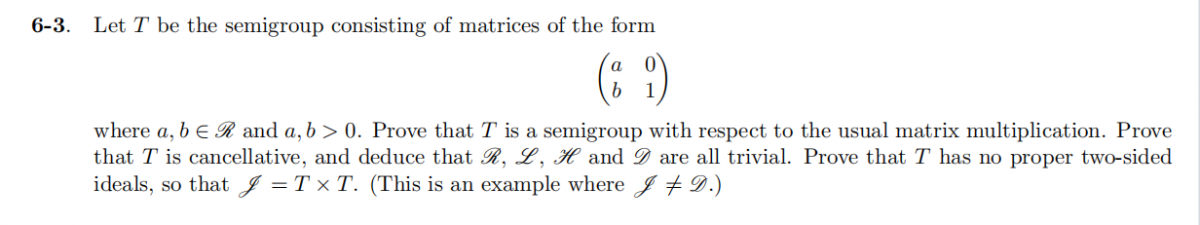
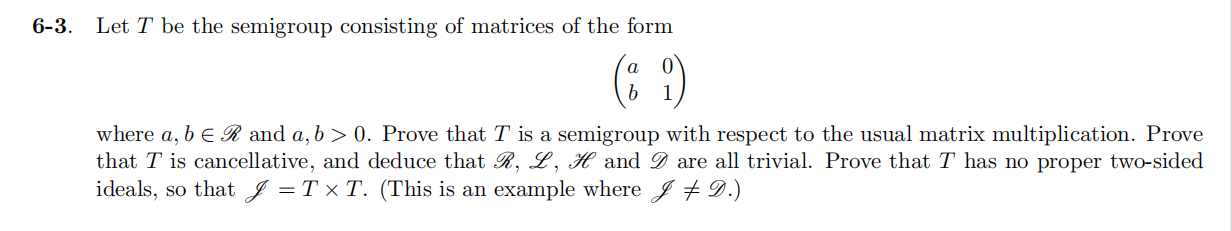
6-2. Let S be a cancellative semigroup without identity. Prove that in S we have R = L = H = D = ∆S.

 

 

Regular semigroups   半群作业代写

6-4.

Let S be a regular semigroup and let ρ be a congruence on S. Assume that a ∈ S is such that a/ρ is an idempotent in S/ρ. If x is any inverse of a² , then prove that e = axa is an idempotent, and that it belongs to a/ρ. (This result is known as Lallement’s Lemma.)

6-5.

Prove that the bicyclic monoid is regular. Prove that every rectangular band is regular.

 

 

6-7.

Let a and b be regular elements of a semigroup S. Prove that

(a) aL b if and only if there are inverses a ′ , b′ ∈ S of a and b, respectively, such that a ′a = b ′ b;

(b) aRb if and only if there are inverses a ′ and b ′ of a and b, respectively, such that aa′ = bb′ ;

(c) aH b if and only if there are inverses a ′ and b ′ of a and b, respectively, such that a ′a = b ′ b and aa′ = bb′ .

6-8.*   半群作业代写

Let S be a regular semigroup, and let E be the set of idempotents of S. For a set X ⊆ S and n ≥ 1 let Xⁿ = {x₁x₂ . . . x_n : x₁, . . . , x_n ∈ X}. For an element x ∈ S denote by V (x) the set of all inverses of x. Finally, for X ⊆ S let V (X) = S_x∈X V (x).

(a) For x = e₁e₂ . . . e_n ∈ Eⁿ and y ∈ V (x) define

f_j = e_j . . . e_nye₁ . . . e_j−1 j = 1, . . . , n.

Prove that all f_j are idempotents and that yxf_nf_n−1 . . . f₂xy = y.

(b) For x = e₁e₂ . . . e_n+1 ∈ Eⁿ⁺¹ and any y ∈ V (x) let

g_j = e_j+1 . . . e_n+1ye₁ . . . e_j    j = 1, . . . , n + 1.

Prove that all g_j are idempotents and that x ∈ V (g_ng_n−1 . . . g₁).

(c) Prove that V (Eⁿ) = Eⁿ⁺¹ for all n ≥ 1.

 

Further problems   半群作业代写

6-9. Let S be a regular semigroup. Show that the following are equivalent:

(a) S has exactly one idempotent;

(b) S is cancellative;

(c) S is a group.

6-10. Give an example of a semigroup S where Green’s R-, L -, H -, and D-relations equal ∆S, but where S is not cancellative, and S has no identity.

 

 

更多代写：cs马来西亚代写  代考雅思  英国final exam 代考   essay论文写作  代做功課犯法  半群理论课业代写

合作平台：essay代写 论文代写 写手招聘 英国留学生代写