Math 402-Wi2021
Exam 1
July 14, 2021
NAME
北美Math数学代写 In each part below, a statement is made. On your answer sheet, you should circle ”T” if the statement is always true.
Instructions. 北美Math数学代写
This is an open book exam; you can use your notes and old homework as well.
SCORE
1= /9
2= /19
3= /12
TOTAL = /40
Conventions: 北美Math数学代写
- Thezero ring is neither a field nor an integral Every domain has at least two elements.
- Z= the ring of integers with the usual operations.
- Q= the ring of rational numbers with the usual operations.
- R= the ring of real numbers with the usual operations.
- C= the ring of complex numbers with the usual operations.
- Zn= {0, 1, . . . , n − 1}= the ring of integers mod n with the usual operations.
- Zn[θ] = {a + bθ|a, b ∈ Zn} where θ is a symbol satisfying the rule θ2= −1.
- Z[i] = {a + bi : a, b ∈ Z} is the ring of Gaussian integers.
- Z[√2] = {a + b√2 : a, b ∈ Z} with operations inherited as a subset of R.
In each part below, a statement is made. On your answer sheet, you should circle ”T” if the statement is always true. Circle ”F” if the statement is not always true. No work required on this part.
1.(T or F) Let R be the set of integers Z. Define + as usual.However, define multiplication >by the rule: a > b = 0, for all a, b ∈ R. Then R is a ring.
- (T or F) Zis a subring of the Gaussian integers Z[i].
- (T or F) The product ring C × C is a fifield.
- (Tor F) The set of all non-units in Z9 is a subring of Z9.
- (T or F) The ring Z16contains eight units. 北美Math数学代写
- (T or F) Recall the ring M2(C) of 2 × 2 complex matrices. The subset of all units in M2(C) is a subring ofM2(C).
- (Tor F) The function φ : Z[√2] ›→ Z[√2]; φ(a + b√2) = a − b√2 is a ring isomorphism.
8.(Tor F) The element 1 + θ is a zero divisor in the ring Z2[θ]. 北美Math数学代写
- (T or F) The rings Z3× Z10 and Z2 × Z15 are isomorphic.
Note: Explanation required in 15 and 16; no explanation required for 10,11,12,13,14. matrix 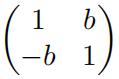 is a unit in M2(Z5). 北美Math数学代写
is a unit in M2(Z5). 北美Math数学代写
- (2)Among the following four functions, list ALL the ring homomorphisms:
(a) F : Z3 → Z3, F (x) = x3.
(b) tt : Z4 → Z4, tt(x) = 0.
(c) H : Z5 → Z5, H(x) = x4.
(d) K : Z6 → Z6, K(x) = 1.
- (2) Give an example of an infinite field that is NOT isomorphic to any of the fields in thelist {Q, R, C} :
- (2) Give an example of a finite field which does not have a prime number ofelements:
14.(1) What is your favoritering? 北美Math数学代写
- (4) This question focuses on the ring Z[√2] = {a + b√2 : a, b ∈ Z} with operations inherited as a subset ofR.
(a)LetE = {even integers}. Is the set Y = {a + b√2|a, b ∈ E} a subring of Z[√2]?
(b)Isthe set W = {a + a√2|a, ∈ Z} a subring of Z[√2]?
- (6) Define R = {a |a, b ∈ Z, b = an odd integer}. Note: R ⊂ Q.
(a)Show R is a subring ofQ.
(b)Show that R has an infinite number of
(c)Is the set X of non-units in R a subring of R?
Note: On the last two problems, saying ”we proved this in class” or ”we proved this in home- work” is not an acceptable justification. Be precise and complete.
- (6) Show COMPLETE work to compute the multiplicative inverse of 13 in Z48. Your finalanswer must be a least residue mod 48 and no credit for guessing.
- (6) Prove: If p is a prime integer, then the only subrings of Zpare {0} and Zp.

更多代写:汇编作业代写 北美exam网课代考 北美网课代写essay润色 北美文科网课essay代写 北美毕业论文代写 澳大利亚数学Midterm代考
