Applied PDE – Homework Assignment 7:
偏微分方程课业代写 Read, from Chapter 5.4 of Strauss – The Subsection entitled ”Three Notions of Convergence”; The Subsection entitled ”Convergence Theorems”,
Read, from Chapter 5.4 of Strauss – The Subsection entitled ”Three Notions of Convergence”; The Subsection entitled ”Convergence Theorems”, up to but not including Example 3;
Read, from Chapter 5.5 of Strauss – The first two paragraphs of the Subsection entitled ”The Gibbs Phenomena”;
Read, from Chapter 5.6 of Strauss – The first two pages, up to eq(10).
Homework: Chapter 5.4, problems 2, 8;
Problem: 偏微分方程课业代写
Consider the ”Initial Boundary Value Problem” (IBVP) for the heat equation:
ut =νuxx,t >0,0< x < π,ν >0,
u(x,t= 0) =x2,
u(x= 0,t) = 0,
u(x=π,t) = 2.
i) Represent the solution as a Fourier Sine Series; ii) Compute the first two terms of the series explicitly; iii) What is the steady state or equilibrium solution?
Problem:
Consider the ”Initial Boundary Value Problem” (IBVP) for the heat equation:
ut =νuxx,t >0,0< x < π,ν >0,
u(x,t= 0) =x2,
ux(x= 0,t) = 0,
ux(x=π,t) = 2.
i) Represent the solution as a Fourier Cosine Series; ii) Compute the first two terms of the series explicitly; iii) What is the steady state or equilibrium solution?
Problem: 偏微分方程课业代写
Consider the (Neumann IBVP) for the inhomogeneous heat equation:
ut −νuxx =f(x,t),t >0,0< x < π,
u(x,t= 0) =uin(x),
ux(x= 0,t) =ux(x=π,t) = 0.
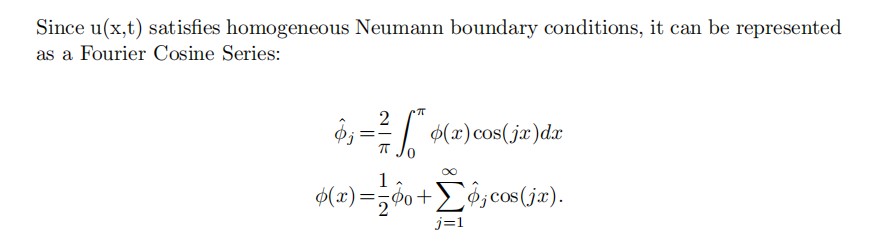
a (15 points): Construct the cosine series representation of the solution u(x,t) in three steps – i) Map the equation and data into Fourier space; ii) solve the initial value problem in Fourier space; iii) map the solution back to x-space. You can leave your answer in terms of integrals.
b (5 points): Consider the specific data
uin(x) =0
f(x,t) =δ(x−1)exp(+t),
where δ(x−1) is the Dirac delta function. For this specific data, do all of the integrals analytically.

更多代写:cgi代写 雅思作弊 英国bio生物代考 bio essay代写 Accounting论文代写 英文PPT代做
