Operations Management:Midterm #1
Show all work and calculations
代考运营管理 A small Starbucks in middle-America serves, on average, 60 customers a night.A typical night in this town is long, about 10 hours.
1)(17 points) 代考运营管理
A small four-step process runs with four employees, each one operating one step of the process. The cycle times are shown in the following table.
| Steps | Employe | Cycletime (seconds/customer) |
| 1 | Bob | 10 |
| 2 | Jim | 15 |
| 3 | Mary | 8 |
| 4 | Liz | 12 |
a)(4points)Draw the process flow diagram
b)(4points)Calculate the capacity of each stepin the process using unit so fcustomers perminute.
c)(2point)Which step is the botleneck? 代考运营管理
d)(2point)What is the capacity of the entireprocess?
e) (5 points) One day Jim called in sick. Mary agreed to do both his step and hers, but she can only do one step at a time. She is just as fast as Jim, and can also do Step 2 in 15 seconds per customer.
Does the capacity of the whole process change on this day? If yes, calculate the new capacity. If no, briefly explain why not. (Must decide on yes or no, not an argument for both.)
2)(10 points)
A small Starbucks in middle-America serves, on average, 60 customers a night.A typical night in this town is long, about 10 hours. There’s not much else to do. The manager observed customers in the store all night and she is wondering if she is allowing them to stay too long, just using the free internet.
She has gathered data on how many customers are in the store at any one time, and believes this average to be 18 customers. These customers are eating food they purchased and drinking coffee, but she has calculated that each customer could finish eating and drinking in 30 minutes. How much extra time (more than they would need to eat and drink) is the average customer spending in the store?

3)(10 points) 代考运营管理
Don’s Donuts makes donuts using a three step process as below. Each dozen of donuts costs $5 and is priced at $10, giving the company a margin of $5/dz. Don’s Donuts is doing a great business, working to full capacity, and selling all the donuts (of any kind) that they can make.
| Step1 | Step2 | Step3 | |
| Numberofworkers | 1 | 1 | 1 |
| Processingtimefor
Don’sDonuts |
5mins/doz | 15mins/doz | 1min/doz |
The company’s founder creates a new recipe, called The Special, that uses higher quality raw material costing more money, but can be made faster. The processing times for each dozen of donuts of the new type are as follows. The cost per dozen of these donuts is $8, and selling price is $12, giving a margin of $4/dz.
| Step1 | Step2 | Step3 | |
| Processingtimefor
Don’sSpecial |
5mins/doz | 10mins/doz | 4mins/doz |
Eventually, they want to make and sell only one type of donut. The company thinks they can make more money per hour making and selling only The Specials, as compared to only the first type. 代考运营管理
a)(6 points) Are they correct? Explain briefly.
Support your answer by showing all work and calculations.
b)(4 points) How much more profit will they make per hour if they follow their strategy to make only the more profitable type? Show all work.
4)(10points)True or False,2 point seach. 代考运营管理
For the following questions, no partial credit will be allowed. Write all answers (the word True or False) on the answer line.
1.a) Truck deliveries charged to a retail store by the supplier would be considered, by the store, to be a carrying cost.
In the EOQ model, stockouts are omitted because carrying and ordering costs are much larger.
b) One basic difference between the EOQ and ELS models is using ordering versus set-up costs.
c) The basic underlying assumptions of the EOQ model would be violated if the product in question had a high seasonal demand. (high sales in the summer and low sales in the winter)
d) Shortening lead-times is effective because it can reduce the re-order inventory levels.
5) (12 points) 代考运营管理
A retailer upgrades its warehousing facility, resulting in a decrease in inventory carrying cost from 40% to 25% of the cost of the items stored. What effect does this have on the following performance parameters for their EOQ model? Write the correct answer (decreases, remains the same, or increases) for each parameter in parts (a), (b) and (c).
Performance parameter
(a) Economic Order Quantity
(b) Ordering frequency
(c) Average monthly ordering cost
6) (15 points)
CK Electronics uses the EOQ model to order aluminum frames from its supplier. CK requires 5000 frames per year for the manufacturing process. The carrying cost is calculated by multiplying their cost of capital (40%) times the purchase cost per unit of the frames. Their ordering cost is $200 per order. The frame supplier has suggested the following price schedule to CK:

(e.g. if order quantity were 1100 frames, each frame would cost $45)
Determine the optimal order quantity and the corresponding total inventory costs per year. (Assume stock-out costs are zero.)
7.(16 points) 代考运营管理
Given the feasible region of some LP problem, with corner points A, B, C and D as shown: X1 and X2 are the number of units of product produced; S1 and S2 are slack variables. The constraints of the problem are: 2×1+4×2 <=48 and 4×1+2×2 <= 60
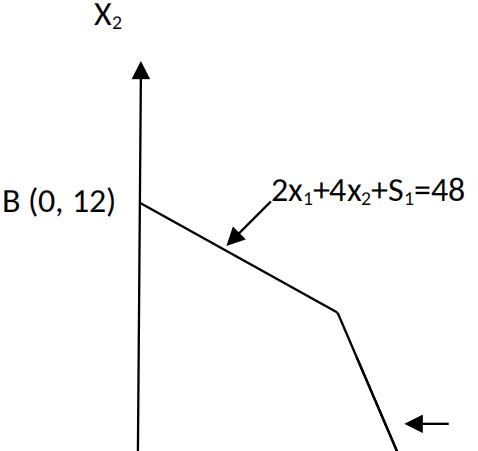
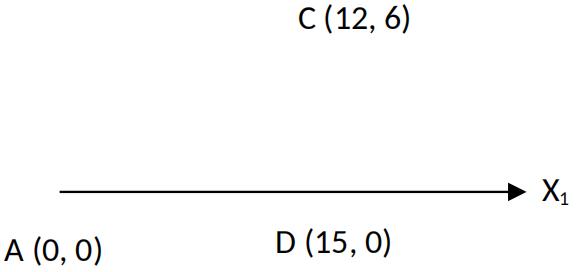
a) (4points) What is the solution at corner B (what are the values of all variables)?
c) (4 points) If the objective function were: Profit = $8×1 + $6×2 , what would be the maximum profit for the problem?
c)(4points)Iftheobjectivefunctionwere:Profit=$8×1+$6×2,whatwouldbethe maximum profitfortheproblem?
d) (4 points) What variables are equal to zero at corner point D?
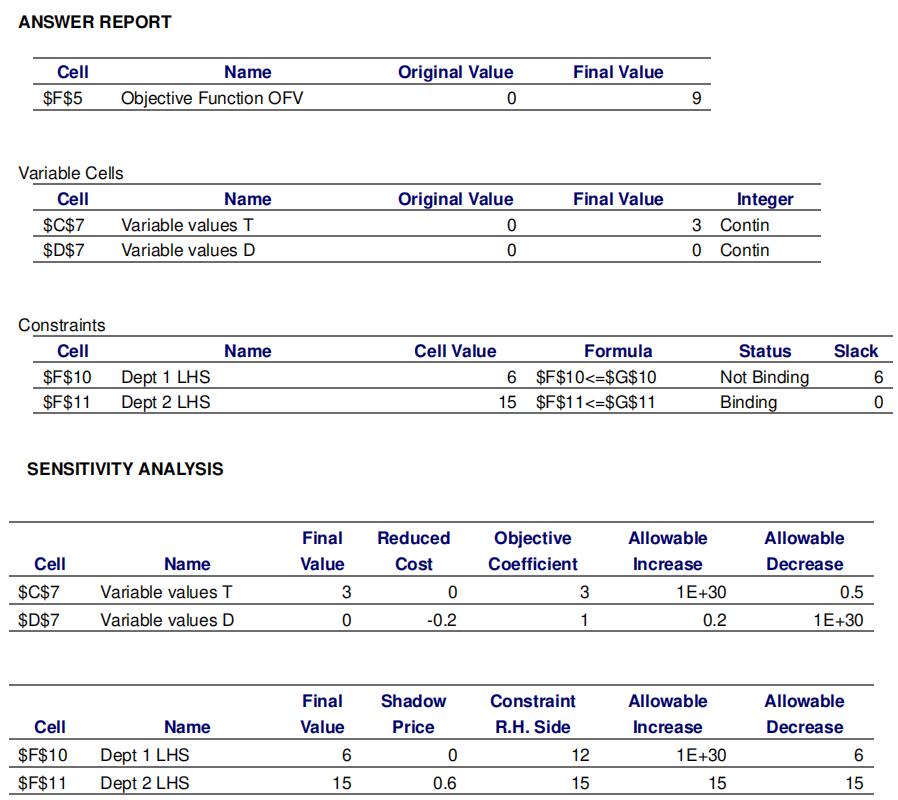
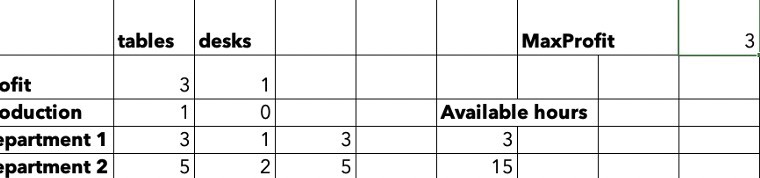
8.(10points)
Wood Products Inc. makes two products, Tables and Desks. They produce these products using a two-stage process designated as Department I and Department II. In Dept I, the raw lumber is processed into pieces of wood that can be used for either product. In Dept II, the tables and desks are assembled. Therefore, every product must go through both departments. 代考运营管理
The products are sold at a profit of $3 for each table and $1 for each desk. In Department I it takes 2 hours to process wood for a table and 3 hours to process wood for a desk. In Department II they use 5 hours to make a table and 2 hours to make a desk. In the current production period, Dept I has 12 hours available and Dept II has 15 hours available. (you should be pretty familiar with this problem already)
Given: This problem, and optimal solution shown on the Excel Solver output, shown on page 6.
a) At optimality, how many hours of unused time exist in Department 1?
b) At optimality, if the coefficient of T in the objective function decreased from $3 to $2.75, what product(s) would be produced?
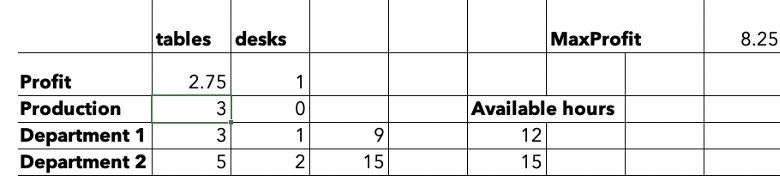
c) If the time available in Department I was reduced from 12 to 10, how much would you agree to pay to get the two hours back?

d) If we got another hour of time in Department II, would we be able to increase the number of tables that we can produce? Answer YES or NO, and explain briefly.

e) If we had to produce one desk (make D=1), would profit increase or decrease, and by how much?