Investments
Problem Sheet 3
代写Investment作业 If the stock market index goes down, high beta shares are also likely to go down, but not by as much as the market index.
True-False 代写Investment作业
- The best way of estimating the future beta of a share is to use the historic beta, computed by regressing the share return on the market return.
- If the stock market index goes down, high beta shares are also likely to go down, but not by as much as the market index.
- The evidence that high beta shares have a higher expected return than low beta shares, after controlling for other factors, is weak.
Questions
- Assets A and B are predicted to have the following returns in the five possible states of the world next period:
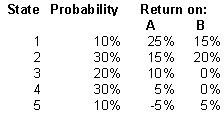
What is the expected return and standard deviation of returns on A and B? What is the correlation between the returns on A and the returns on B? Given that the only two assets you can invest in are A and B, is there any reason why anyone should want to buy some of B? 代写Investment作业
- Mr Entrepreneur’s entire wealth of £50m consists of shares in the company he founded. He is not happy holding such an undiversified portfolio, but is unwilling to sell any of his shares because he does not want to lose control of the company. A friend suggests that he could diversify by borrowing some money from the bank against the security of his shares and invest it in the stock market. In this way he would have a much more diversified portfolio. The bank is ready to lend him up to £25m. Can he reduce his total risk by doing as his friend suggests? Explain carefully.

Problems 代写Investment作业
- An investor can invest in two risky assets 1 and 2, and the risk-free asset. The expected returns on the risky assets are μ1= 0.15, μ2= 0.2, and their variance-covariance matrix is

(so each share has a volatility of √0.3, or about 55%, and their correlation is 2/3). The risk-free rate RF is 5%.
(a) What is the expected return and standard deviation of returns for a portfolio with:
(i) 50% invested in each of the two risky assets? 代写Investment作业
(ii) 25% invested in asset 1 and 75% invested in asset 2?
(b) Compute the Sharpe ratio for the four portfolios (100,0;25,75;50,50;0,100) where the Sharpe ratio is defined as
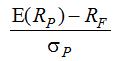
and sketch on a graph.
(c) Pretend that asset 2 is the market portfolio. Compute its correlation with the other portfolios, and their betas on it. Verify whether or not the Capital Asset Pricing Model holds if asset 2 is indeed the market portfolio.
2.The following annual excess rates of return were obtained for nine individual stocks and a market index. 代写Investment作业
| Year | Market Excess Returns(%) | A | B | C | D | E | F | G | H | I |
| 1 | 29.65 | 33.88 | -25.20 | 36.48 | 42.89 | -39.89 | 39.67 | 74.57 | 40.22 | 90.19 |
| 2 | -11.91 | -49.87 | 24.70 | -25.11 | -54.39 | 44.92 | -54.33 | -79.76 | -71.58 | -26.64 |
| 3 | 14.73 | 65.14 | -25.04 | 18.91 | -39.86 | -3.91 | -5.69 | 26.73 | 14.49 | 18.14 |
| 4 | 27.68 | 14.46 | -38.64 | -23.31 | -0.72 | -3.21 | 92.39 | -3.82 | 13.74 | 0.09 |
| 5 | 5.18 | 15.67 | 61.93 | 63.95 | -32.82 | 44.26 | -42.96 | 101.67 | 24.24 | 8.98 |
| 6 | 25.97 | -32.17 | 44.94 | -19.56 | 69.42 | 90.43 | 76.72 | 1.72 | 77.22 | 72.38 |
| 7 | 10.64 | -31.55 | -74.65 | 50.18 | 74.52 | 15.38 | 21.95 | -43.95 | -13.40 | 28.95 |
| 8 | 1.02 | -23.79 | 47.02 | -42.28 | 28.61 | -17.64 | 28.83 | 98.01 | 28.12 | 39.41 |
| 9 | 18.82 | -4.59 | 28.69 | -0.54 | 2.32 | 42.36 | 18.93 | -2.45 | 37.65 | 94.67 |
| 10 | 23.92 | -8.03 | 48.61 | 23.65 | 26.26 | -3.65 | 23.31 | 15.36 | 80.59 | 52.51 |
| 11 | -41.61 | 78.22 | -85.02 | -0.79 | -68.70 | -85.71 | -45.64 | 2.27 | -72.47 | -80.26 |
| 12 | -6.64 | 4.75 | 42.95 | -48.60 | 26.27 | 13.24 | -34.34 | -54.47 | -1.50 | -24.46 |
a.Perform the first-stage regressions (in Excel!) and tabulate the summary statistics
b.Specify the hypothesis for a test of the second-stage cross-sectional regression for the Security Market Line. 代写Investment作业
c.Perform the second-stage cross-sectional regression (Security Market Line regression) by regressing the average excess return of each portfolio on its beta.
d.Summarize your test results and compare them to the results reported in the text.
e.Group the nine stocks into three portfolios, maximizing the dispersion of the betas of the three resultant portfolios. Repeat the test and explain any changes in the results.

